filmov
tv
How To Solve Inverse Laplace transform Using Completing the Square
Показать описание
Solving Inverse Laplace Transform With Completing The Square.
In mathematics, the Laplace transform, named after its discoverer Pierre-Simon Laplace, is an integral transform that converts a function of a real variable (usually t, in the time domain) to a function of a complex variable s (in the complex frequency domain, also known as s-domain, or s-plane).
The transform has many applications in science and engineering because it is a tool for solving differential equations. In particular, it transforms ordinary differential equations into algebraic equations and convolution into multiplication. For suitable functions f, the Laplace transform is defined in this video.
In this video, we are going to solve the inverse Laplace Transform using partial fraction and completing the square.
#laplace_transformations
#laplacetransformation
#inverselaplacetransform
#inverselaplace
#completingthesquare
#avitechmaths
#avitechnologies
#engineeringmathematics
#advancedengineeringmathematics
In mathematics, the Laplace transform, named after its discoverer Pierre-Simon Laplace, is an integral transform that converts a function of a real variable (usually t, in the time domain) to a function of a complex variable s (in the complex frequency domain, also known as s-domain, or s-plane).
The transform has many applications in science and engineering because it is a tool for solving differential equations. In particular, it transforms ordinary differential equations into algebraic equations and convolution into multiplication. For suitable functions f, the Laplace transform is defined in this video.
In this video, we are going to solve the inverse Laplace Transform using partial fraction and completing the square.
#laplace_transformations
#laplacetransformation
#inverselaplacetransform
#inverselaplace
#completingthesquare
#avitechmaths
#avitechnologies
#engineeringmathematics
#advancedengineeringmathematics
0:05:36
The Inverse Laplace Transform - Example and Important Theorem
0:08:53
Inverse Laplace Transform Example using Partial Fractions
0:19:15
Inverse Laplace examples | Laplace transform | Differential Equations | Khan Academy
0:04:34
inverse laplace transform, example#5, with completing the square
0:10:12
Inverse Laplace
0:07:17
07 - Practice Calculating Inverse Laplace Transforms, Part 1
2:56:15
Ultimate Inverse Laplace Transform Study Guide
0:09:19
The Inverse Laplace Transform by Partial Fraction Expansion
0:00:59
Find Inverse Laplace Transform of [ e^(-3s) ×(s /(s^2+16)) ] _ shorts
0:07:27
Inverse Laplace Transform with unit step function, sect7.6#15
0:07:54
Inverse Laplace Transform, Sect 7.4#33
0:02:13
Inverse Laplace Transform, Sect 7.4#1
0:03:52
Inverse Laplace transform with unit step function, sect7.6#13
1:15:18
Inverse Laplace Transforms - Full Tutorial
0:03:40
Inverse Laplace Transform with completing the square of the denominator
0:03:11
Inverse Laplace Transform Example (With Completing the Square)
0:35:00
Inverse Laplace Transform
0:25:31
Convolution Method to Find Inverse Laplace Transforms | Practice Problems
0:30:59
How To Solve Inverse Laplace transform Using Completing the Square
0:06:26
How to Find the Inverse Laplace Transform of (s + 4)/(s^2 + 4s + 8)
0:09:25
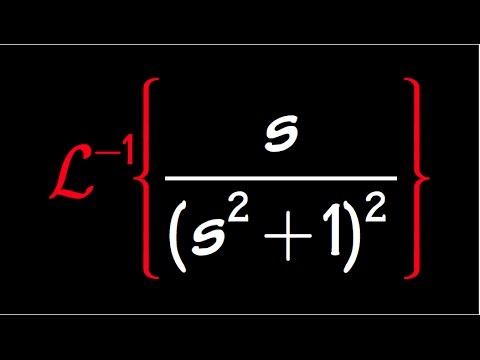
inverse laplace of s/(s^2+1)^2, using convolution theorem
0:08:45
Find Inverse Laplace Transforms: sinh(at) and e^(at)
0:09:17
08 - Practice Calculating Inverse Laplace Transforms, Part 2
0:05:35
How to find Inverse Laplace transform examples(PART-1) by easy maths easy tricks
Комментарии