30-60-90 triangle side ratios proof | Right triangles and trigonometry | Geometry | Khan Academy

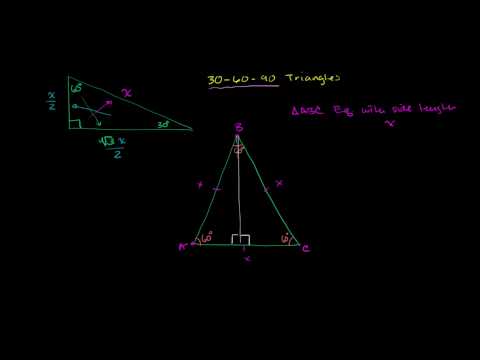
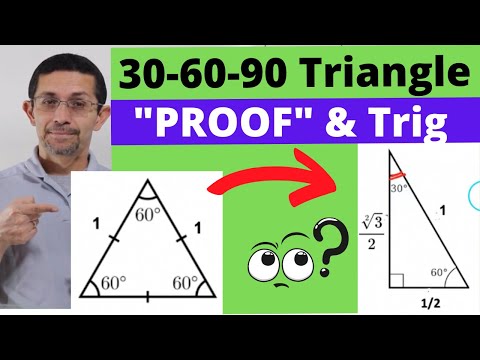
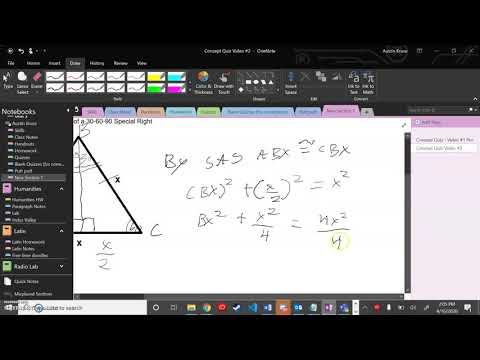
Proving the ratios between the sides of a 30-60-90 triangle

Missed the previous lesson?

Geometry on Khan Academy: We are surrounded by space. And that space contains lots of things. And these things have shapes. In geometry we are concerned with the nature of these shapes, how we define them, and what they teach us about the world at large--from math to architecture to biology to astronomy (and everything in between). Learning geometry is about more than just taking your medicine ("It's good for you!"), it's at the core of everything that exists--including you. Having said all that, some of the specific topics we'll cover include angles, intersecting lines, right triangles, perimeter, area, volume, circles, triangles, quadrilaterals, analytic geometry, and geometric constructions. Wow. That's a lot. To summarize: it's difficult to imagine any area of math that is more widely used than geometry.

About Khan Academy: Khan Academy offers practice exercises, instructional videos, and a personalized learning dashboard that empower learners to study at their own pace in and outside of the classroom. We tackle math, science, computer programming, history, art history, economics, and more. Our math missions guide learners from kindergarten to calculus using state-of-the-art, adaptive technology that identifies strengths and learning gaps. We've also partnered with institutions like NASA, The Museum of Modern Art, The California Academy of Sciences, and MIT to offer specialized content.

For free. For everyone. Forever. #YouCanLearnAnything

Subscribe to Khan Academy’s Geometry channel:

[sdhafele]

If you draw an isoscolese triangle with the 2 congruent sides labled as "a, " the triangle's height, h, will split the base, b, into 2 parts. If one of those parts is x, the other MUST be b-x. By the Pythagorean Theorem, x^2+h^2=a^2. By the Pythagorean Theorem, (b-x)^2+h^2=a^2. Using the Algebraic Identity (a-b)^2, you'll now know that b^2-2bx+x^2+h^2=a^2. Replacing x^2+h^2 w/ a^2 gives you b^2-2bx+a^2=a^2. Solving this equation for x tells you that x, one of the base's 2 parts, is b/2. The other part of the base, b-x, is b-(b/2), or, (2b/2)-(b/2), or simply b/2. All this proves that the height of an isoscolese triangle cuts the base of the triangle in half.

[MikeCasey]

Thank you, thank you. Every other video used these values without proof. 👍👍🇺🇸

[MoonLight]

therefore, the length of the sectors AB, BC, and AC are equal. It's concluded that AB line = BC line = AC line. BD line = CD line = 0.5*AB line = 0.5*AC line.
I hope it helps :)

[MoonLight]

This theorem can be proven by inscribing a 60-60-60 triangle (ABC) in a circle. Draw AB line such that it makes an angle of 30 deg with the horizontal diameter of a circle, drawn in a Cartesian coordinate system, of a center point (0, 0). Then, draw BC line such that it makes right angle with the horizontal diameter and then draw AC line. Since BC line is perpendicular to the horizontal diameter, therefore, BC line is bisected by the horizontal diameter in, let's say, D point.

[MoonLight]

If we look closely we will find out that two congruent right triangles are formed ADB and ADC. As a conclusion, BD line = DC line = 0.5*BD line. ABD angle = ACD angle = 60 deg. DAB angle = DAC angle = 30 deg. It's concluded that ABC is a 60-60-60 triangle. The inscribed angles, BAC, ABC, and BCA are equal to 60 deg and they are equal to half of their central angles each. Sector length = radius* angle(rad). Since the three angles of the inscribed 60-60-60 triangle are equal, therefore, Continued..

[trentonbouchard]

Perfect. I needed this video. I finally get it

[lukababilodze]

Thank you my math teacher doesn’t know how to teach us properly. (Of course she has the knowledge tho)

[natcat]

It is easier to think about to have the short leg as x, the long leg as x√3, and the hypotenuse as 2x.

[katrinaultriyama]

can you help me with this problem?

"The Pythagorean theorem is used to prove the side lengths of a right triangle. Use complete sentences to describe the proof of a right triangle with sides lengths of 7 meters, 24 meters, and 25 meters."

[ThomasGiles]

I think I get it now. Took a a lot of re-watching. Towards the end, you were moving a bit fast, I think.

[Ensign_Cthulhu]

@Veronicaboost You can never watch too much Khanacademy before a test. Provided of course that it's relevant to the test...

[skateraptor]

How is the leg bigger than the hypotenuse then when the hypotenuse is 10 radical 3? That means that 10 radical 3 times radical 3 all divided by 2 is 15?

[jonathan]

Where on earth did he get all those 4s, he just kept on adding them out of nowhere, someone please explain?

[ThomasGiles]

Why are we multiplying and voiding by 4

[ninderchitosiya]

Sr hindi me post krna plz
ya hindi medium ka koy channel btana plz

[LordOfNoobstown]

why can't you take the square root first in your final proof on x sqrt(3)/2

[dildobaggins]

"Khan academy ime gonna drop an altitude". Me: "Just say you drew a line"...

[bofa-zifj]

why is (BD)^2 not simplified to B^2 D^2?

[novanova]

just draw an right angled triangle then construct another right angled triangle then prove the whole triangle to be equiangular triangle then start the proof it is the easiest method

[babyhppoo]

What's that weird thing they have thats Like the square root of three and stuff