filmov
tv
Complex Analysis Part 1/2
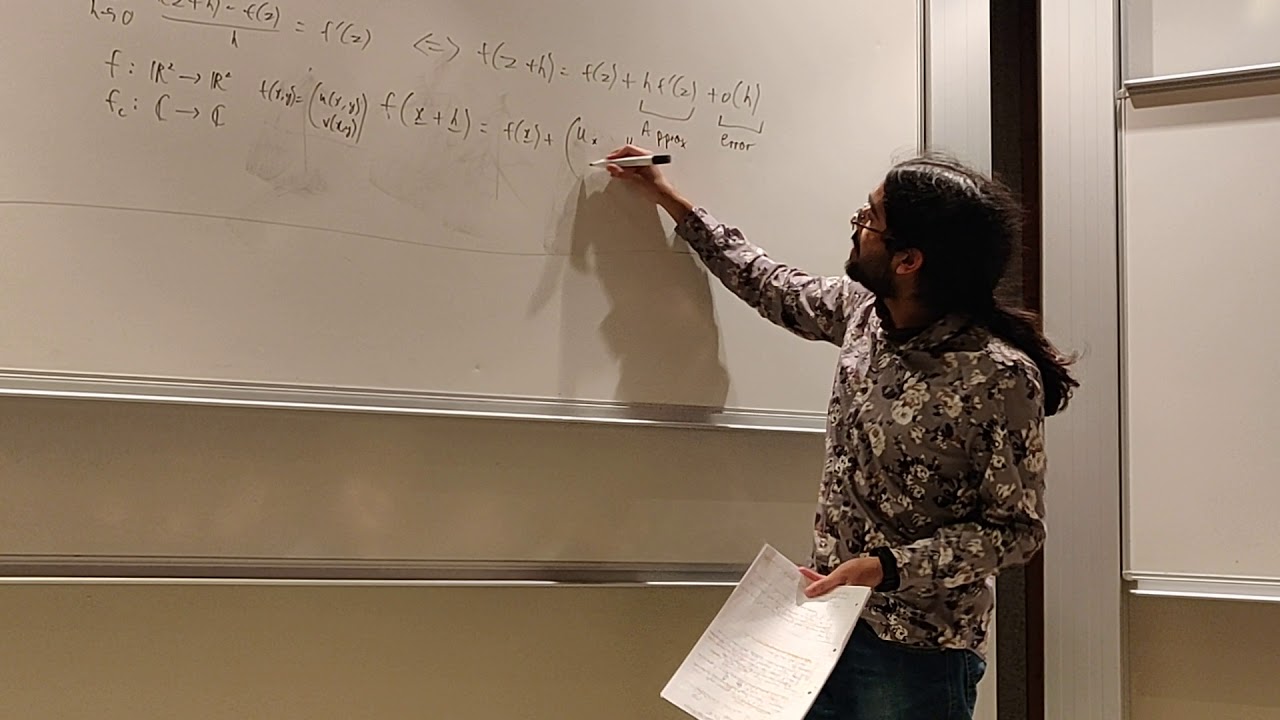
Показать описание
Neel Nanda gives a talk on some of the motivations, intuitions and underlying deeper maths for the 1B Complex Analysis course.
This talk was held in the Fisher Building, St. John's College
Time Stamps:
Chapter 1 (Intro & intuitions behind holomorphic functions, why they're so nice, and their basic properties, like angle preserving and Cauchy-Riemann) 0:00
Chapter 1*: Branch cuts! 17:00
Chapter 2 (Intuition behind what's really going on when we integrate) 29:00
Chapter 3 Intuitions behind the actual theorems and proofs:
55:30 Cauchy's for triangles & convex Cauchy
1:15:10 Cauchy's Integral Formula
1:23:15 Max Modulus, Liouville's & Fundamental Theorem of Algebra
1:35:25 Power series, Principle of Isolated Zeros
1:45:10 Infinite Differentiability, Morera's Thm, and how this should affect our intuitions from real analysis
0:09:47
Complex Analysis 1 | Introduction
0:07:01
Complex Analysis (Lesson 1)
0:21:16
History of Mathematics - Complex Analysis Part 1: complex numbers. Oxford Maths 3rd Yr Lecture
0:05:47
Imaginary Numbers Are Real [Part 1: Introduction]
0:12:34
Complex Analysis 02: Mappings
0:09:46
Complex Analysis 1 | Introduction [dark version]
0:32:18
The basics of complex numbers -- Complex Analysis 1
0:09:05
Complex Analysis | Unit 2 | Lecture 13 | Example of Cauchy's Integral Formula
0:25:40
Google Shares: Stockmarket Analysis With the Experts (The Market Edge, ep 4)
0:13:56
Laurent Series Explained | How to Determine Laurent Series | Complex Analysis #9
0:00:21
Complex analysis 101: imaginary numbers are real!
0:12:10
Complex Analysis | Analytic Function | Cauchy Riemann Equation BY GP sir
0:16:25
Type 1A (Analytic Function) - Problem 1 and 2 - Complex Variable - Engineering Mathematics 3
0:00:57
Introduction to Complex Variables - Math Book ASMR
0:54:30
Complex analysis by denni g zill solutions- lecture#4 Exercise#1.2 Questions 1 - 12 Complex analysis
0:00:32
Complex Analysis by Ahlfors #shorts
0:21:43
Complex Analysis Chapter 2 Analytic or Holomorphic Functions Example 1, 2, 3 and 4 related to C-R Eq
0:34:28
Exercise# 2.6 Q# 1to 8 Complex analysis by denni g zill - Limits of Complex Functions Part 1
0:07:16
Complex Analysis 11: Laurent Series
0:08:28
Complex Analysis 4 | Holomorphic and Entire Functions
0:00:39
Famous Graduate Level Complex Analysis Book
0:59:51
Complex Analysis: analytic at infinity, Laurent series, 10-29-24 part 1
0:22:11
But what is the Riemann zeta function? Visualizing analytic continuation
2:26:45
PYQs on Complex Analysis|June 2011- 2023| Part B and Part C| Short Cut Tricks
Комментарии