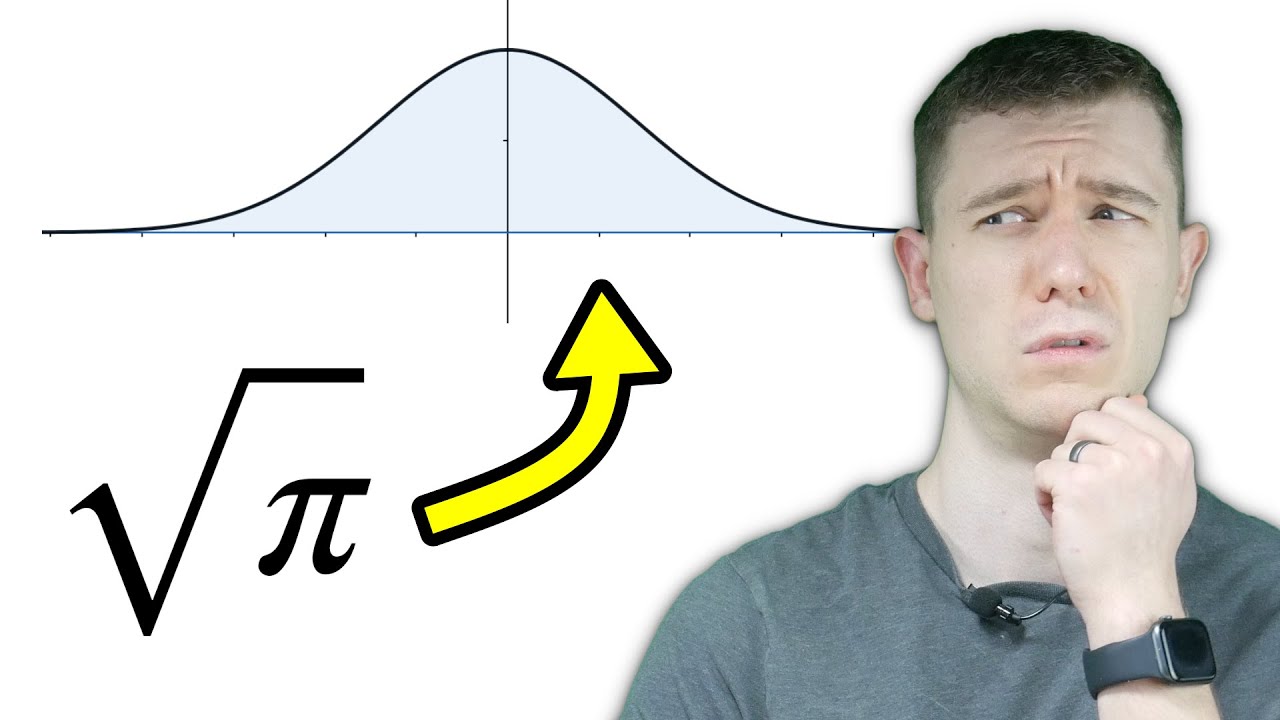
This isn't a Circle - Why is Pi here?

This famous bell shaped curve has a pretty famous result. It's not exactly clear why the circle constant pi is showing up in this normal distribution curve. Let's investigate this Gauss Curve (Gaussian Distribution) and get to the bottom of why Pi is here!

#math #brithemathguy#integral

►BECOME A CHANNEL MEMBER

Disclaimer: This video is for entertainment purposes only and should not be considered academic. Though all information is provided in good faith, no warranty of any kind, expressed or implied, is made with regards to the accuracy, validity, reliability, consistency, adequacy, or completeness of this information.

[BriTheMathGuy]

🎓Become a Math Master With My Intro To Proofs Course! (FREE ON YOUTUBE)

[Maibes]

I honestly believed this guy was writing backwards until I realized you can just flip the video :p

[mykolahubchak]

Damn that was clean. What a smooth transition to pi

[thoranevans]

I'm literally taking an upper-level mathematical statistics course right now and immediately saw that equation and thought isn't that the pdf of a standard normal distribution???"

[fotnite_]

Figuring this out on our own was a homework problem in the calculus course I took last semester

[henrylee]

I think there are some confusing elements here. The y domain does not "become" the theta domain, it's more of a double substitution (x, y)->(r, theta) and maybe explain more why it is that dxdy=r*dr*d(theta) from the jacobian etc.

[tumak]

Great presentation! We see, again, why Pi is one of "holiest" numbers in mathematics. Cheers

[GlorifiedTruth]

"Did you expect a pie to fall out of here?" THAT'S WHAT SHE SAID.

[JayTemple]

Funny story: When I was in college, I took a course designed for people who wanted to be math professors. One of the presenters showed us (old tech alert) a calculator that you could put on the glass surface of an overhead projector. It would even integrate functions, something I hadn't seen done on any calculator at the time. For fun, I asked the presenter to have it integrate e^(-x^2). She didn't even understand why I was asking for that particular function until the professor explained that it didn't have a closed-form antiderivative. She dutifully typed it in and it responded (whatever the constant is) times erf(x). I protested, "That's cheating!"

[tylershepard]

I often deal with Gaussian curves in many dimensions as an electrical engineer with signal processing. The really cool part is that (given that the covariance matrix is diagonal), the pdf is constant over an N-D sphere! I think that is a beautiful way to connect the two concepts.

[merkurin]

I haven't even learned about integrals in school. Why am I watching this?
Sounds rather interesting, though.. I'll come back when I know about it.

[hhill]

As soon as you started talking about using y in the second integral I saw it.... that is so cool

[turtle]

Slowly learning claculus and understanding these types of videos better and better is the most satisfying thing ever

[kanvolu]

Im taking integral calculus in college rn and our teacher told us this integral would be the last one we'd integrate at the course, but yesterday she made us demonstrate it comverges. It always impresses me how creative you gotta get to solve some math problems.

[TruthOfZ]

This is so beautifully done ✅ I love math even more now !!!

[crimfan]

A few comments from a statistician:

Nice walkthrough of the polar coordinates trick. I will link this video for my class!

The Gaussian distribution isn't too common for actual empirical things. For example, raw scores on IQ tests aren't really Gaussian; the tests are transformed to be Gaussian via the probability integral transformation. Heights too aren't Gaussian. Even in a restricted population such as adult females, heights tend to be somewhat skewed. What is usually Gaussian? Sampling distributions of well-behaved estimators. (In a sense, "well-behaved" actually means "has a Gaussian sample distribution in a reasonable sample size". In other words, the Central Limit Theorem holds.) It also works out tolerably well as an error distribution in regression, but that comes after systematic effects have been removed, and a lot of modern statistics involves using the Gaussian as a building block to make better distributions that fit more empirical phenomena.

An even deeper reason the pi shows up is because of Stirling's approximation. The original derivation of the Gaussian was by Abraham de Moivre, who used it to approximate probabilities of the binomial and sought something that would be easier to calculate than the intractable combinatoric terms. That would make a great video, too.

In think in practice the Gaussian integral (aka erf) is actually approximated by a series, although as I recall it's not a Taylor series, but a Hermite polynomials---don't quote me on that. I suspect that in Ye Olde Days they used a power series to do it.

[parkerp.]

I am really really trying to understand this, but I have not taken any form of a Calculus class yet (not even precal) and it is very difficult.

But I will continue watching these videos until I understand. We persevere.

[unknownuser]

Just in time. I have probability and statistics course this sem and I learned about normal distribution

[fatsquirrel]

I thought in stats we just let that area equal 1. The chance something will appear under that circle is 1.

[SciFurLycan]

Having recently finished calculus in 3D, I started having flashbacks when I saw that polar coordinates could be substituted in