Parametric Curves: Example 7: The Cycloid: Proof Part 1

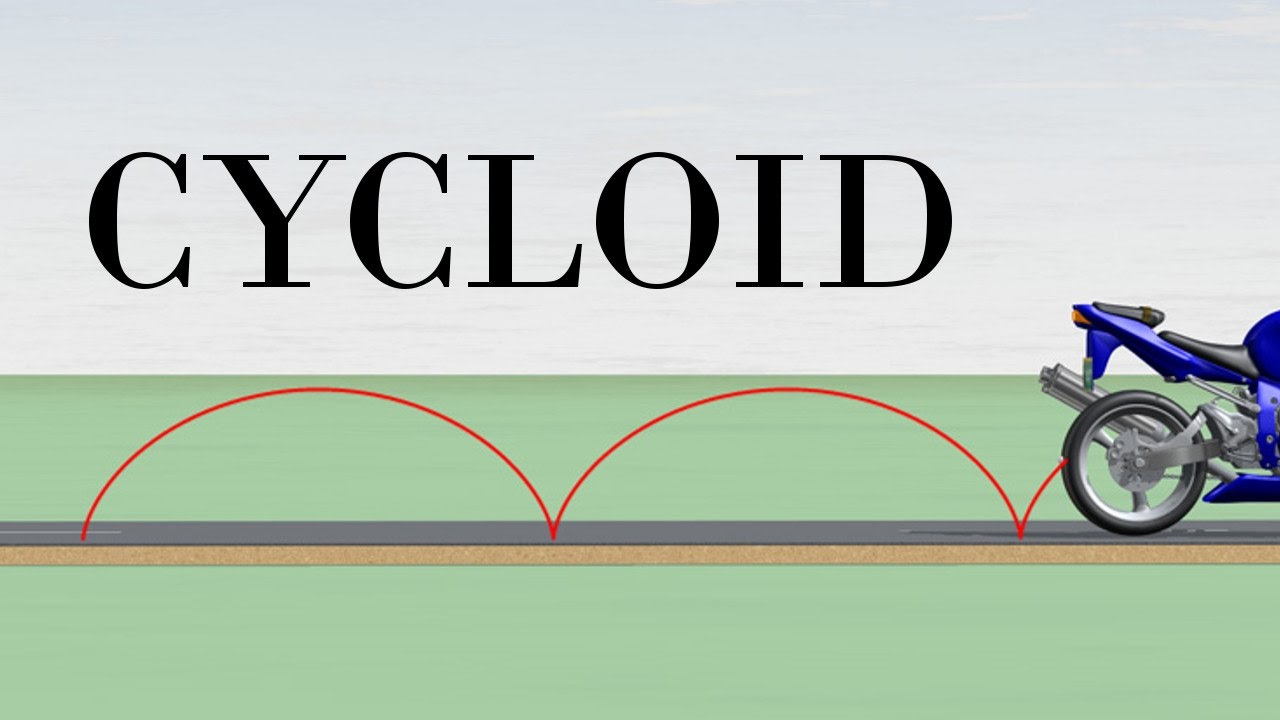
In this video I go over the cycloid curve and derive the parametric equations for the case in which the angle inside the circle is between 0 and π/2. The cycloid is formed by tracing a point on the circumference of a circle as it rotates along a straight line. The resulting parametric equations are shown below:

x = r(θ - sinθ)
y = r(1 - cosθ)

Although I derived these equations for the case where θ is between 0 and π/2, these equations do in fact work for all values of θ. I will go over the proof for other values of θ in a later video so stay tuned for that!

Also, these parametric equations can be rearranged to eliminate θ and to write x as a function of y, but the resulting Cartesian equation is much more complicated. I will nonetheless derive the Cartesian form of the cycloid in a later video, as well as the very interesting history of the cycloid, so stay tuned!

Related Videos:

------------------------------------------------------

[mes]

I don't always derive parametric equations but when I do they usually are for the famous Cycloid Curve ;)


#GetOnSteem

[scarlettgolds]

This is the best video I’ve found on this questions, thanks so much

[gazijarin]

Thank you! This was so easy to understand.

[ВікторТараба]

Thank you from Ukraine! Very easy and beautiful proof