filmov
tv
How to rationalize the radical to evaluate a limit

Показать описание
👉 Learn how to evaluate the limit of a function by rationalizing the radical. The limit of a function as the input variable of the function tends to a number/value is the number/value which the function approaches at that time.
The limit of a function is usually evaluated by direct substitution of the value which the variable tends to. When the function is a rational expression such that direct substitution leads to zero in the denominator, we find a way to either eliminate the denominator by multiplying both the numerator and the denominator by a common factor (this can involve rationalization) or decompose the denominator and the numerator into constituent parts so that like terms can cancel out.
Organized Videos:
✅The Limit
✅Evaluate Limits of Complex Fractions
✅Evaluate Limits of Polynomials
✅Evaluate Limits of Rational Expressions
✅Evaluate Limits with Square Roots
✅Evaluate Limits with Trig
✅Limits of Piecewise Functions
✅Evaluate Limits with Transcendentals
✅Evaluate Limits Difference Quotient
✅Evaluate Limits from a Graph
✅Evaluate Limits of Absolute Value
✅Evaluate Limits of Square Root
✅Holes and Asymptotes of Rational Functions
✅Learn about Limits
✅Find the Value that makes the Function Continuous
✅Is the Functions Continuous or Not?
✅Evaluate Limits using a Table of Values
✅Evaluate Limits at Infinity
Connect with me:
The limit of a function is usually evaluated by direct substitution of the value which the variable tends to. When the function is a rational expression such that direct substitution leads to zero in the denominator, we find a way to either eliminate the denominator by multiplying both the numerator and the denominator by a common factor (this can involve rationalization) or decompose the denominator and the numerator into constituent parts so that like terms can cancel out.
Organized Videos:
✅The Limit
✅Evaluate Limits of Complex Fractions
✅Evaluate Limits of Polynomials
✅Evaluate Limits of Rational Expressions
✅Evaluate Limits with Square Roots
✅Evaluate Limits with Trig
✅Limits of Piecewise Functions
✅Evaluate Limits with Transcendentals
✅Evaluate Limits Difference Quotient
✅Evaluate Limits from a Graph
✅Evaluate Limits of Absolute Value
✅Evaluate Limits of Square Root
✅Holes and Asymptotes of Rational Functions
✅Learn about Limits
✅Find the Value that makes the Function Continuous
✅Is the Functions Continuous or Not?
✅Evaluate Limits using a Table of Values
✅Evaluate Limits at Infinity
Connect with me:
Комментарии
 0:03:19
0:03:19
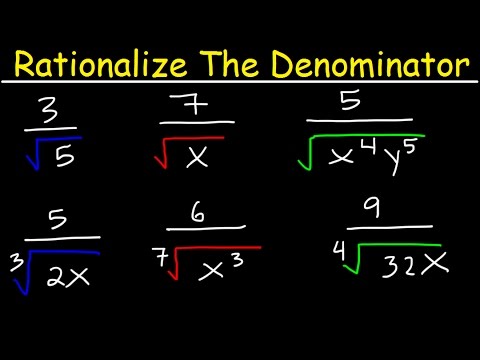 0:10:55
0:10:55
 0:10:18
0:10:18
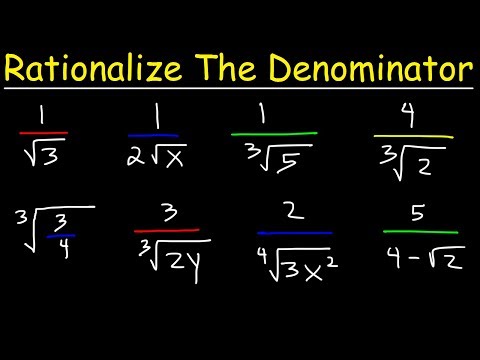 0:10:04
0:10:04
 0:02:33
0:02:33
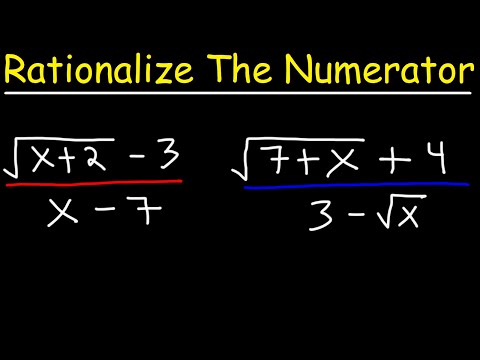 0:10:50
0:10:50
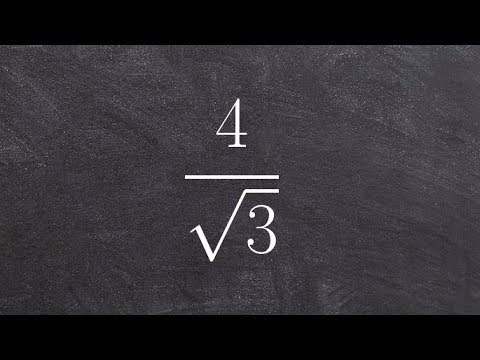 0:03:44
0:03:44
 0:02:22
0:02:22
 0:02:00
0:02:00
 0:14:54
0:14:54
 0:04:04
0:04:04
 0:03:06
0:03:06
 0:07:25
0:07:25
 0:02:47
0:02:47
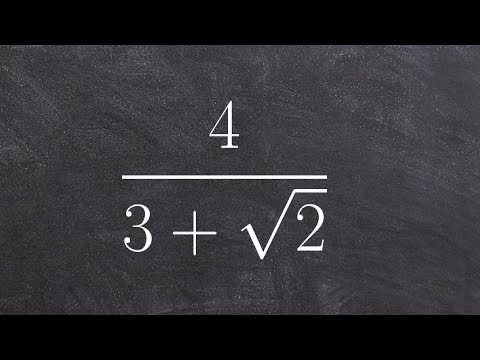 0:04:00
0:04:00
 0:13:14
0:13:14
 0:04:19
0:04:19
 0:02:45
0:02:45
 0:03:33
0:03:33
 0:01:34
0:01:34
 0:03:04
0:03:04
 0:01:28
0:01:28
 0:03:03
0:03:03
 0:04:01
0:04:01