filmov
tv
Math Olympiad || A Nice Irrational Equation || Find x
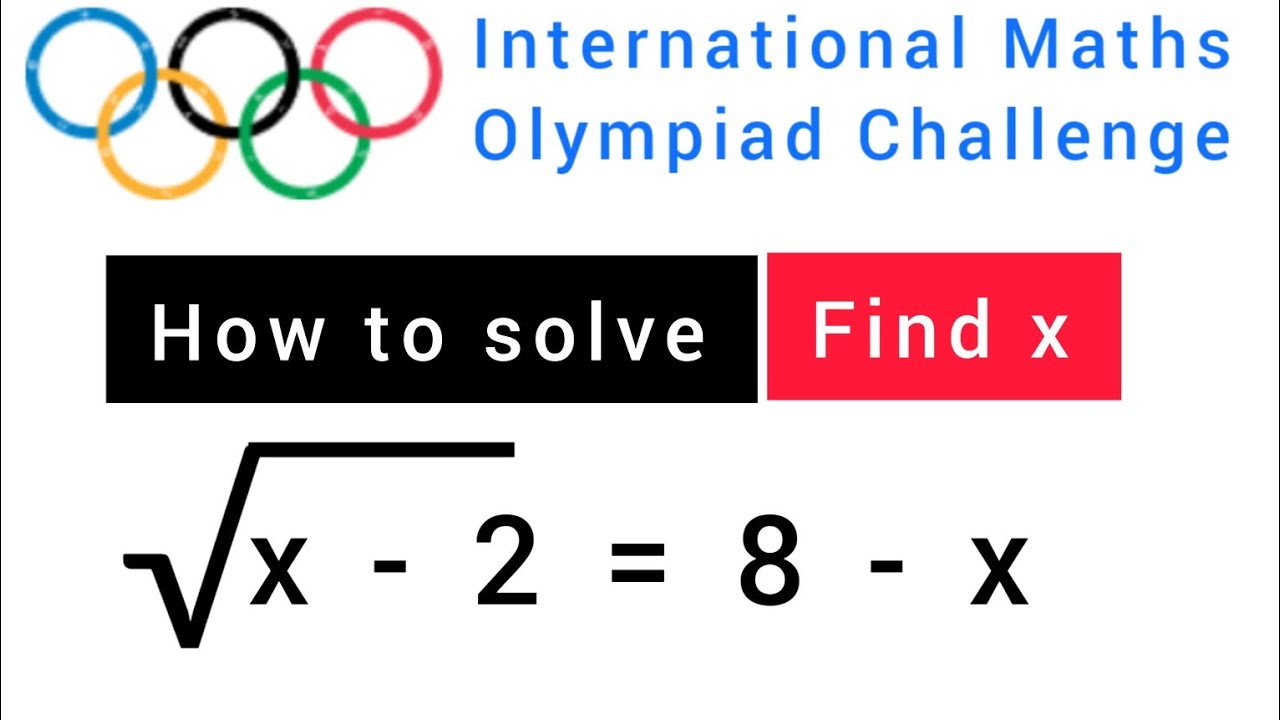
Показать описание
#matholympiad #irrationalequation #squareroot #olympiad
Hello Genius 👋
Hope you all are doing well 😊
Today we learn about irrational equation.
Irrational Equation are those where the variable is inside the radical that is the reason irrational equation is also known as Radical Equation.
plz subscribe to support me ❤️
Do you know what is reciprocal equation??
#matholympiadlreparation #matholympiadquestions#matholympiadtraining#MathOlympiad #solvingradicalequations#howtosolveradicalequations#solvingradicalequations withsquareroots#Radicalequations#algebraRadicalequationswithsquare roots#squarerootmethod#squareroottrick#Squareroot #expansionof(a+b)^2#mathematics#irrationalnumbersforclass9#irrationalnumber#irrationalnumbers
math olympiad preparation
math olympiad questions
math olympiad training
Math Olympiad
solving radical equations
how to solve radical equations
solving radical equations with square roots
Radical equations
Radical equations with square roots
square root method
square root trick
Square root
expansion of (a+b)^2
irrational numbers for class 9
irrational number
irrational numbers
Hello Genius 👋
Hope you all are doing well 😊
Today we learn about irrational equation.
Irrational Equation are those where the variable is inside the radical that is the reason irrational equation is also known as Radical Equation.
plz subscribe to support me ❤️
Do you know what is reciprocal equation??
#matholympiadlreparation #matholympiadquestions#matholympiadtraining#MathOlympiad #solvingradicalequations#howtosolveradicalequations#solvingradicalequations withsquareroots#Radicalequations#algebraRadicalequationswithsquare roots#squarerootmethod#squareroottrick#Squareroot #expansionof(a+b)^2#mathematics#irrationalnumbersforclass9#irrationalnumber#irrationalnumbers
math olympiad preparation
math olympiad questions
math olympiad training
Math Olympiad
solving radical equations
how to solve radical equations
solving radical equations with square roots
Radical equations
Radical equations with square roots
square root method
square root trick
Square root
expansion of (a+b)^2
irrational numbers for class 9
irrational number
irrational numbers
A Nice Math Olympiad Exponential Equation 3^x = X^9
Canada Math Olympiad | A Very Nice Geometry Problem
Thailand | Math Olympiad Question | Nice Algebra Equation
Mexico - A Nice Math Olympiad Exponential Problem
A beautiful international math olympiad problem
Japanese Math Olympiad Problem | A Nice Math Problem : Comparison
Nice Math Olympiad Simplification Problem | Fraction Exponents | You Should NOT use Calculators
Germany| A Nice math Olympiad exponential question.
How to Solve Nice Exponential Problem |Math Olympiad Preparation | Find value of X
A Nice Olympiad Exponential Multiplication Problem #short #olympiad #mathematics #maths #exponents
A nice math Olympiad problem |you should try to solve this#math #matholympiad
Math Olympiad | A Nice Algebra Problem 👇
Luxembourg - Math Olympiad Question | You should know this trick
Nice Olympiad Math | x^2–x^3=12 | Nice Math Olympiad Solution
Japanese| A nice math Olympiad question #maths #matheolympiad
China | Math Olympiad | A Nice Algebra Problem 👇👇👇
France Math Olympiad | A Very Nice Geometry Problem
A Nice Math Olympiad Geometry Challenge | Circle inside a semicircle | Find the radius of the circle
A nice Math Olympiad Simplification Problem | Can you solve this ?
Math Olympiad | A Very Nice Algebra Problem
Norway Math Olympiad Question | You should be able to solve this!
Français | Math Olympiad | A Nice Algebra Problem
Français | Can you solve this ? | A nice Math Olympiad Exponential Simplification | Algebra Problem
A nice math Olympiad question #math #matholympiad
Комментарии
 0:02:34
0:02:34
 0:11:24
0:11:24
 0:01:26
0:01:26
 0:08:36
0:08:36
 0:01:00
0:01:00
 0:04:15
0:04:15
 0:13:59
0:13:59
 0:07:36
0:07:36
 0:03:27
0:03:27
 0:00:52
0:00:52
 0:16:39
0:16:39
 0:14:11
0:14:11
 0:02:51
0:02:51
 0:15:05
0:15:05
 0:09:55
0:09:55
 0:13:13
0:13:13
 0:12:37
0:12:37
 0:16:01
0:16:01
 0:09:54
0:09:54
 0:08:38
0:08:38
 0:03:21
0:03:21
 0:11:59
0:11:59
 0:10:49
0:10:49
 0:07:53
0:07:53