filmov
tv
What’s the sum? 1 - 1/2 + 1/4 - 1/8 + 1/16...

Показать описание
One way to find the sum is by using the formula for a geometric series, but there’s an even more interesting visual approach!
In this video, we use the shaded area of a hexagon to reveal the answer. If you know of any other approach, let us know in the comments!
---
Learn math the right way. Signup for a FREE trial session.
Link on the homepage.
#math #education #learning #tutor #tutoring #shorts #reels #area #hexagon #geometric #series #sum
In this video, we use the shaded area of a hexagon to reveal the answer. If you know of any other approach, let us know in the comments!
---
Learn math the right way. Signup for a FREE trial session.
Link on the homepage.
#math #education #learning #tutor #tutoring #shorts #reels #area #hexagon #geometric #series #sum
The Weeknd - Is There Someone Else? (Lyrics)
I was sleeping in the RV and suddenly I heard someone knocking on the door #rv #rvliving
Lewis Capaldi - Someone You Loved (Lyrics)
Gauss discovered this at a very young age (Sum 1 To 100 - Based On True Story)
He pushed someone until #shorts
The rabbit almost poured water on someone else#Short #Officer Rabbit #angel
Someone is copying you in Dress to Impress..
Is Someone Else Actually Making My Cakes While I Take Credit?
How to Tell if Someone is using their ADHD as an Excuse vs an Explanation #adhd #shorts
When someone refers to you as “chief” 😳👩🚒😂 #shorts
the most famous Ramanujan sum 1+2+3+...=-1/12
Nagin is lonely looking for someone to play with🥺, the police attack😱 #sakuraschoolsimulator #shorts...
When someone throws off your VIBE...
What Might 'Trigger' Someone with BPD, Borderline Personality Disorder
The Number One Sign Someone Is Constantly Thinking About You #psychictips
How do YOU respond when someone uses “what’s up” as an on-the-go greeting? #millennials #relatable...
Someone help her in the comments #wusworld
POV: someone saw your character ai chats #characterai
Tag someone who can relate 🤣💄
I can’t believe someone gave Reddington a nickname.#shorts #shortvideo #viralvideo #foryou
Music taste is important,imagine dating someone who doesn’t know what comes after this intro #metal...
Someone please save Zenitsu😭 #demonslayer #fyyy #anime #art #inosuke #tanjiro #zenitsu
An Obituary For Someone Who Definitely Faked Their Own Death
The Lowest GPA Someone Got Into Harvard With
Комментарии
 0:03:20
0:03:20
 0:00:41
0:00:41
 0:03:03
0:03:03
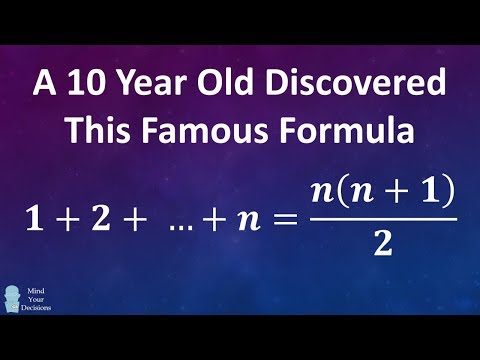 0:05:24
0:05:24
 0:00:19
0:00:19
 0:00:25
0:00:25
 0:00:17
0:00:17
 0:00:34
0:00:34
 0:00:32
0:00:32
 0:01:00
0:01:00
 0:11:40
0:11:40
 0:00:33
0:00:33
 0:00:21
0:00:21
 0:01:01
0:01:01
 0:00:53
0:00:53
 0:00:14
0:00:14
 0:01:00
0:01:00
 0:00:13
0:00:13
 0:00:10
0:00:10
 0:01:00
0:01:00
 0:00:12
0:00:12
 0:01:01
0:01:01
 0:01:00
0:01:00
 0:00:27
0:00:27