Louis Kauffman, Reconnection Number of Vortex Knots

This is a talk on the conference 'Knot theory and applications',
June 29 - July 4, 2022

Abstract:
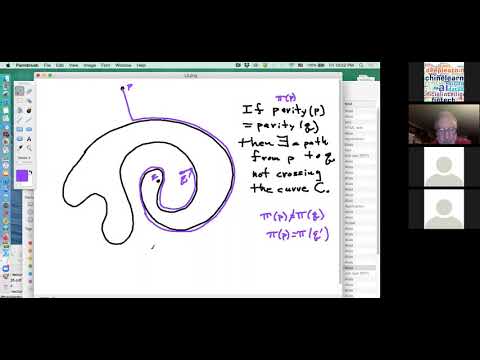
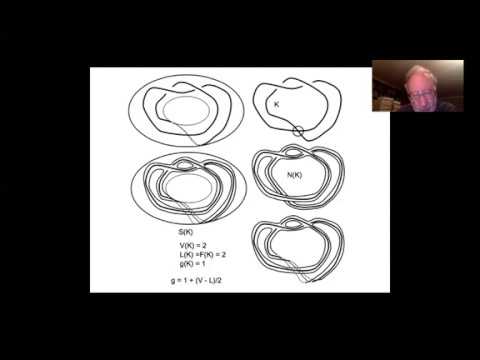
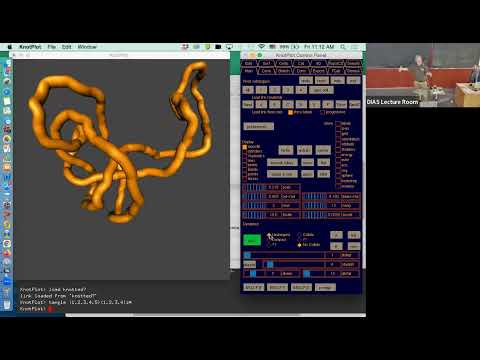
Knotted vortices such as those produced by Kleckner and Irvine at the Frank Institute of the Univ of Chicago tend to transform by reconnection to collections of unknotted and unlinked circles. The reconnection number R(K) of an oriented knot of link K is the least number of reconnections (oriented re-smoothings) needed to unknot/unlink K. Putting this problem into the context of knot cobordism, we show, using Rasmussen’s Theorem that the reconnection number of a positive knot is equal to twice the genus of its Seifert spanning surface. In particular an (a,b) torus knot has R = (a-1)(b-1). For an arbitrary positive knot or link K, R(K) = c(K) - s(K) + 1 where c(K) is the number of crossings of K and s(K) is the number of Seifert circles of K. Examples of vortex dynamics will be illustrated.