sec^3 x tan x dx, Evaluate the indefinite integral.

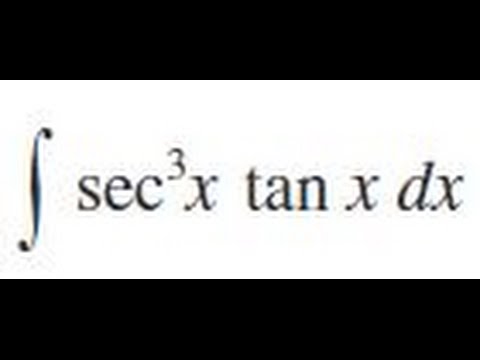
preview_player
Показать описание
sec^3 x tan x dx, Evaluate the indefinite integral.
  1. MSolved Tutoring
Рекомендации по теме
Evaluate the Integral 0:02:40

Evaluate the Integral sec^3 x tan x dx with substitution rule

sec^3 x tan 0:00:51

sec^3 x tan x dx, Evaluate the indefinite integral.

Integral of tan^3(x)*sec(x), 0:02:40

Integral of tan^3(x)*sec(x), calculus 2 tutorial

Integral of tan^3x, 0:02:46

Integral of tan^3x, with tan and sec

Integral of sec^3x 0:07:01

Integral of sec^3x

Evaluate the Integral 0:02:05

Evaluate the Integral tan x sec^3 x dx U-Substitution.

Integral of tan(x) 0:02:54

Integral of tan(x)

integral tan(3x) sec(3x) 0:01:14

integral tan(3x) sec(3x) dx , secante por tangente

Class 12th – 0:02:39

Class 12th – Integral of tan x dx | Integrals | Tutorials Point

trig integrals involving 0:16:51

trig integrals involving secant and tangent (calculus 2)

Class 12th – 0:03:29

Class 12th – Integral of Tanx Tan2x Tan3x dx | Integrals | Tutorials Point

Evaluate the Integral 0:04:25

Evaluate the Integral tan^5 x sec^3 x dx U-Substitution. Example 24. Trigonometric

Evaluate the Integral 0:02:21

Evaluate the Integral tan^3 x sec x dx U-Substitution. Example 25. Trigonometric

integral of sec 0:02:48

integral of sec x tan x dx

Integral of tan^3x*sec^4x 0:04:17

Integral of tan^3x*sec^4x (read pinned comment)

Integral of sec(x) 0:12:41

Integral of sec(x) but without that trick!

Integral of sec(x) 0:02:16

Integral of sec(x)

Integral of tanx 0:05:28

Integral of tanx

Integral of sec^2(x)tan^3(x) 0:00:25

Integral of sec^2(x)tan^3(x) dx

integral of x*tan^ 0:05:04

integral of x*tan^ -1(x), calculus 2 tutorial, integration by parts

7-30 Evaluate the 0:00:33

7-30 Evaluate the indefinite integral. ∫^3 x tanx d x

my favorite calculus 0:07:05

my favorite calculus 2 trigonometry integral (two results off by a constant)

Class 12th – 0:02:46

Class 12th – Integral of sec x dx | Integrals | Tutorials Point

when calculus students 0:00:43

when calculus students use trig identities too early

Комментарии
Автор

FOR THOSE WONDERING ABOUT MISSING STEPS


What he did was take one sec(x) factor out so that he was left with sec^2(x)*sec(x)*tan(x). You can see that, with u = sec(x) and du = sec(x)tan(x), the sec(x)tan(x) cancel out so that we are left with sec^2(x) or u^2.

jhansenhlebica
Автор

Just a side tip, people that come on here have no clue what they are doing, and they would love clarification. I just came to check my answer, but hey. But really we need help haha.

mrvomitman
Автор

This worked for me. My school uses webassign...very unforgiving...thx!

PrincessPea
Автор

Great video. This just still doesn't make any sense. How are we able to pick and choose which sec(x) ends up as part of our "u"?

JDMaxton
Автор

El resultado de la integral que se resuelve en el video es incorrecto sumandole que tiene mál procedimiento; el resultado correcto es: 1/3sec^3(x)

yesshc
Автор

how come it's u^2 and not u^3 after you sub u back in?

katiiiielynn
Автор

This has got to be the MOST unhelpful thing i've ever seen

husse