filmov
tv
Convolution theorem Method|| lnverse Laplace Transform of imp. problems|| BS Calculus ||ShahHussain

Показать описание
Learn Mathematics By Shah Hussain
Dear viewer well come to my channel
☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆
Learn Mathematics By Shah Hussain
CONVOLUTION:
Convolution is a mathematical tool which is used to calculate the output.
convolution is also an inverse laplace transform tool. basically convolution is a mathematical operator
▪A convolution is an integral that express the amount of overlap of one function when it is shifted over anothet function.
APPLICATION OF CONVOLUTION:
It is applied in Electrical engineering , circuit and also applied in signal processing
PROPERTIES OF CONVOLUTION:
▪Commutative law hold
f*g=g*f
▪Distributive law hold
f*(g+h)=f*g+f*h
▪Assosiative law hold
f*(g*h)=(f*g)*h
▪f*0=0
▪f*1 is not=1 in general
▪1*1 is not=1
▪1*1=t
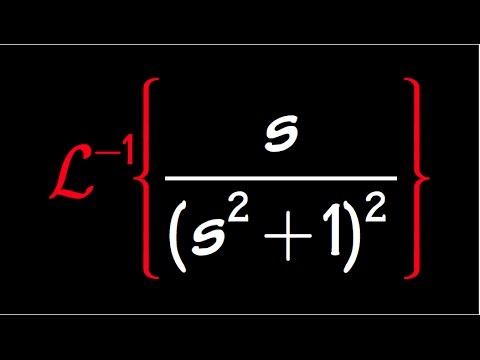
CONVOLUTION THEOREM
or
FALTUNG THEOREM
L{f(t)*g(t)}=L{f(t)}L{g(t)}
or
L{f(t)*g(t)}=F(s)G(s)
where " * " is convolution operator
Note:
L{f(t)g(t)} is not=L{f(t)}L{g(t)}
or
L{f(t)g(t)} is not=F(s)G(s)
.........................................................................
"The Laplace Transform"
▪The laplace transform is an efficient technique for solving linear differential equations with constant coefficients .We shall study it's basic properties and will apply them to solve initial value problems.
As the name suggests,
▪ Laplace Transform is an operator which transforms a function f of the variable t into a function F of the variable s.
"Let f be a real- valued piecewise continues function defined on [0,infinity). The laplace transform of f , denoted by L(f), is the function F defined by F(s)
Provided the improper integral in 1 converges.
▪The domain of F is the set of all real numbers s for which the above integral converges."
▪The transform operation transforms the given function f of the variable t into a new function F of the variable s
..........................................
▪The laplace transform exists only for certain class of functions.
▪Hope so you have understand it.
For more information and lectures visit to my channel maths online by Shah Hussain.
8Also give your feedback and valuable suggestions in comment box.
☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆
☆Links:
☆Whatsapp: +923006967727
Note:
If you realise that this YouTube video helped you in mathematics, you can donate money to a video creator .This gesture shows motivation for video creator and an appreciation. The amount does not have to be big but it truly show the encouragement ,appreciation and support.
SHAH HUSSAIN
Meezan Bank-Depalpur, Sahiwal Area
Account Number: 98130103854230
.............................................................................
Thank you so much😊😊❤❤
It gives me immense pleasure for your valuable suggestions and comments.
..............................................................................
#SHAH_HUSSAIN#CONVOLUTION_THEOREM
#CONVOLUTION_THEOREM#BScMaths
#CONVOLUTION_PROPERTIES
#FALTUNG_THEOREM
#SCALING_PROPERTY
#LAPLACE_TRANSFORM
#MATHS_ONLINE#BS_MATHS
#INVERSE_TRANSFORM
#DERIVATIVE_TRANSFORM
#TRANSFORM_DERIVATIVE
#CHANGE_SCALE#PROPERTY
#LAPLACE_PROPERT
Dear viewer well come to my channel
☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆
Learn Mathematics By Shah Hussain
CONVOLUTION:
Convolution is a mathematical tool which is used to calculate the output.
convolution is also an inverse laplace transform tool. basically convolution is a mathematical operator
▪A convolution is an integral that express the amount of overlap of one function when it is shifted over anothet function.
APPLICATION OF CONVOLUTION:
It is applied in Electrical engineering , circuit and also applied in signal processing
PROPERTIES OF CONVOLUTION:
▪Commutative law hold
f*g=g*f
▪Distributive law hold
f*(g+h)=f*g+f*h
▪Assosiative law hold
f*(g*h)=(f*g)*h
▪f*0=0
▪f*1 is not=1 in general
▪1*1 is not=1
▪1*1=t
CONVOLUTION THEOREM
or
FALTUNG THEOREM
L{f(t)*g(t)}=L{f(t)}L{g(t)}
or
L{f(t)*g(t)}=F(s)G(s)
where " * " is convolution operator
Note:
L{f(t)g(t)} is not=L{f(t)}L{g(t)}
or
L{f(t)g(t)} is not=F(s)G(s)
.........................................................................
"The Laplace Transform"
▪The laplace transform is an efficient technique for solving linear differential equations with constant coefficients .We shall study it's basic properties and will apply them to solve initial value problems.
As the name suggests,
▪ Laplace Transform is an operator which transforms a function f of the variable t into a function F of the variable s.
"Let f be a real- valued piecewise continues function defined on [0,infinity). The laplace transform of f , denoted by L(f), is the function F defined by F(s)
Provided the improper integral in 1 converges.
▪The domain of F is the set of all real numbers s for which the above integral converges."
▪The transform operation transforms the given function f of the variable t into a new function F of the variable s
..........................................
▪The laplace transform exists only for certain class of functions.
▪Hope so you have understand it.
For more information and lectures visit to my channel maths online by Shah Hussain.
8Also give your feedback and valuable suggestions in comment box.
☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆☆
☆Links:
☆Whatsapp: +923006967727
Note:
If you realise that this YouTube video helped you in mathematics, you can donate money to a video creator .This gesture shows motivation for video creator and an appreciation. The amount does not have to be big but it truly show the encouragement ,appreciation and support.
SHAH HUSSAIN
Meezan Bank-Depalpur, Sahiwal Area
Account Number: 98130103854230
.............................................................................
Thank you so much😊😊❤❤
It gives me immense pleasure for your valuable suggestions and comments.
..............................................................................
#SHAH_HUSSAIN#CONVOLUTION_THEOREM
#CONVOLUTION_THEOREM#BScMaths
#CONVOLUTION_PROPERTIES
#FALTUNG_THEOREM
#SCALING_PROPERTY
#LAPLACE_TRANSFORM
#MATHS_ONLINE#BS_MATHS
#INVERSE_TRANSFORM
#DERIVATIVE_TRANSFORM
#TRANSFORM_DERIVATIVE
#CHANGE_SCALE#PROPERTY
#LAPLACE_PROPERT
Комментарии
 0:09:25
0:09:25
 0:13:35
0:13:35
 0:04:56
0:04:56
 0:13:46
0:13:46
 0:10:33
0:10:33
 0:06:24
0:06:24
 0:00:06
0:00:06
 0:00:10
0:00:10
 0:50:11
0:50:11
 0:16:55
0:16:55
 0:00:05
0:00:05
 0:10:54
0:10:54
 0:11:17
0:11:17
 0:25:31
0:25:31
 0:07:03
0:07:03
 0:33:57
0:33:57
 0:42:15
0:42:15
 0:09:04
0:09:04
 0:48:26
0:48:26
 0:03:36
0:03:36
 0:03:57
0:03:57
 0:00:13
0:00:13
 0:05:12
0:05:12
 0:18:31
0:18:31