The Weierstrass Definition of the GAMMA FUNCTION! - Proving Equivalence!

Help me create more free content! =)

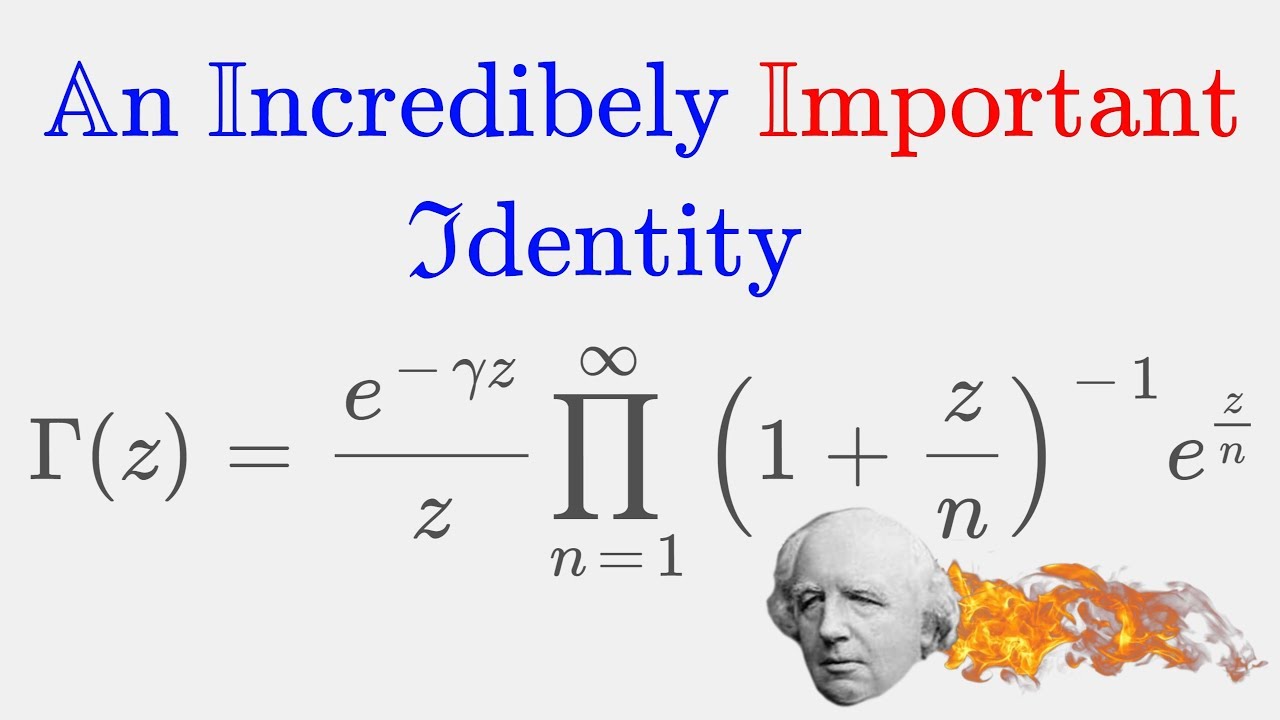
Today we are going to backwards engineer the WEIERSTRASS Definition of the gamma function to our already derived Euler-Gauss Definition! Infinite Product fun! =D Enjoy :)

[vangrails]

The gamma function is the gift that keeps on giving.

[everlastingauraX]

The gender of this operator is left as an exercise to the reader.

[neilgerace]

9:20 yeah I got confused last time you did this, but it makes sense to me now because you are taking the limit

[mihaipuiu]

Flammable...you are a very good teacher. Your explanation is perfect, very clean, and nice.Thanks

[neilgerace]

0:55 Ah, good. I've been waiting to learn about Weierstrass from someone who can pronounce it

[duncanw]

I'd say that the pi is an infinity grill. Because it looks like a literal grill.

[kalvin]

Oh my God! BRAVO 🍄 and I absolutely cannot control myself for the long-awaited digamma and polygamma functions!!!! 🍄🍄🍄

[TheRandomFool]

Infinite sum is infinity boi, infinite product is infinity grill.

[J.P.Nery.N.]

My mother language is portuguese and here the product is called "o produto", with "o" indicating it's male. So it's an infinity hell yeah! Lol

[duncanw]

Them: what u watch
me: polygamma function

[ylenolsreppeptgs]

i cant wait for some of the sexy definite integrals papa is about to solve after he derives the taylor expansion for ln(gamma(1 + x))

[ralphhebgen]

Ok I am going to say it. Sometimes you are supremely funny. “This is some stuff you can use to get rid of your spare time” 😂😂😂. “We can do the limit Hadoken”. That one must be the coolest way to refer to limit operations EVER. 😂😂😅

[wraithreaper]

If you define your product in order to express n as being of the form k/k-1 then your index k has to be equals to or greater than 2, running up until n because setting k=1, as you proposed, means dividing by zero. Also, if terms on the product will be of the form (k+1)/k. index may run from 1 to n-1. Please correct me if I'm wrong, Papa Flammy.

[leafbaguette]

this formula is so nice, it makes the poles at the negative integers explicit

[Azelide]

Product notation = letter Pi = Cutie Pie = female 🤣

[borisivanov]

Product is big and boxy, so it’s a boi. Sum has a somewhat hourglass shape, so it’s the sum that must be a grill.

[silentinferno]

Where can I find that book in the intro??? I'm in desperate need of

[mrandersonpw]

2 min 35 seg of introduction? Man, you are getting harder in every next video. So Savage Papa.

[MathematicsOptimization]

But what *is* a bröh-moment?!



































-> when the autofocus is still not disabled

[tanvec]

I'm going to use mathhematical hadouken to wake my students up