What are Equally Likely Events | Equally Likely Event Vs Not Equally Likely Events

Equally Likely Event Vs Not Equally Likely Events.

Events having equal no of chances are called Equally likely Events.

Events having unequal no of chances are called Not Equally likely Events.

#equallylikelyevents
#notequallylikelyevents
#equalevents
#notequalevents

In probability theory, events can be categorized into equally likely events and unequally likely (or not equally likely) events based on the likelihood of each outcome occurring.

1. Equally Likely Events:

Equally likely events are events that have the same probability of occurring. In other words, each outcome in the sample space has the same chance of happening.

Example:

Tossing a fair coin: The outcomes (heads and tails) are equally likely because the probability of getting heads is 50%, and the probability of getting tails is also 50%.

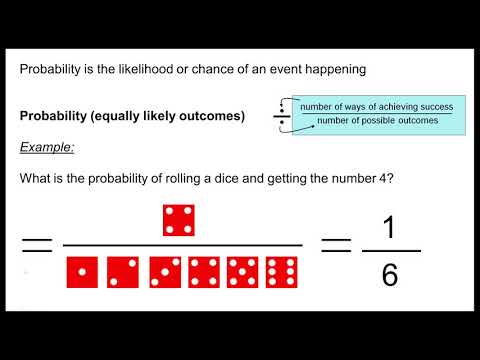
Rolling a fair six-sided die: Each number (1, 2, 3, 4, 5, or 6) has the same probability of occurring, which is 1/6.

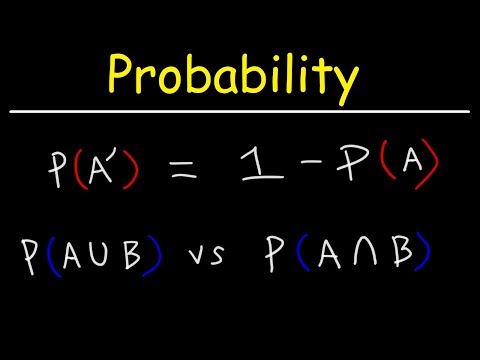
Mathematically, for equally likely events:

The probability of each event is the same

2. Unequally Likely Events:

Unequally likely events are events where the outcomes have different probabilities of occurring. Some outcomes are more likely than others.

Example:

Rolling a biased die: If a die is weighted so that it is more likely to land on a certain number (say, 6), then the events are not equally likely. The probability of rolling a 6 would be higher than the probability of rolling any other number.

Drawing a card from a deck without replacement: If you remove a card from the deck, the remaining cards are not equally likely to be drawn because there is one less card, and the probabilities change.

Mathematically, for unequally likely events:

The probability of each event is different, and it depends on the conditions or biases affecting the outcome

Equally likely events: All outcomes have the same probability (e.g., fair coin toss, fair die roll).

Unequally likely events: Some outcomes have a higher probability than others (e.g., biased die, certain conditions in card drawing).

Probability

#probability #prioriprobability #axiomaticprobability #randomexperiment #deterministicexperiment #setandtypesofset #mutiluallyexclusiveevents #independentevents
#equallylikelyevents #exhaustiveevents #samplespace #eventspace #posterioriprobability #lawsofprobability #bayestheorem #applicationofbayestheorem
#applicationoflawsofprobability #questionsbasedonprobability # #conditionalsamplespace #reducedsamplespace #permutation #combination #rulesofmultiplication
#treediagram #venndiagram #pictorialdiagram
#rulesonevents #operationsonevents #complementryevents #lawofcomplement
#additionlawofmutuallyexclusive
##additionlawofnotmutuallyexclusive
#compoundevent #singletonevent

Links Play List