filmov
tv
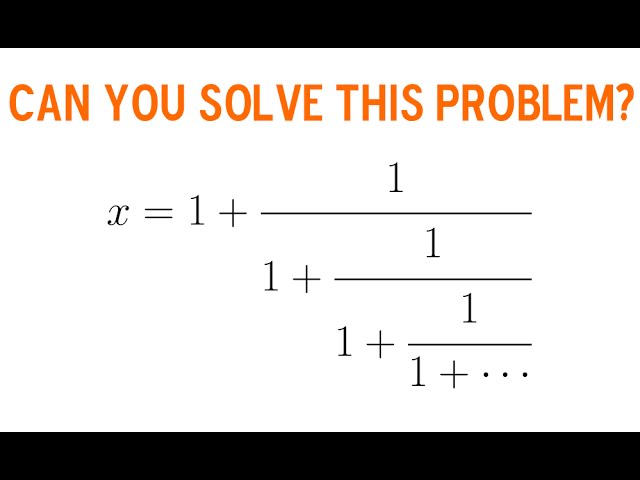
Can You Solve This Infinite Fraction?
Показать описание
What is the value of x= 1/(1 + 1/(1 + 1/(1 + ...)))? This continued infinite fraction has an interesting value!
Connect on social media. I update each site when I have a new video or blog post, so you can follow me on whichever method is most convenient for you.
My Books
Connect on social media. I update each site when I have a new video or blog post, so you can follow me on whichever method is most convenient for you.
My Books
This one weird trick will get you infinite gold - Dan Finkel
Can you solve the wizard standoff riddle? - Dan Finkel
Can You Solve This Infinite Fraction?
Can You Solve This? Infinite Radicals
How An Infinite Hotel Ran Out Of Room
Can you solve the three gods riddle? - Alex Gendler
Can You Solve x^x^x^... = 2? Infinite Exponent Tower Trick
Can You Solve this Infinite Series in 1 minute? | Quick & Simple Trick
How to Fix Valorant Infinite Loading Screen on PC | Valorant Loading Screen Bug
got an infinite answer in calculator
Can you solve the unstoppable blob riddle? - Dan Finkel
How to steal chocolate for free - infinite chocolate
Can you solve 'Einstein’s Riddle'? - Dan Van der Vieren
Infinite chocolate trick explained
Can you solve the rogue AI riddle? - Dan Finkel
Can You Solve the Poison Wine Challenge? | Infinite Series | PBS Digital
Why can't you divide by zero? - TED-Ed
The Infinite Hotel Paradox - Jeff Dekofsky
Can you solve the egg drop riddle? - Yossi Elran
Infinite cube glitch? 🤔
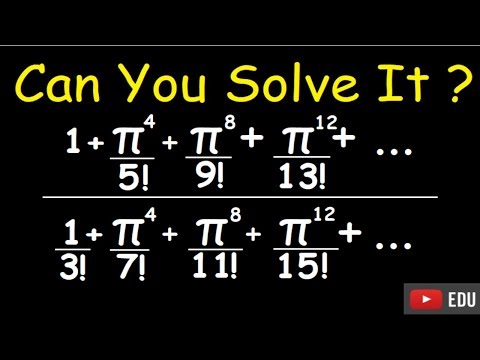
Can you solve this INFINITE SERIES Question ??
Can You Solve This Cambridge Exam Question? | Number Theory | Infinite Descent
Solve an infinite continued fraction with a simple answer!
Infinite Series - Numberphile
Комментарии
 0:05:08
0:05:08
 0:05:26
0:05:26
 0:01:30
0:01:30
 0:08:00
0:08:00
 0:06:07
0:06:07
 0:04:54
0:04:54
 0:01:00
0:01:00
 0:03:50
0:03:50
 0:03:04
0:03:04
 0:00:22
0:00:22
 0:03:43
0:03:43
 0:00:15
0:00:15
 0:05:13
0:05:13
 0:00:48
0:00:48
 0:05:13
0:05:13
 0:08:46
0:08:46
 0:04:51
0:04:51
 0:06:00
0:06:00
 0:04:47
0:04:47
 0:00:19
0:00:19
 0:09:29
0:09:29
 0:03:05
0:03:05
 0:01:40
0:01:40
 0:09:31
0:09:31