filmov
tv
Counting Integer Points in Polygons with Negative Numbers | A 'moral' Intro to Generating Functions
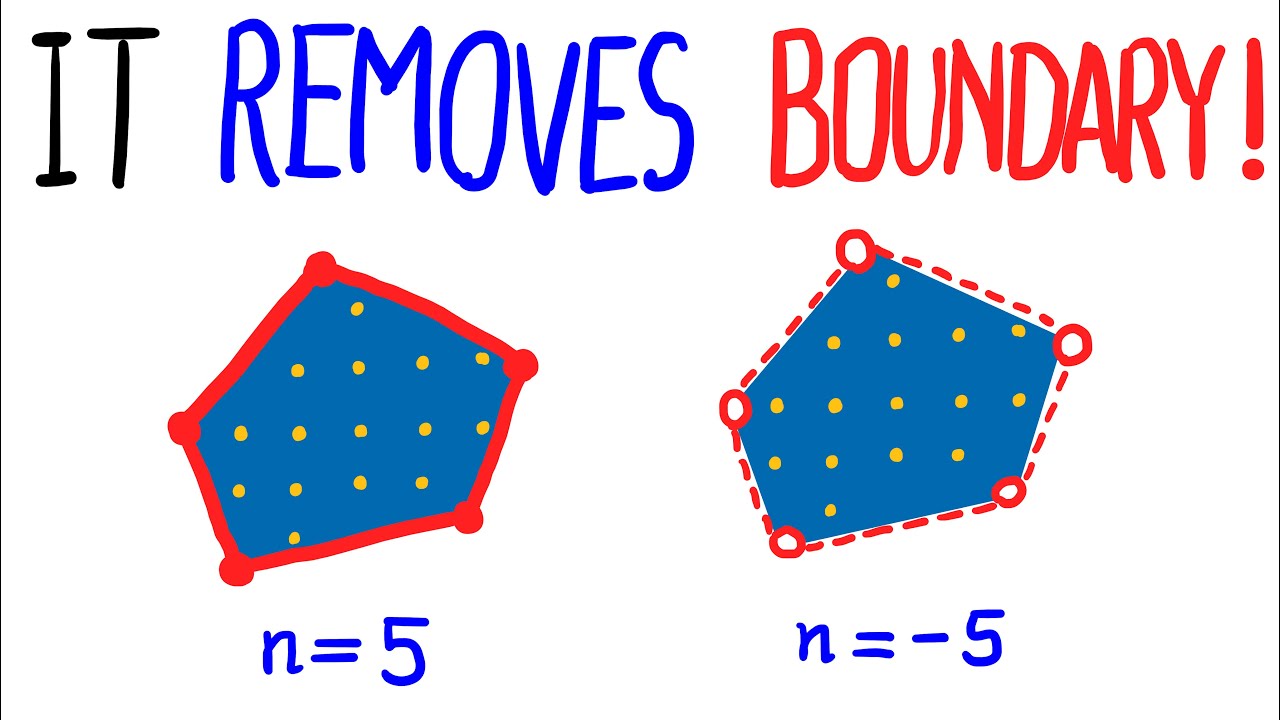
Показать описание
Turn on the subtitles for the BEST experience. :)
0:00 - Introduction
5:17 - Section 1: The What and Why of Generating Functions
15:18 - Section 2: Finding GFs for Lattice Counting Functions
34:11 - Section 3: Substituting Negative Numbers
47:46 - Section 4: The Finale
58:09 - Conclusion
I tried my best to motivate generating functions and give an insight to why the generating functions work the way they should. Why they are intimately connected to the structure of the objects we are counting and their relation with linear homogenous recurrence relations is explained. The ideas are illustrated along the lines of the proof of the Ehrhart polynomials and Ehrhart Macdonald reciprocity which is about counting lattice points in dilated polytopes and what exactly are “negative dilates”. Making this video has been in the back of my mind for a while. Thank you so much to Grant and James for hosting Summer of Math Exposition 2 which helped me with my motivation to put this video together. :)
Sorry that I skipped over many details for the video. Please comment the plot holes that you find. If you want a non-hand-wavy version, or if you are interested to know more about reciprocity nature in combinatorics, give this book a read (at least the first chapter, highly recommended):
Music in this video
Stock footage credit to
#generatingfunctions #recurrence #SoME2
0:00 - Introduction
5:17 - Section 1: The What and Why of Generating Functions
15:18 - Section 2: Finding GFs for Lattice Counting Functions
34:11 - Section 3: Substituting Negative Numbers
47:46 - Section 4: The Finale
58:09 - Conclusion
I tried my best to motivate generating functions and give an insight to why the generating functions work the way they should. Why they are intimately connected to the structure of the objects we are counting and their relation with linear homogenous recurrence relations is explained. The ideas are illustrated along the lines of the proof of the Ehrhart polynomials and Ehrhart Macdonald reciprocity which is about counting lattice points in dilated polytopes and what exactly are “negative dilates”. Making this video has been in the back of my mind for a while. Thank you so much to Grant and James for hosting Summer of Math Exposition 2 which helped me with my motivation to put this video together. :)
Sorry that I skipped over many details for the video. Please comment the plot holes that you find. If you want a non-hand-wavy version, or if you are interested to know more about reciprocity nature in combinatorics, give this book a read (at least the first chapter, highly recommended):
Music in this video
Stock footage credit to
#generatingfunctions #recurrence #SoME2
Комментарии
 0:59:48
0:59:48
 0:00:20
0:00:20
 0:00:58
0:00:58
 0:00:34
0:00:34
 0:00:16
0:00:16
 0:01:40
0:01:40
 0:01:00
0:01:00
 0:00:23
0:00:23
 0:00:55
0:00:55
 0:00:13
0:00:13
 0:10:50
0:10:50
 0:07:14
0:07:14
 0:08:34
0:08:34
 0:04:43
0:04:43
 0:00:06
0:00:06
 0:00:14
0:00:14
 0:13:13
0:13:13
 0:49:53
0:49:53
 0:00:14
0:00:14
 0:14:13
0:14:13
 0:00:24
0:00:24
 0:05:36
0:05:36
 0:00:11
0:00:11
 0:05:42
0:05:42