📚 How to find the partial derivative of a function (Part 1)

Follow us:

What you'll need:

Rules for finding partial derivatives of z=f(x,y)⁆:

1. To find f_x, regard y⁆ as a constant and differentiate f(x,y)⁆ with respect to x⁆.
2. To find f_y, regard x⁆ as a constant and differentiate f(x,y)⁆ with respect to y⁆.

Notation of partial derivatives:

If y=f(x,y)⁆, then:

f_x (x,y) ≡ f_x ≡ ∂f/∂x ≡ ∂/∂x f(x,y) ≡ D_x

○ ∂f/∂x is read "the partial derivative of f⁆ with respect to x⁆.
○ Do not confuse this symbol with 𝛿 (delta)

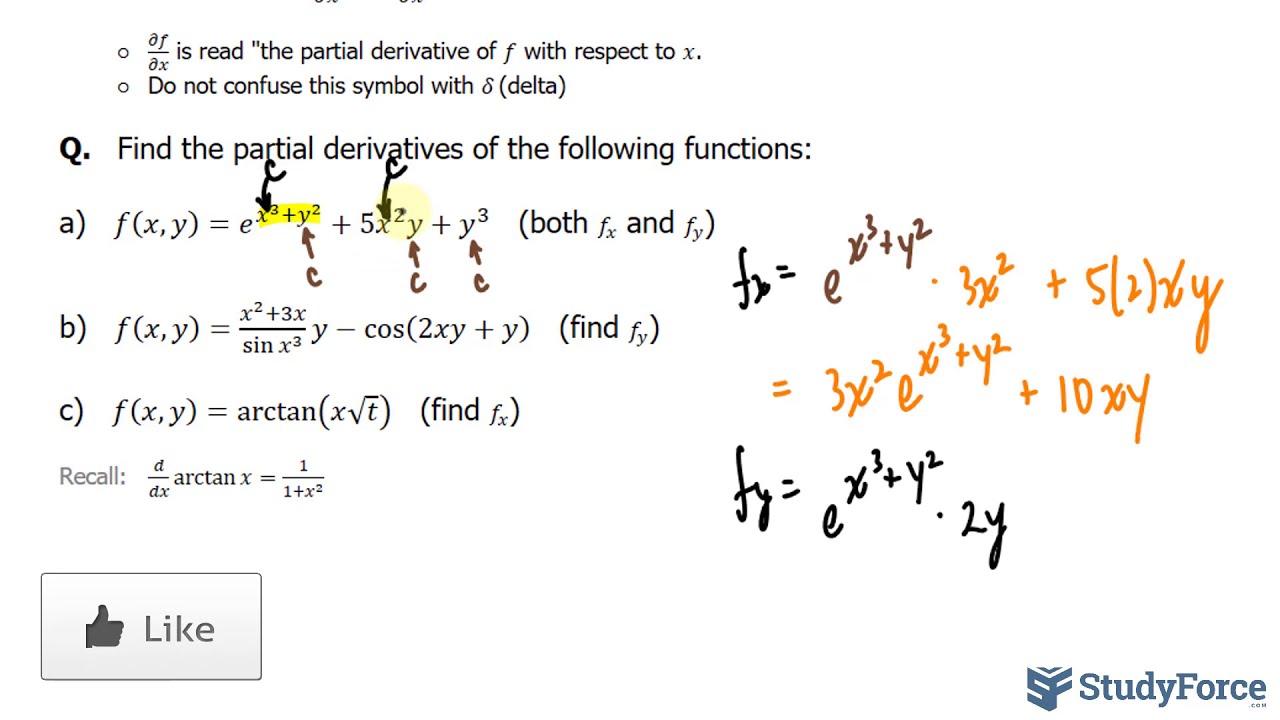
Q. Find the partial derivatives of the following functions:

a) f(x,y)=e^(x^3+y^2 )+5x^2 y+y^3 ⁆ (both f_x and f_y)

b) f(x,y)=(x^2+3x)/sin⁡〖x^3 〗 y−cos⁡(2xy+y) ⁆ (find f_y)

c) f(x,y)=arctan⁡(x√t) ⁆ (find f_x)

Recall: d/dx arctan⁡x=1/(1+x^2 )