filmov
tv
Compare the volume of sphere with cube | English

Показать описание
We often see spherical objects such as a football that are covered in cubical boxes. How much empty space is left in the box? Can we calculate it? Lets’ see show to do this!
For this, take a cubical box and a sphere such that sphere should perfectly fit inside the cube. To do this, take a cubical container of side 5 cm and a spherical metal ball having outer diameter 5 cm.
Initially place the sphere inside the cube. Now fill the entire remaining space inside the cube with water. After removing the sphere from the cubical box, you will observe that the amount of water remained inside the cubical container is approximately 50% of the entire volume of the cube.
Let’s check if this is mathematically correct.
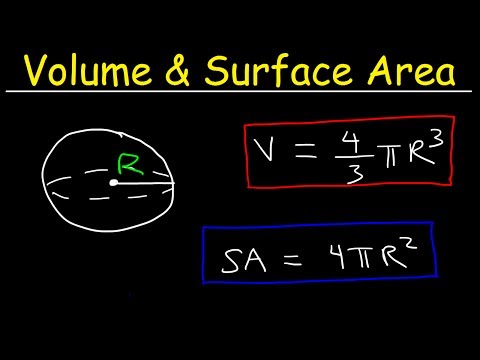
Volume of sphere is = 4/3 πr^3
= 4/3×3.142×r^3
≈ 4r^(3 ) (considering 3≈3.142)
Volume of cube=(side)^3
= (2r)^3 (side of cube = 2r)
= 8r^3
From this we can conclude that if diameter of sphere and side of cube are equal, then volume of cube is approximately double than the volume of sphere.
This simple activity allows us not only to compare volumes of different 3D shapes, but also is very surprising as most people do not think that so much space is left empty in such a box! For more such interesting activities visit our YouTube channel: IISER Pune Science Activity Centre. Have Fun!
Production team: Ashok Rupner, Chaitanya Mungi, Neha Apte, Prof. B. D. Chakradeo, Shraddha Bhurkunde, Mukund Mehendale
Camera: Vivek Kannadi, Kanchan Sharma
Music: Dr. Manasi Kulkarni
For this, take a cubical box and a sphere such that sphere should perfectly fit inside the cube. To do this, take a cubical container of side 5 cm and a spherical metal ball having outer diameter 5 cm.
Initially place the sphere inside the cube. Now fill the entire remaining space inside the cube with water. After removing the sphere from the cubical box, you will observe that the amount of water remained inside the cubical container is approximately 50% of the entire volume of the cube.
Let’s check if this is mathematically correct.
Volume of sphere is = 4/3 πr^3
= 4/3×3.142×r^3
≈ 4r^(3 ) (considering 3≈3.142)
Volume of cube=(side)^3
= (2r)^3 (side of cube = 2r)
= 8r^3
From this we can conclude that if diameter of sphere and side of cube are equal, then volume of cube is approximately double than the volume of sphere.
This simple activity allows us not only to compare volumes of different 3D shapes, but also is very surprising as most people do not think that so much space is left empty in such a box! For more such interesting activities visit our YouTube channel: IISER Pune Science Activity Centre. Have Fun!
Production team: Ashok Rupner, Chaitanya Mungi, Neha Apte, Prof. B. D. Chakradeo, Shraddha Bhurkunde, Mukund Mehendale
Camera: Vivek Kannadi, Kanchan Sharma
Music: Dr. Manasi Kulkarni
 0:02:32
0:02:32
 0:03:12
0:03:12
 0:04:14
0:04:14
 0:04:05
0:04:05
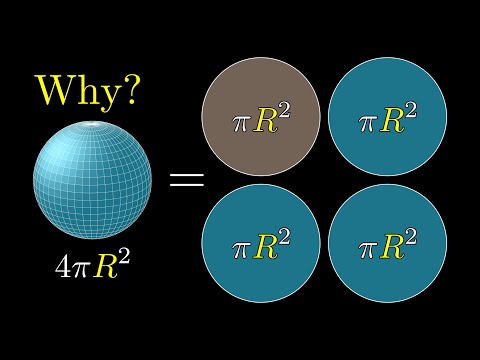 0:15:51
0:15:51
 0:01:57
0:01:57
 0:01:27
0:01:27
 0:00:43
0:00:43
 0:16:20
0:16:20
 0:12:36
0:12:36
 0:02:35
0:02:35
 0:01:27
0:01:27
 0:01:00
0:01:00
 0:04:46
0:04:46
 0:03:01
0:03:01
 0:03:20
0:03:20
 0:09:09
0:09:09
 0:00:49
0:00:49
 0:03:40
0:03:40
 0:07:07
0:07:07
 0:05:47
0:05:47
 0:03:22
0:03:22
 0:03:20
0:03:20
 0:11:09
0:11:09