filmov
tv
Problems with Quadratic Equations: A Review (GRE Quantitiative/Math Practice)
Показать описание
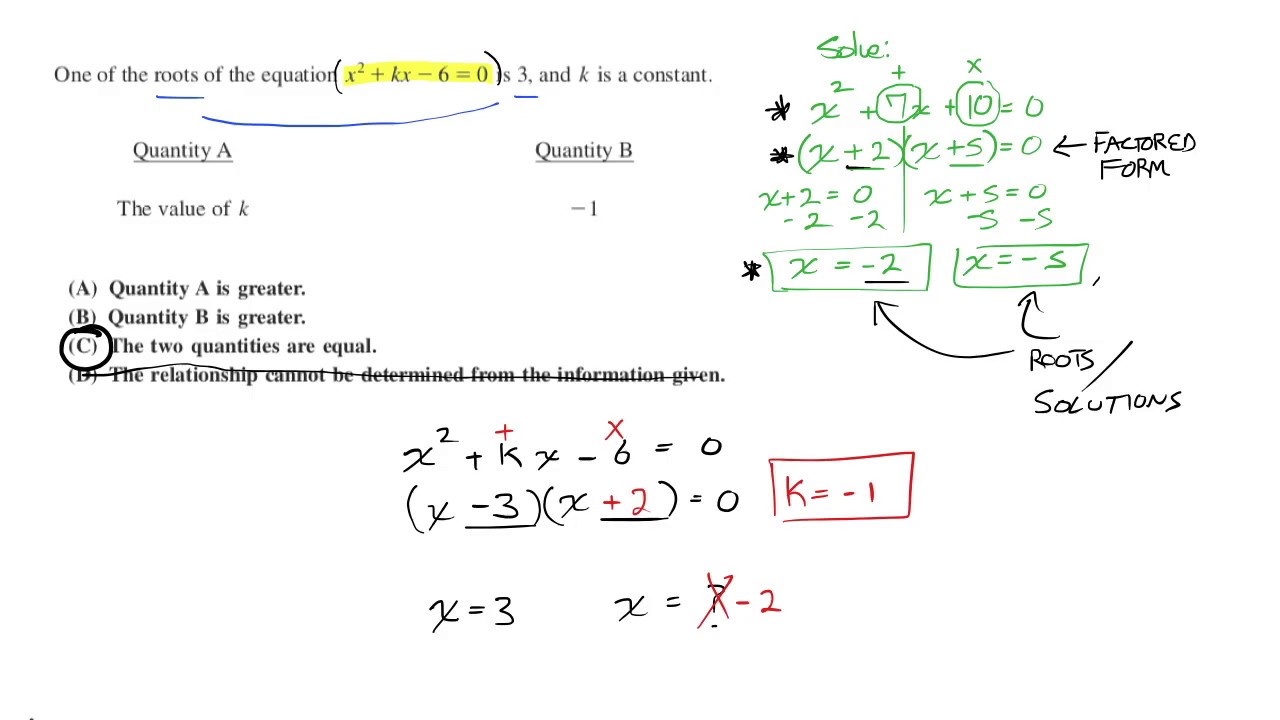
So listen, you could just plug in 3 for x. It is known to be a solution, which means you are allowed to substitute it. This leaves you with the equation:
(3)^2 +k(3) - 6 = 0
Try it; the solution to this one-variable equation is -1.
This method is obviously superior to the one I outlined here.
BUT, this is not always a possibility and you will often have to solve a real quadratic as I did in my review. You do NOT want to go into the GRE without the ability to solve a quadratic or identify the different steps that come out from the process, so please learn what you can from the video! You might thank me for looking at this the "advanced" way. Cheers!
One of the roots of the equation x^2 + kx - 6 is 3, and k is a constant.
(3)^2 +k(3) - 6 = 0
Try it; the solution to this one-variable equation is -1.
This method is obviously superior to the one I outlined here.
BUT, this is not always a possibility and you will often have to solve a real quadratic as I did in my review. You do NOT want to go into the GRE without the ability to solve a quadratic or identify the different steps that come out from the process, so please learn what you can from the video! You might thank me for looking at this the "advanced" way. Cheers!
One of the roots of the equation x^2 + kx - 6 is 3, and k is a constant.
Quadratic Word Problems (practice problems)
Example 4: Applying the quadratic formula | Quadratic equations | Algebra I | Khan Academy
How To Solve Quadratic Equations Using The Quadratic Formula
How To Solve Quadratic Equations By Factoring - Quick & Simple! | Algebra Online Course
Quadratic Equation Area Word Problem
APPLICATIONS FOR QUADRATIC EQUATIONS | Most Common Word Problems | Algebra 1
Word Problems - Solving Quadratic Equations by Factoring
QUADRATIC EQUATIONS: word problem
Tricky Math Problem on the SAT Exam #maths #shorts #satprep
Quadratic Equations – Practice Problems and Solutions
How to solve word problems with quadratic equations
09 - The Quadratic Formula Explained, Part 1 (Practice Problems & Solutions)
quadratic equations in every day life!
SOLVING PROBLEM INVOLVING QUADRATIC EQUATIONS || GRADE 9 MATHEMATICS
Maximum and Minimum Value Word Problems - Quadratic Equations
Solving Word Problem Involving Quadratic Equation
Quadratic Equations | Solve by factoring | Free Math Videos
Quadratic Turning Point | GCSE Maths 2025
Solving Quadratic Equations by Factoring #mathteachergon #quadraticequation #factoring #grade9math
SOLVING PROBLEMS INVOLVING QUADRATIC EQUATIONS || GRADE 9 MATHEMATICS Q1
Solving Problems Involving Quadratic Equations | Geometry | Area and Perimeter |
More Word Problems Using Quadratic Equations - Example 1
Most Important Problems on Quadratic Equations | Class 10th MATH'S Board Exam 🎯
Quadratic Formula Explained - Detailed Step by Step Practice Problem
Комментарии
 0:57:09
0:57:09
 0:05:53
0:05:53
 0:05:56
0:05:56
 0:12:29
0:12:29
 0:05:23
0:05:23
 0:08:34
0:08:34
 0:15:02
0:15:02
 0:12:09
0:12:09
 0:01:01
0:01:01
 0:19:48
0:19:48
 0:05:38
0:05:38
 0:25:59
0:25:59
 0:05:11
0:05:11
 0:07:27
0:07:27
 0:05:58
0:05:58
 0:07:08
0:07:08
 0:02:43
0:02:43
 0:00:54
0:00:54
 0:01:00
0:01:00
 0:15:48
0:15:48
 0:21:03
0:21:03
 0:04:59
0:04:59
 0:11:17
0:11:17
 0:19:30
0:19:30