filmov
tv
Proposition:- let f(x) be polynomial of degree 2 or 3 then f(x) is reducible if and only if it has
Показать описание
#fieldthoery
#field
#irreduciblepolynomials
#irreducible
#skclasses
#mscmaths
#mathematics
#bscmathematics
#bscmathematics
#ringtheory
#grouptheory
#theoremoffield
theorems of field theory
#field
#irreduciblepolynomials
#irreducible
#skclasses
#mscmaths
#mathematics
#bscmathematics
#bscmathematics
#ringtheory
#grouptheory
#theoremoffield
theorems of field theory
0:17:59
Proposition:- let f(x) be polynomial of degree 2 or 3 then f(x) is reducible if and only if it has
0:02:04
Let F be a finite field then there exists an irreducible polynomial of degree n over F
0:18:41
21 Let f(x) be an irreducible polynomial over a field F, then f(x) has a multiple root iff f'(x...
0:12:50
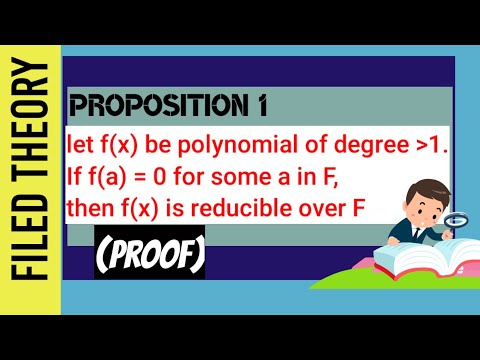
If polynomial has a root in F then it is reducible over F.
0:06:14
Field Theory 7, Zeros of an irreducible polynomial
0:09:28
Let f(x) be a polynomial of degree four having | IIT JEE Mains-2015 | Mathematics
0:14:22
Let f(x) be monic polynomial. If f(x) has a root in Q then a is in Z and a divides constant term
0:59:51
Abstract Algebra II: general polynomial set-up, 2-11-22 part 1
0:10:27
Number Theory | Polynomial Congruence Proposition
0:03:50
Eisenstein's Criterion Example (Polynomial is Irreducible over the Rationals ℚ)
0:17:35
Algebraic Extensions: The Minimal Polynomial (Algebra 3: Lecture 9 Video 2)
0:03:32
Let f(x) be a polynomial function of seconddegree. If f(a) =f(-a) and a, b, c are in A.P.then
0:19:08
BEST Examples to understand Reducible/Irreducible polynomials
0:06:58
Let f (x) be a polynomial of degree 8 such that F(r)=1/r, r=1,2,3,…,8,9, then (1)/(F(10)) = | 1...
0:07:53
Reducible Polynomial with Example | Finite Fields and Polynomial
0:26:44
4.4 Polynomial Functions, Roots, and Reducibility
0:07:47
Let f(x) be a polynomial of degree 3 such that f(k)=-2/k for k = 2,3,4,5. the value of 52-10f(10)
0:06:22
If f(x) has roots in F then polynomial is reducible over R proof
0:27:42
Algebraic elements form a field
0:31:09
Symmetric polynomials: definition and examples
0:23:11
Algebraic Number Theory 14: The Minimal Polynomial of Integral Elements
0:43:18
The Method of Interlacing Polynomials - A
0:00:15
Cosplay by b.tech final year at IIT Kharagpur
0:37:45
Discrete Mathematical Structures, Lecture 2.6: Propositions over a universe
Комментарии