Chemical Thermodynamics 3.12 - Heat Capacity

Short physical chemistry lecture on the constant pressure and constant volume heat capacities of ideal gases.

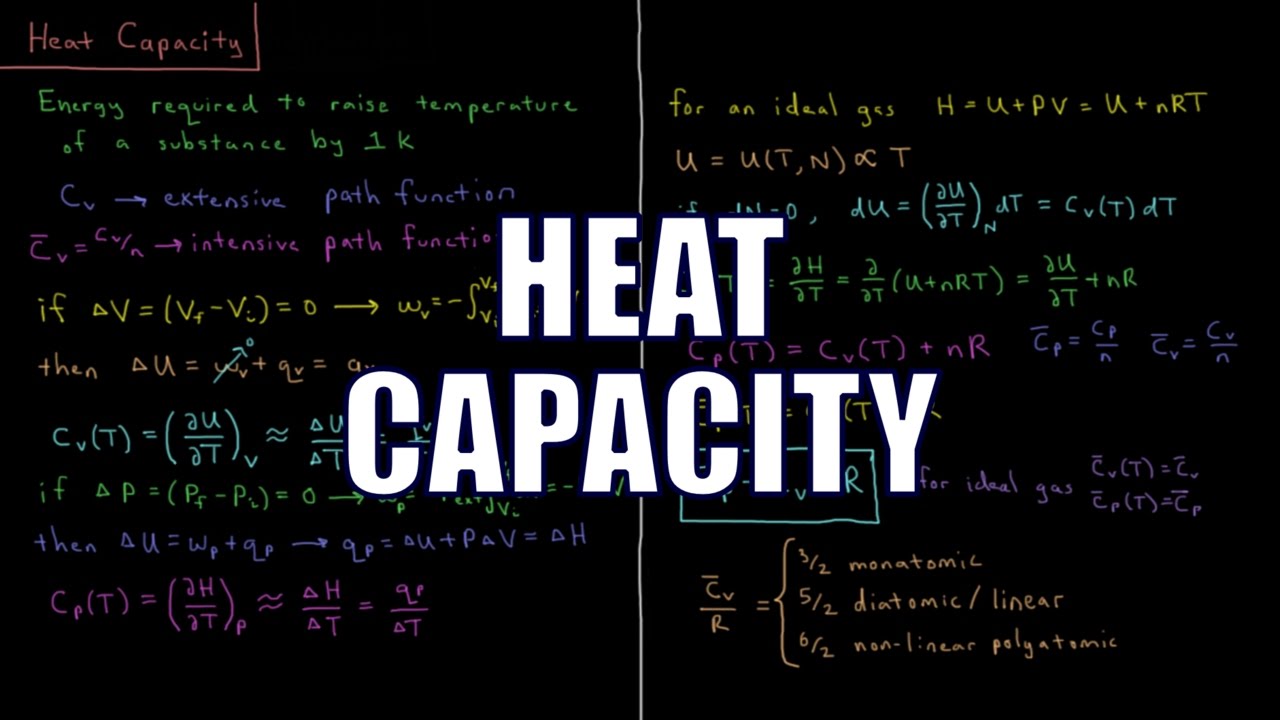
The constant volume heat capacity of a system is defined as the partial derivative of the internal energy with respect to temperature. The constant pressure heat capacity of a system is defined as the partial derivative of the enthlapy with respect to temperature.

--- About TMP Chem ---

All TMP Chem content is free for everyone, everywhere, and created independently by Trent Parker.

--- Video Links ---

--- Social Links ---

--- Equipment ---

Microphone: Blue Yeti USB Microphone

Drawing Tablet: Wacom Intuos Pen and Touch Small

Drawing Program: Autodesk Sketchbook Express

Screen Capture: Corel Visual Studio Pro X8

[bohanxu]

About the mono/di/poly-atomic molecule heat capacity, you should add that you are ignoring vibrational contribution to heat capacity. Good video overall still.

[nkyu]

Let me ask you how the equation C_P(T)=C_V(T) +nR is derived.
This equation came from C_P(T)=dH/dT { I think it should be (dH/dT)_P } = (du/dT)_P +nR.
and I wonder how (du/dT)_P term ends up being C_V(T) which is (du/dT)_V.

Is it because (du/dT)_P and (du/dT)_V are same for the ideal gas,
OR they are just same in that they are partial dervatives regardless of subscript P and V, which is nothing to do with the value and is only for the purpose of clarification?

[nkyu]

in the third line of the right side of the screen, you said du=(du/dT)_N *dT = C_V *dT.
how does (du/dT)_N equal C_V? C_V equals (du/dT)_V.

[safwanahmad]

please i need example about it..not prove

[nkyu]

why is C independent of T for ideal gas?