filmov
tv
What are the formulas for the sum and difference of two cubes
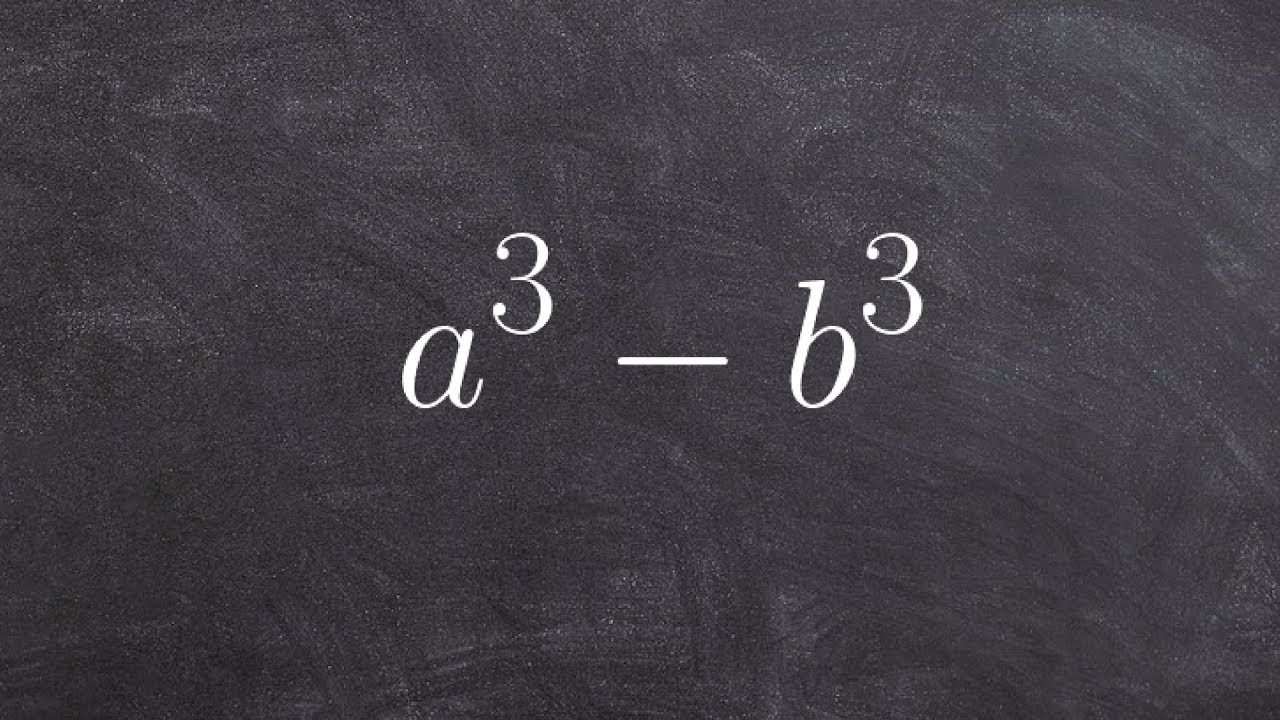
Показать описание
👉 Learn how to factor polynomials using the sum or difference of two cubes. A polynomial is an expression of the form ax^n + bx^(n-1) + . . . + k, where a, b, and k are constants and the exponents are positive integers. To factor an algebraic expression means to break it up into expressions that can be multiplied together to get the original expression.
Sum of two cubes method of factoring is used when the polynomial has two terms which can be expressed as the cube of numbers (or expressions) and the plus sign in between the two terms while the difference of two cubes method of factoring is used when the polynomial has two terms which can be expressed as the cube of numbers (or expressions) and the minus sign is between the two terms.
To factor a polynomial using the sum of two cubes, we express the polynomial as a product of the sum of the cube roots of the two original terms of the polynomial and the difference of the sum of the square of the cube roots of the terms and the product of the cube roots of the terms, i.e. (a^3 + b^3) = (a + b)(a^2 - ab + b^2). To factor a polynomial using the difference of two cubes, we express the polynomial as a product of the difference of the cube roots of the two original terms of the polynomial and the sum of the square of the cube roots of the terms and the product of the cube roots of the terms, i.e. (a^3 - b^3) = (a - b)(a^2 + ab + b^2).
If the original polynomial has a common factor, it is helpful to first factor out the common factor of the expression before factoring using the sum/difference of two cubes method.
Organized Videos:
✅How to Factor a Polynomial
✅How to factor a polynomial | Learn about
✅How to factor out the GCF from a polynomial
✅How to factor a perfect square polynomial
✅How to factor a polynomial by difference of two squares
✅How to factor a polynomial by sum or difference of two cubes
✅How to factor out an expression from a polynomial
✅How to factor a polynomial by grouping with two variables
✅How to factor a polynomial by grouping
✅How to factor a polynomial to a higher power
Connect with me:
#polynomials #brianmclogan
Sum of two cubes method of factoring is used when the polynomial has two terms which can be expressed as the cube of numbers (or expressions) and the plus sign in between the two terms while the difference of two cubes method of factoring is used when the polynomial has two terms which can be expressed as the cube of numbers (or expressions) and the minus sign is between the two terms.
To factor a polynomial using the sum of two cubes, we express the polynomial as a product of the sum of the cube roots of the two original terms of the polynomial and the difference of the sum of the square of the cube roots of the terms and the product of the cube roots of the terms, i.e. (a^3 + b^3) = (a + b)(a^2 - ab + b^2). To factor a polynomial using the difference of two cubes, we express the polynomial as a product of the difference of the cube roots of the two original terms of the polynomial and the sum of the square of the cube roots of the terms and the product of the cube roots of the terms, i.e. (a^3 - b^3) = (a - b)(a^2 + ab + b^2).
If the original polynomial has a common factor, it is helpful to first factor out the common factor of the expression before factoring using the sum/difference of two cubes method.
Organized Videos:
✅How to Factor a Polynomial
✅How to factor a polynomial | Learn about
✅How to factor out the GCF from a polynomial
✅How to factor a perfect square polynomial
✅How to factor a polynomial by difference of two squares
✅How to factor a polynomial by sum or difference of two cubes
✅How to factor out an expression from a polynomial
✅How to factor a polynomial by grouping with two variables
✅How to factor a polynomial by grouping
✅How to factor a polynomial to a higher power
Connect with me:
#polynomials #brianmclogan
 0:03:09
0:03:09
 0:10:22
0:10:22
 0:04:22
0:04:22
 0:09:43
0:09:43
 0:12:29
0:12:29
 0:42:22
0:42:22
 0:27:19
0:27:19
 0:23:04
0:23:04
 0:18:36
0:18:36
 0:32:23
0:32:23
 0:00:05
0:00:05
 0:04:16
0:04:16
 0:04:17
0:04:17
 0:23:23
0:23:23
 0:09:19
0:09:19
 0:04:12
0:04:12
 0:02:51
0:02:51
 0:18:37
0:18:37
 0:15:50
0:15:50
 0:15:52
0:15:52
 0:22:38
0:22:38
 0:06:51
0:06:51
 0:00:14
0:00:14
 0:14:30
0:14:30