How to Add and Subtract Complex Numbers | Complex Analysis

How do we add and subtract complex numbers? We go over just that in today's lesson on the addition and subtraction of complex numbers!

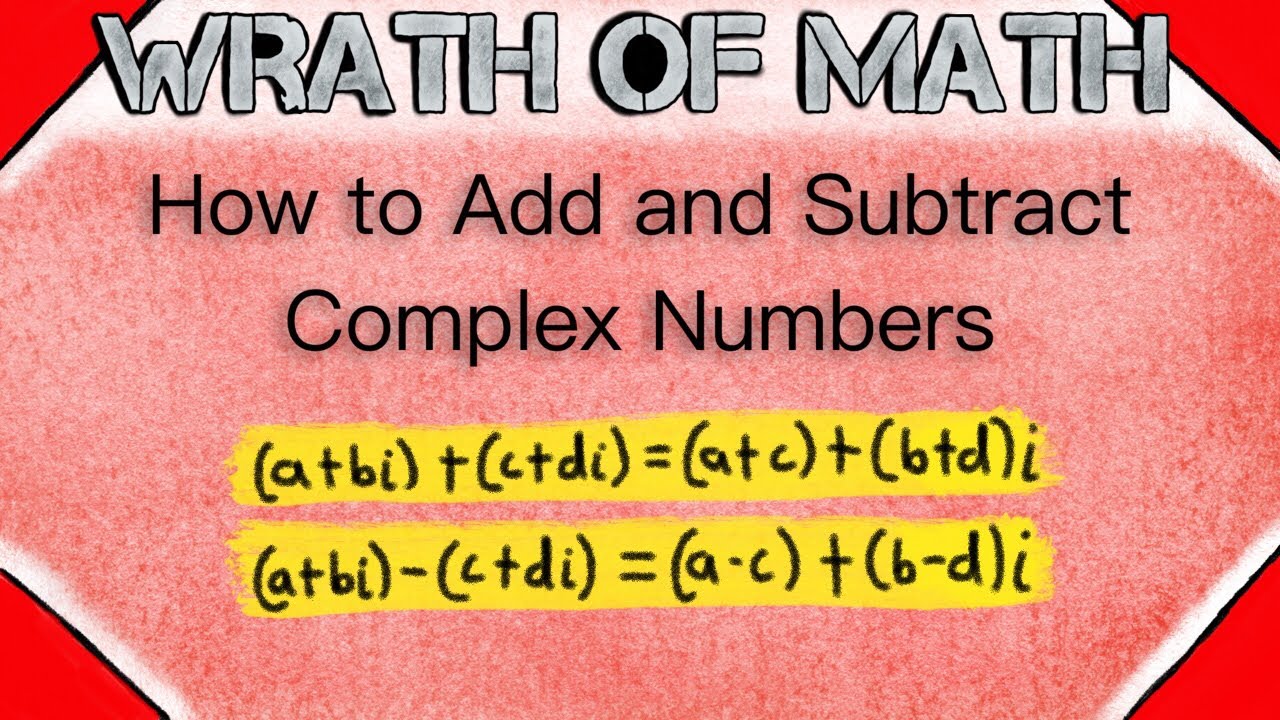
Consider two complex numbers (a + bi) and (c + di). Now we calculate their sum, (a + bi) + (c + di). First, we drop the parentheses, which are unimportant. So we have (a + bi) + (c + di) = a + bi + c + di. Then all we have to do is add like terms, so we have a + bi + c + di = (a + c) + (b + d)i, where the parentheses are just there to distinguish between the real part (a + c) and the imaginary part (b + d). Thus, for any two arbitrary complex numbers (a + bi) and (c + di), their sum (a + bi) + (c + di) is equal to (a + c) + (b + d)i. All we have to do is add the real parts and add the imaginary parts multiplied by i.

Now let's look at a concrete example of addition.

Let's consider the sum (2 + 3i) + (4 + 6i). Then, we have (2 + 3i) + (4 + 6i) = 2 + 3i + 4 + 6i = 6 + 9i. All we have to do is add like terms.

Consider another example, (3 + 5i) + (-2 - 3i). Think of the subtraction in these problems as the addition of negative numbers. The advantage to this is that addition is commutative, so writing subtraction as addition allows us to add the numbers in whatever order we please. As an example, 2 - 3 is the same as 2 + (-3). And 2 + (-3) is the same as (-3) + 2. but 2 - 3 is not the same as 3 - 2. Going back to our example, we have (3 + 5i) + (-2 - 3i) = 3 + 5i + (-2) + (-3i). Then again, all we need to do is add like terms. So we have 3 + 5i + (-2) + (-3i) = 1 + 2i. All we do, put simply, is add the real parts of the complex numbers, add the imaginary parts multiplied by i, then add those together to get our final sum.

Now let's look at subtraction.

Consider two complex numbers (a + bi) and (c + di). Now we calculate their difference, (a + bi) - (c + di). First, we drop the parentheses, but this time in order to do so we first need to distribute the negative. So we have (a + bi) - (c + di) = a + bi - c - di, we distributed the negative through (c + di) and dropped the parentheses. Then all we have to do is add like terms, just like before, so we have a + bi - c - di = (a - c) + (b - d)i. Again, it may help to think of the subtraction as the addition of negatives. Thus, for any two arbitrary complex numbers (a + bi) and (c + di), their difference (a + bi) - (c + di) is equal to (a - c) + (b - d)i. Notice it is just the difference of their real parts and the difference of their imaginary parts times i.

Now let's look at a concrete example of subtraction.

Let's consider the difference (2 + 3i) - (4 + 6i). Then, we have (2 + 3i) - (4 + 6i) = 2 + 3i - 4 - 6i = -2 - 3i. All we have to do is distribute the negative, then add like terms (whether it be adding negatives or positives).

Consider another example, (3 + 5i) - (-2 - 3i). Remember that a negative times a negative is a positive, and we have to distribute. So we have (3 + 5i) - (-2 - 3i) = 3 + 5i + 2 + 3i. Then again, all we need to do is add like terms. So we have 3 + 5i + 2 + 3i = 5 + 8i. All we do, put simply, is subtract the real parts of the complex numbers, subtract the imaginary parts multiplied by i, then add those together to get our final difference.

I hope you find this video helpful, and be sure to ask any questions down in the comments!

********************************************************************
The outro music is by a favorite musician of mine named Vallow, who, upon my request, kindly gave me permission to use his music in my outros. I usually put my own music in the outros, but I love Vallow's music, and wanted to share it with those of you watching. Please check out all of his wonderful work.

********************************************************************

+WRATH OF MATH+

Follow Wrath of Math on...