Sketching Rational Functions Having Removable Discontinuities

Follow us:

A removable discontinuity is a point on the graph that is undefined or does not fit the rest of the graph. If you find a restriction in the function that can be canceled out, this will result in a hole leading to a removable discontinuity.

Q1. Sketch the graph of f(x)=(x^2−1)/(x+1), and find the coordinates of all removable discontinuities.

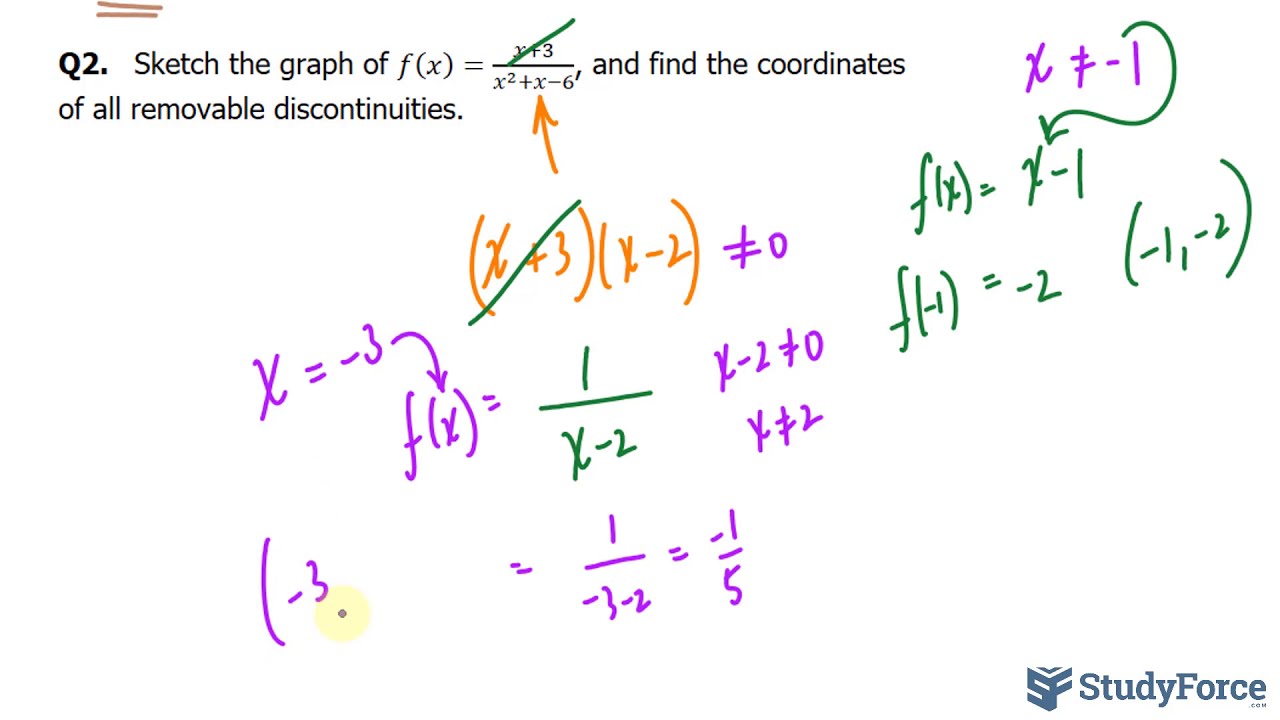
Q2. Sketch the graph of f(x)=(x+3)/(x^2+x−6), and find the coordinates of all removable discontinuities.