filmov
tv
Factoring trinomials with a non-1 leading coefficient by grouping
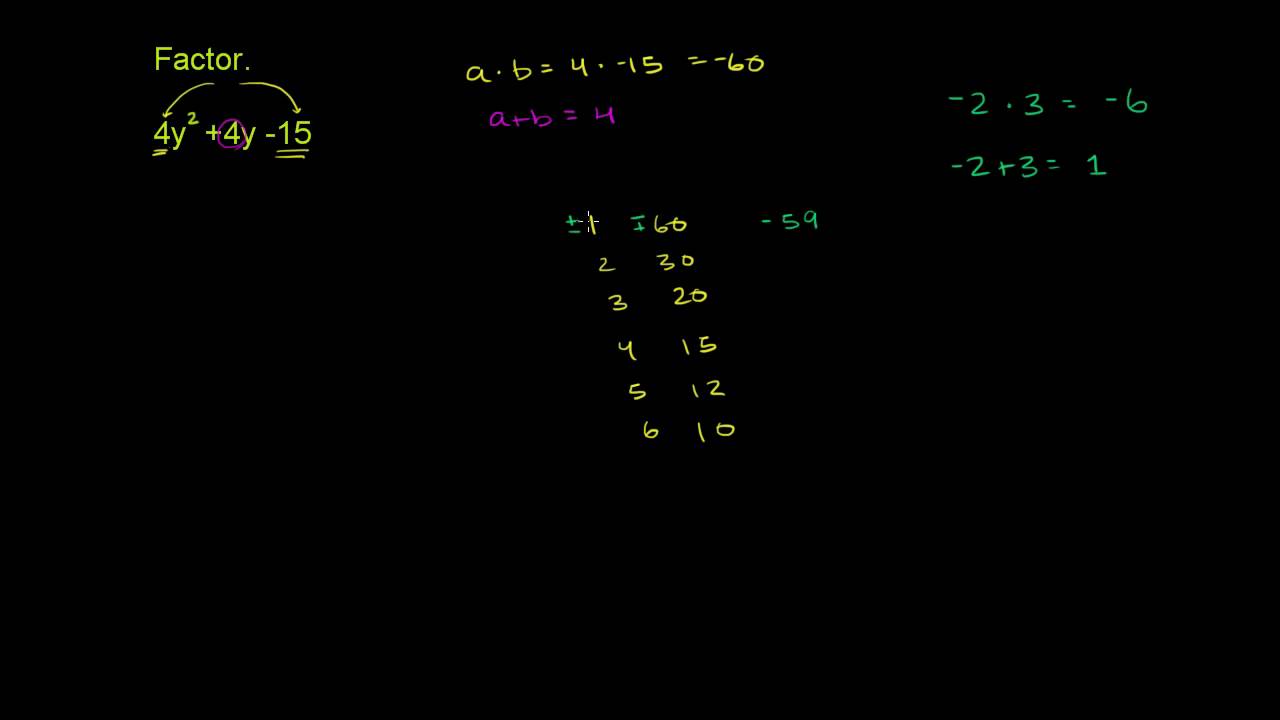
Показать описание
u12_l2_t1_we3 Factoring trinomials with a non-1 leading coefficient by grouping
Factoring trinomials with a non-1 leading coefficient by grouping
Learn How to Factor a Trinomial in Your Head When a is not Equal to One
Factoring Trinomials
Factoring Trinomials with Leading Coefficient not 1 (fast way)
Example 1: Factoring trinomials with a common factor | Algebra II | Khan Academy
How to Factor a Trinomial When a is Not 1 Explained! (AC Method)
*Quick* How to Factor Trinomials when the Leading Coefficient is not 1 | Solve by Factoring
Factoring Trinomials with Leading Coefficient not 1 AC Method
Chapter 5 - Section 5.6.- Factoring Trinomials Part 2
How to Factor Trinomials with Reverse FOIL (Simple AC Algorithm Method when a is not 1)
Learn the AC METHOD in 2 MINUTES | Factoring Trinomials | Algebra 2
Box Method of Factoring Trinomials (In Under 2 Minutes)
Factoring when a is greater than one
Ex: Factor Trinomials When A is NOT Equal to 1 - Trial and Error Method
FASTEST way to factor a trinomial! #shorts
Factoring Quadratics - When my factors don’t add up to b!
Factoring using a perfect square trinomial when a is not 1
How to Factor a Trinomial where a does not equal 1 (ax²+bx+c)
How to identify when a trinomial is not factorable
How to Factor and Solve Quadratic Trinomials - No Fuss Factoring - Quick Math Trick
Grade 8 - Factoring Trinomials in the form of ax² + bx + c when x is not equal to 1.
Factoring a trinomial a = 1
Xbox factoring trinomials where a is not 1
Factoring Trinomials, a is not 1(HD)
Комментарии
 0:05:39
0:05:39
 0:04:28
0:04:28
 0:04:17
0:04:17
 0:02:05
0:02:05
 0:05:02
0:05:02
 0:08:47
0:08:47
 0:04:10
0:04:10
 0:02:50
0:02:50
 0:23:19
0:23:19
 0:03:20
0:03:20
 0:02:45
0:02:45
 0:01:36
0:01:36
 0:03:50
0:03:50
 0:05:23
0:05:23
 0:00:55
0:00:55
 0:02:01
0:02:01
 0:01:16
0:01:16
 0:06:38
0:06:38
 0:01:38
0:01:38
 0:03:54
0:03:54
 0:10:38
0:10:38
 0:04:31
0:04:31
 0:15:36
0:15:36
 0:03:02
0:03:02