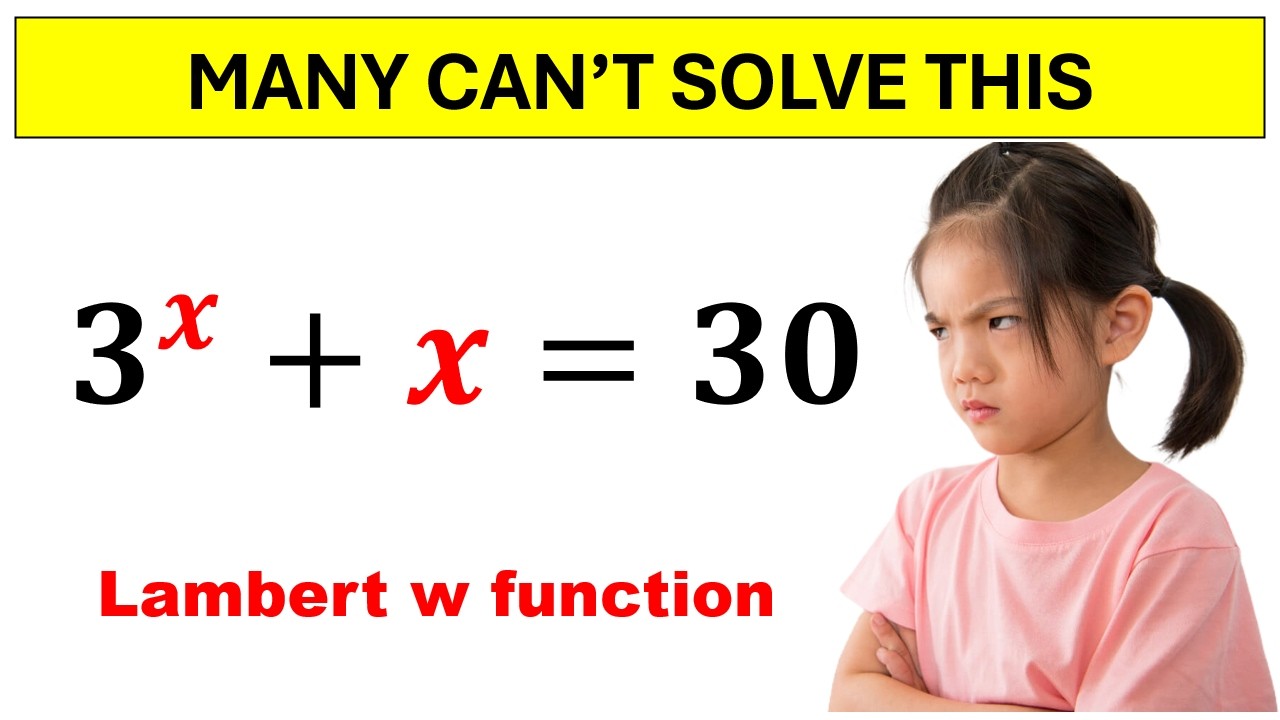
Math Olympiad Exam | Lambert W Function

Welcome to MathMinds, the place to be for all things math! We create short, engaging videos that explore the beauty and wonder of numbers and mathematics. Our videos feature visualizations of complex math concepts, using animations and graphics to help make even the most challenging topics easy to understand.

Our channel covers a wide range of mathematical topics, from puzzles and brain teasers to advanced concepts like calculus and statistics. We also provide video lessons on high school math topics such as algebra and geometry, perfect for students looking to supplement their learning or anyone who wants to brush up on the basics.

Our goal is to make math accessible and enjoyable for everyone, so we're always looking for new and exciting ways to present mathematical ideas.

So if you're ready to dive into the world of math and explore its beauty and complexity, make sure to subscribe to our channel and join the MathMinds community today!

If you like this video about

Math Olympiad Exam | Lambert W Function

Please Like & Subscribe my channel.

#imo #Math #mathequations

[omarkandou]

We can just notice that 3power(3)+3=30. Since the function is increasing. Then 3 is the unique solution.

[mikeinjapan]

Only mathematicians appreciate this elegant solution! Nice introduction to Lambert function.

[Tempest_]

Everyone is acting like what he did in this video uses unnecessary steps, but the goal of this video is not just to teach you how to solve 3^x+x=30, it is to teach you how to use the Lambert function to solve for x in similar situations.

[tungyeeso]

This is my approach: Plot a graph using y=3^x and y=30-x, the solution can be found where the curves meet. Simple and easy.

[khundeejai]

Moving x to the right side, we get 3^x = -x+30. The exponential function is increasing while the linear function is decreasing and there is only one intersection. It's obvious that x=3 is the solution.

[vspvilw]

I hope my car navigation NEVER gives me the directions like this

[luftmaxsa]

In Russia I haven't even been told about the Lambert W Function. Instead I've been taught multiple times about Gauss functions, and many other fundamentals of mathematical framework in many aspects (calculus, vector calculus, complex analysis, tensor analysis)... but W-function is what I've never heard of before...

[TheCktulhu]

3^x + x = 3^3 + 3
x=3
30 const ; 3^x + x is increasing func -> hence 1 intersection and 1 solution
selection method 3 root.

[Packerfan]

Define f:R -> R by f(x) = 3^x + x - 30.

Note that f is differentiable (and thus, continuous) on (-inf, inf) and f(3) = 0.

Note that f '(x) = 3^x ln 3 + 1 > 0 on (-inf, inf) since 3^x > 0 on (-inf, inf).

Suppose by contradiction there exists a in R such that f(a) = 0 and a != 3. If a < 3, then by the Mean Value Theorem there exists c in (a, 3) such that f '(c) = [f(3) - f(a)] / [3 - a] = 0, but this is a contradiction since f '(x) > 0 on (-inf, inf). We can use the MVT to show that a > 3 also leads to a contradiction. Therefore, f has one root at x = 3.

[DandoPorsaco-hozs]

This method works if you can split 30 into two numbers x and y, such that x + y = 30 and 3^x = y (ie. 3 +27 = 30 and 3^x = 27), where x must be the solution you are trying to calculate. In other words, you need the solution of the problem in order to split 30 and use this complicated method… to find that number you just used.
For example, if you try to solve 3^x + x = 40, you just need to split in your head 40 into 3.279887… and 36.72011..., both with infinite decimals, and since 3.279887… + 36.72011... = 40 and 3^3.279887… = 36.72011..., you can use this method to find the solution, which is 3.279887…, the number you used to find that very number.
Brilliant method!

[guitarbap]

First glance you all can notice X= 3, and its done. Fact 😂

[nasrullahhusnan]

It is simpler to solve the problem using modular algebra.
Take modulo 3:
mod(3^x, 3)+mod(x, 3)=mod(30, 3)
[mod(3, 3)]^x+mod(x, 3)=0
0+mod(x, 3)=0 --> mod(x, 3)=0
It means that x=3k where k is any integer. Plugging it back to the given equation:
3^(3k)+3k=30
Divide by 3: 3^(3k-1)+k=10
=3²+1
Comparing both sides we get k=1
Thus x=3. As check:
LHS=3^x+x=3³+3
=27+3
=30 equals to RHS

[VlesSiah]

since 3^x and x are both increasing functions the function f(x)=3^x+x is in an increasing function. f(0)=1<30 therefore x >0
for positive x, 3^x+x=30 <=> ln(3^x+x)=ln(30) <=> x*ln(3)*ln(x)=ln(30) <=> x*ln(x)= ln(27) <=> x*ln(x)=3ln(3) <=> x=3

[thessalonician]

Because of the sum (30), x should be either 2 or 3. As 3^2+2=11 and 3^3+3=30, then by inspection x = 3.. It won't take more than 1' to get the solution.
You can get there easily just by trial..

[Morpheye]

I gave up on contest math years ago being not nearly brilliant enough for it, just got recommended this and it absolutely blew my mind❤

[yhbygrn]

я придумал два способа немного легче...
1 способ: перенести х вправо и нарисовать две функции у=3^х и у=30-х, найти пересечение и всё
2 способ: снова перенести х и получить: 3^х=30-х, увидеть, что одна функция возрастает, а другая — убывает, следовательно, есть хотя бы одно решение и методом подбора нашёл, что х=3

[Jay_Richardson]

3 to the power of 3 = 27 + 3. Pretty easy X = 3. Dont need a big ass page explaining it all re writing it multiple times. Just work through to the powers 3x0, 3x3, 3x3x3 = Remainder left 3.

[christiansmakingmusic]

Btw x is congruent to zero mod three, so you can rewrite it as 27^x+3x=30, and immediately we see using the canonical homomorphism between Z[3] and Z/3Z, that the new x must be one, and the original must have been 3.

[SttNguyenPhuocTrongA]

Giải phương trình :3^x+x=30
+) Tập xác định:D=R
+) Phương trình trên tương đương với phương trình sau:
3^x+x-30=0
Xét hàm số f(x) =3^x+x-30, x∈R
=>f'(x) =(3^x)ln3+1>0∀x
=>f(x) đồng biến trên R
=>phương trình f(x) =0 có tối đa 1 nghiệm trên tập số thực
+) Mà f(3) =0
=>X=3 là 1 nghiệm duy nhất của phương trình f(x) =0
Kết luận: tập nghiệm của phương trình là S={3}

[sukmble]

As a generalization, the method presented always works if in the equation a^x + x = b one has b = a^a + a, with a solution x = a. Try it out with some numbers or repeat the derivation as in the video.