filmov
tv
F. Luo - An introduction to discrete conformal geometry of polyhedral surfaces (Part 3)
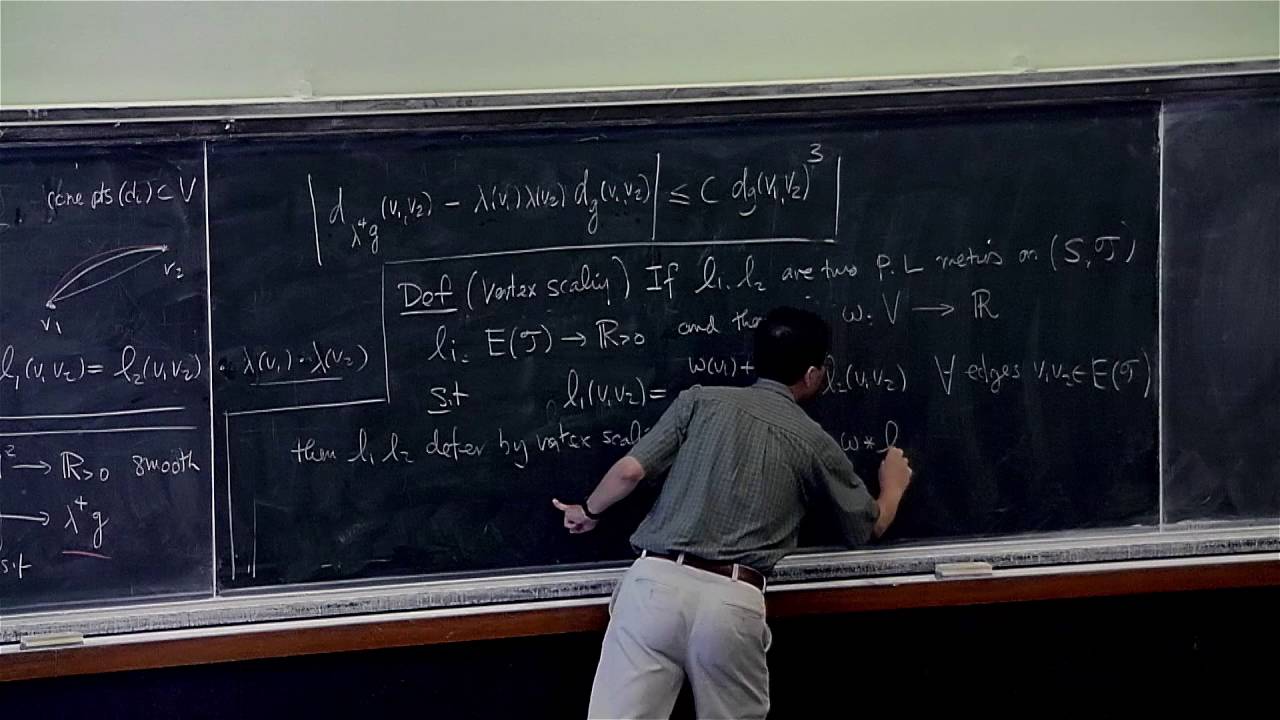
Показать описание
The goal of the course is to introduce some of the recent developments on discrete conformal geometry of polyhedral surfaces. We plan to cover the following topics.
- The Andreev-Koebe-Thurston theorem on circle packing polyhedral metrics and Marden-Rodin’s proof
- Thurston’s conjecture on the convergence of circle packings to the Riemann mapping and its solution by Rodin-Sullivan
- Finite dimensional variational principles associated to polyhedral surfaces
- A discrete conformal equivalence of polyhedral surfaces and its relationship to convex polyhedra in hyperbolic 3-space
- A discrete uniformization theorem for compact polyhedral surfaces
- Convergence of discrete conformality and some open problems
- The Andreev-Koebe-Thurston theorem on circle packing polyhedral metrics and Marden-Rodin’s proof
- Thurston’s conjecture on the convergence of circle packings to the Riemann mapping and its solution by Rodin-Sullivan
- Finite dimensional variational principles associated to polyhedral surfaces
- A discrete conformal equivalence of polyhedral surfaces and its relationship to convex polyhedra in hyperbolic 3-space
- A discrete uniformization theorem for compact polyhedral surfaces
- Convergence of discrete conformality and some open problems
 1:32:36
1:32:36
 1:26:54
1:26:54
 1:25:09
1:25:09
 1:33:21
1:33:21
 1:30:41
1:30:41
 0:02:01
0:02:01
 1:04:02
1:04:02
![[PADL'23] Fluo: A](https://i.ytimg.com/vi/4OMiHOcia8k/hqdefault.jpg) 0:28:22
0:28:22
 0:00:18
0:00:18
 0:00:17
0:00:17
 0:09:44
0:09:44
 0:36:58
0:36:58
 0:00:30
0:00:30
 0:01:05
0:01:05
 0:01:22
0:01:22
 0:02:52
0:02:52
 0:03:17
0:03:17
 0:12:04
0:12:04
 0:00:31
0:00:31
 0:00:06
0:00:06
 0:00:09
0:00:09
 0:00:10
0:00:10
 0:00:15
0:00:15
![[HD] intro fluo](https://i.ytimg.com/vi/vw3DR5Sme7E/hqdefault.jpg) 0:00:11
0:00:11