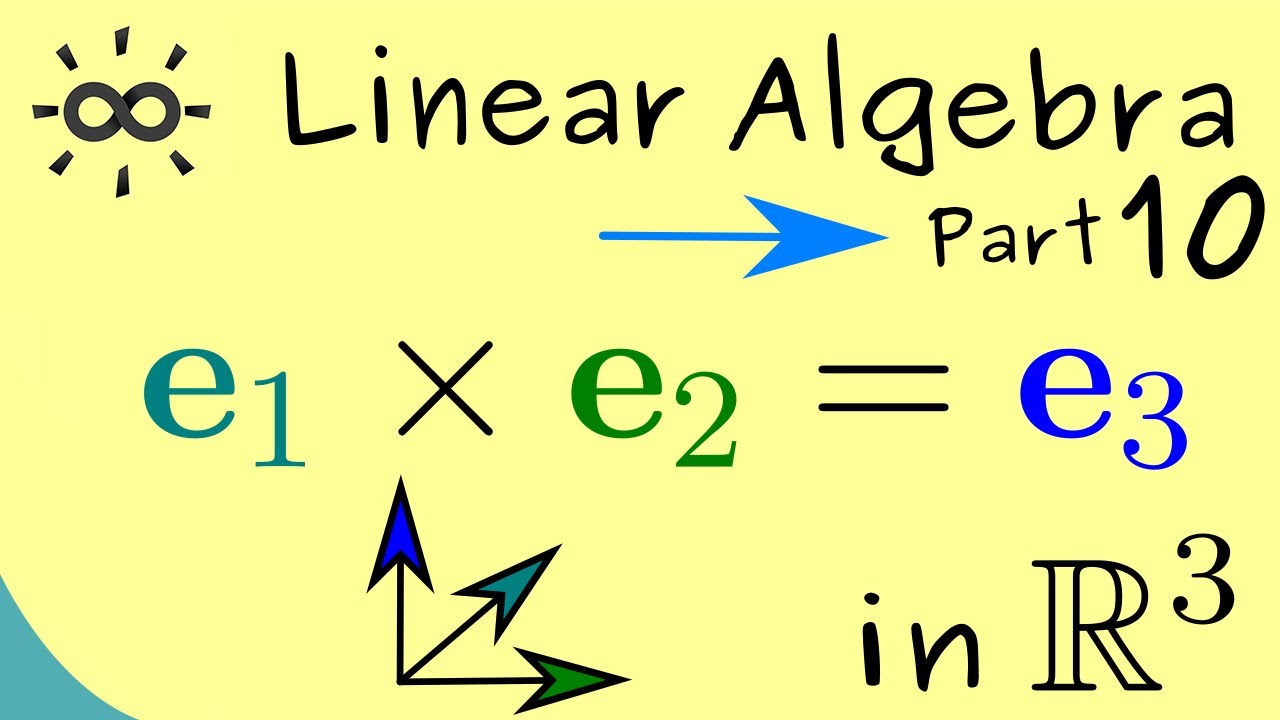
Linear Algebra 10 | Cross Product

Thanks to all supporters! They are mentioned in the credits of the video :)

This is my video series about Linear Algebra. I hope that it will help everyone who wants to learn about it.

#LinearAlgebra
#Vectors
#Matrices
#MachineLearning
#Eigenvalues
#Calculus
#Mathematics
x

(This explanation fits to lectures for students in their first year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)

[bikespike]

By far one of the best Math channels (and in my opinion recourses in general) out there. Thank you for your efforts. Keep it up

[malawigw]

I will cross my fingers for this video

[Hold_it]

Oooh, now it makes finally sense why the length gives us the area of the Parallelogramm.

I just got told that it works, but i didn't see why it makes sense geometrically. The connection was so random for me.
Thank you!

[andrewxiwu]

As a way to remember this, wouldn't it be nice to point out that component 1 is the determinant of row2+row3? (and similar for other components) :). Of course, this determinant thing will happen again and again in later studies

[thiloherold]

Hi,
As always a brilliant video! But one question arises while watching, why does this only work in R^3? In other words: what makes this space so different from R^2, R^4 or R^42?
Thank you in advance!

[Independent_Man]

Hello, I live in a slightly noisy environment. Can you please increase your mic volume in future videos? I like watching your videos.

[ChrisOffner]

The one thing that every single video on the cross product, including this one, seems to omit, is how to connect the algebraic formula/definition to a geometric intuition, i.e. *why* (in a geometric sense) does this algebraic definition result in a vector that's perpendicular to *u* and *v* and whose length matches the area of the parallelogram?
The same goes for introductions of the determinant: *Why* (in a geometric sense) does the algebraic definition of the determinant of a matrix M give us the volume of the paralellepiped to which which the unit hypercube is mapped by the linear map encoded in M?