Cross products in the light of linear transformations | Chapter 11, Essence of linear algebra

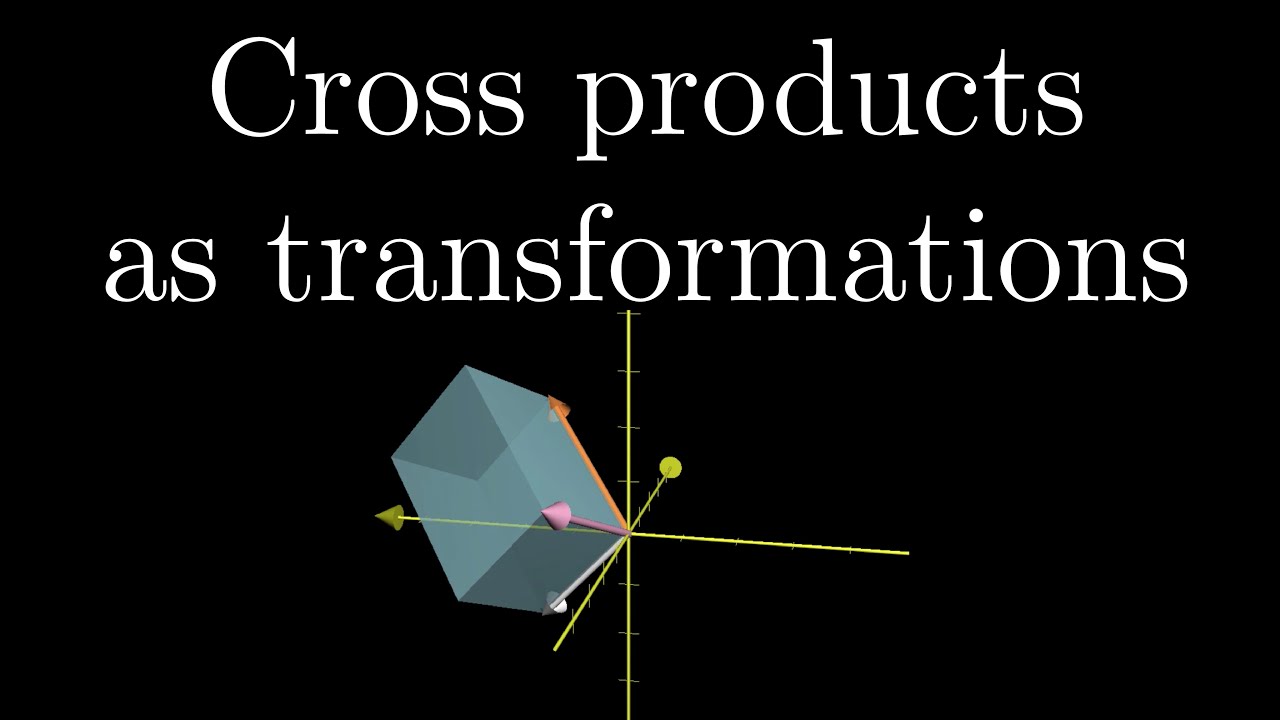
Why the formula for cross products matches the geometric intuition.
An equally valuable form of support is to simply share some of the videos.

For anyone who wants to understand the cross-product more deeply, this video shows how it relates to a certain linear transformation via duality. This perspective gives a very elegant explanation of why the traditional computation of a dot product corresponds to its geometric interpretation.

Minor error at 1:44, the third line of the matrix should read "v1 * w2 - w1 * v2"

*Note, in all the computations here, I list the coordinates of the vectors as columns of a matrix, but many textbooks put them in the rows of a matrix instead. It makes no difference for the result since the determinant is unchanged after a transpose, but given how I've framed most of this series I think it is more intuitive to go with a column-centric approach.

Future series like this are funded by the community, through Patreon, where supporters get early access as the series is being produced.

Thanks to these viewers for their contributions to translations
Hebrew: Omer Tuchfeld
Vietnamese: @ngvutuan2811

------------------

3blue1brown is a channel about animating math, in all senses of the word animate. And you know the drill with YouTube, if you want to stay posted about new videos, subscribe, and click the bell to receive notifications (if you're into that).

Various social media stuffs:

[mohandeshpande]

The next step after watching this video is to watch this video again.

[SkunZielonyJakMech]

I'll try to explain it further (the way I understand the clue of this video).
We define vector p to be such a vector, that for any given vector (x, y, z) dot product of (p) and (x, y, z) is equal to determinant of matrix [x v1 w1 / y v2 w2 / z v3 w3] (let's call this matrix M).
As we have seen in previous videos determinant of a matrix is equal to volume of parallelepiped formed by its column vectors.
From above facts and definitions we know that: Volume of parallelepiped = det(M) = vector (p) (dot) vector (x, y, z)

Now let's look at the volume of parallelepiped more geometrically. It's defined by formula: area of base * height.
We know that area of the base is equal to area of parallelogram of vectors v and w. Now, what is its height? It's the portion of vector (x, y, z) which is perpendicular to the parallelogram! How do we find it?

From previous videos we know that we can find it simply by taking dot product of vector (x, y, z) with unit vector which is perpendicular to parallelogram. Let's call this vector u.

Now our new formula for volume of parallelepiped is: Area of parallelogram * {vector (u) (dot) vector (x, y, z)}

Compare both results: Area of parallelogram * {vector (u) (dot) vector (x, y, z)} = vector (p) (dot) vector (x, y, z)

And you clearly see that: Area of parallelogram * vector (u) = vector (p).

So vector (p) is vector (u) (which is, by definition, UNIT vector perpendicular to parallelogram of v and w) multiplied by *Area of parallelogram*. So vector p is: 1) perpendicular to both v and w 2) has a magnitude = area of parallelogram of v and w.

Amazing result.

[prakhar]

It feels so good to know that there's someone kind enough to put in so much effort and time to make these quality videos(and videos like these are very hard to find) and make them available free to all students around the world.

[taraspokalchuk]

Please tell me where did you learn this from? They do not teach this in uni nd the textbooks are awful. It's scary that my knowledge of linear algebra depends on some good man's will to share this videos.

[ahmedkamal]

I beg you, Please make "Essence of Probability" Series

[ChumX]

Tbh this one is a bit tough to grasp on the first try, but something tells me is gonna feel amazing when I finally get it, thank you so much for sharing! This stuff is gold.

[jamesjin]

If you don't understand it, all I can say is to not give up, because when you finally figure it out, the clarity of understanding and the delayed gratification are simply astounding.
I had to watch the video 2 times and think for at least 30 minutes to finally get it, and I am really glad I persevered.

[gibsonman]

My brain short-circuited somewhere between 0:00 and 13:09. I just woke up. I don't know where I am and I'm scared

[rowangoebel-bain]

You tube is Revolutionizing education. They give us postdocs at Dartmouth who have barely taught before and all I need are these videos. Love the visual clarity

[MikeAuerNixego]

Your series has let me glimpse perhaps a morsel of how theoretical physicists like Einstein see something in the "real world" in terms of its geometric essence, and then use mathematics to describe it. Thank you for that!

[shoumikacharya]

please make a series of "Essence of Complex Analysis"

[kostathomas]

I'm a first year aerospace engineering student who's taking linear algebra and statics. It took me 5 attempts at watching before it finally started to make sense.

I have to think about these things intuitively to know if what I'm doing is correct and I can't imagine learning the same things without having watched this series.

[juustgowithit]

You will probably rewind several times, so:
"what vector p has the special property, that when you take a dot product between the p and some vector [x, y, z] it gives the same result as plugging [x, y, z] to the first column of the matrix whose other two columns have the coordinates of v and w, then computing the determinant?"

[shreyjoshi]

This geometric interpretation of the dot product is brilliant, and when applied to define the computation for the cross product is very insightful. This is literally what I was missing in my HS precal class: reasons for why these methods for computing this stuff works.

On an unrelated note, I would appreciate it if you made an "Essence of Classical Mechanics" series!

[sathirasilva]

You just covered my 6 months course by only 15 videos. HUGE RESPECT! We need more series like these. Thank you sir!

[GTGTRIK]

Okay, so the problem with linear algebra is that people write things in weird ways and then wink at you knowingly.

[BharCode]

This makes more sense in physics than in Math(geometry). Analogy for this cross product is winding/unwinding a screw, where the forces (screwing) applied is in 2D plane, the screw itself gets deeper inside or comes outside into or out of 2d plane, i.e 3D, which is ofcourse perpendicular. It's called Torque. This is also applicable in figuring out magnetic field on a current carrying conductor.
Dot product is for work done (or displacement) given the direction and magnitude of forces applied.
Now go through this video again and as he says "Pause and ponder" that might help.
Thanks for these videos!

[dayliss]

Holy shit. I almost broke down crying about how much sense this makes. Thank you so much!

[ianc]

This is how I understand it:

VOLUME(determinant of 3 3D vectors, i.e. volume of parallelepiped) = BASE (parallelogram of vectors v and w) x HEIGHT(vector [x, y, z] mapped from 3 dimensions onto 1 dimension that is perpendicular to the base)

Hence, the magnitude of the vector P gives the BASE while mapping the vector [x, y, z] to the 1-dimensional direction of P gives the HEIGHT. Thus, the dot product between P and [x, y, z] == VOLUME

Essentially, this means that P serves as a "special 3D vector such that taking the dot product between p and any other vector [x, y, z] gives the same result as plugging x, y, z into the first column of a 3x3 matrix, then computing the determinant" of that 3x3 matrix.

Thank you, Grant, for this beautiful piece of knowledge.

[charlesd]

this is really amazing, the height of the cross product embeds the information of the determinant of V and W (area) and meanwhile the dot product with a variable vector gives us the "height" of the parallelipiped so together they make the volume.



It's also interesting that if we view the cross product as a linear transform, its null space is spanned by V and W. This corresponds to the fact that:
1) for any variable vector that we dot with it, if it has components from the null space, they add 0 to the volume
2) geometrically this means tilting the parallelipied, which doesnt change the volume (like a shear)


today I learned about how the determinant function was derived from 3 constraints of being multilinear, alternating, and normalized. It's really hard to tell where there should be intuition, or "the numbers just work out", or it's just magic


(PS part 1 is reflected in the determinant function, if we nudge the variable vector in the direction of the null space, by linearity of det, we can calculate that volume slice separately, and it is equal to zero because the nudge direction is linearly dependent on V and W, aka a flat additional volume)