filmov
tv
Pre-Algebra 16 - Reducing Fractions
Показать описание
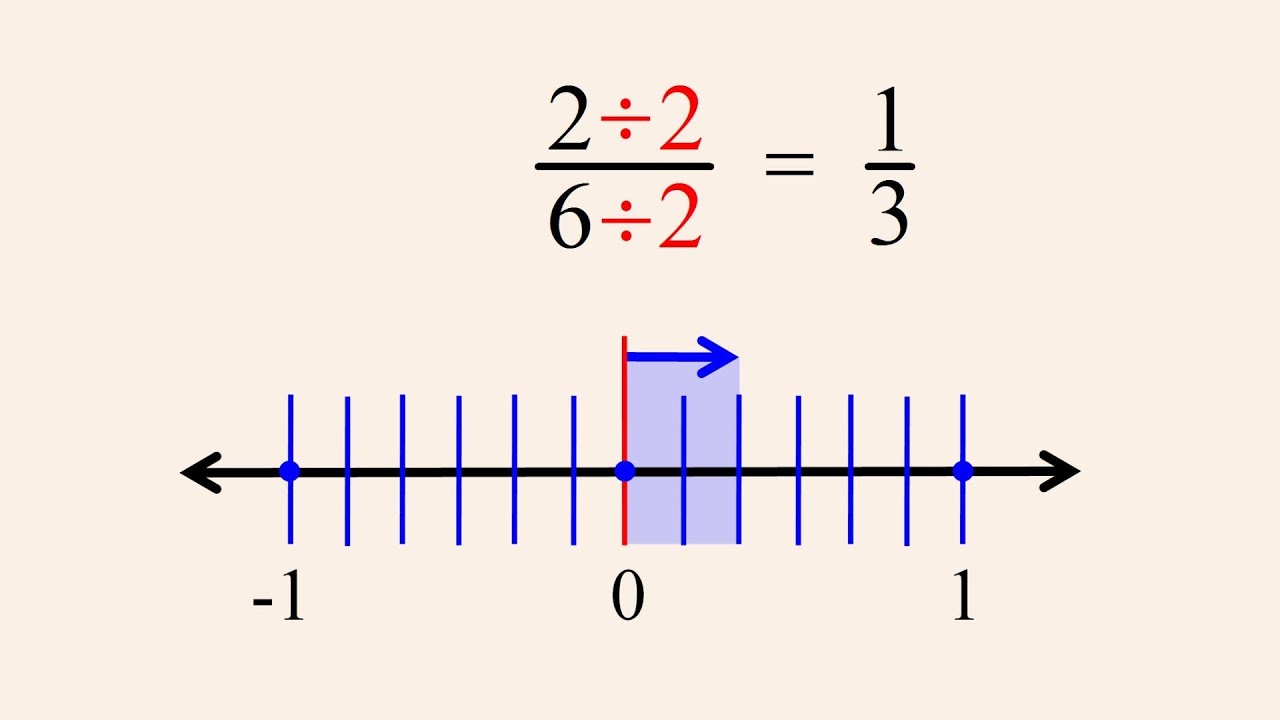
The process of reducing any fraction to its simplest possible form is easily visualized using the number line.
Pre-Algebra 16 - Reducing Fractions
Fractions in lowest terms | Fractions | Pre-Algebra | Khan Academy
ALGEBRA & PRE-ALGEBRA REVIEW: Ch 1 (19 of 53) How to Reduce Fractions
Prealgebra 3.03d - Three Ways to Reduce a Fraction
Prealgebra 3.03c - Three Ways to Reduce a Fraction
ALGEBRA & PRE-ALGEBRA REVIEW: Ch 1 (6 of 53) Equivalent Fractions
Pre-Algebra Lesson #29 Simplifying Fractions | Reducing Fractions to Lowest Terms
Pre-Algebra 17 - Improper Fractions and Mixed Numbers
How to Do Ratios in the Simplest Form in Pre-Algebra : Math Skills
How to Simplify the Fraction 16/38
How to Simplify the Fraction 16/54
Reducing Fractions - Algebra - fwk - TryThis01-0401
How to Simplify the Fraction 16/32
Prealgebra - Part 61 (Writing Fractions in Lowest Terms)
Simplifying Fractions | Three Different Methods to Simplify Fractions | Pre-Algebra
How to Simplify the Fraction 16/18
Saxon Pre Algebra Lesson 14 - Expanding and Reducing Fractions
14 Saxon Pre Algebra, Lesson 014, Expanding and Reducing Fractions
How to simplify fractions to the lowest terms math video
Finding common denominators | Fractions | Pre-Algebra | Khan Academy
Simplifying Fractions
Pre-Algebra 19 - Converting Terminating Decimal Numbers to Fractions
The Hardest Math Test
Fractions & Negative Fractional Exponents | Algebra Properties of Radicals #shorts #maths #math...
Комментарии
 0:05:43
0:05:43
 0:05:42
0:05:42
 0:03:56
0:03:56
 0:06:59
0:06:59
 0:06:38
0:06:38
 0:05:49
0:05:49
 0:19:03
0:19:03
 0:06:49
0:06:49
 0:01:44
0:01:44
 0:01:03
0:01:03
 0:01:17
0:01:17
 0:01:56
0:01:56
 0:01:21
0:01:21
 0:11:04
0:11:04
 0:11:18
0:11:18
 0:01:23
0:01:23
 0:18:19
0:18:19
 0:12:03
0:12:03
 0:03:56
0:03:56
 0:04:42
0:04:42
 0:03:03
0:03:03
 0:03:39
0:03:39
 0:00:28
0:00:28
 0:00:29
0:00:29