filmov
tv
𝑀𝑎𝑠𝑡𝑒𝑟𝑖𝑛𝑔 𝐸𝑥𝑝𝑜𝑛𝑒𝑛𝑡𝑖𝑎𝑙 𝐸𝑞𝑢𝑎𝑡𝑖𝑜𝑛𝑠: 𝑆𝑜𝑙𝑣𝑖𝑛𝑔 8^𝑥 + 2^𝑥 = 130

Показать описание
Welcome back to Prime Math Lovers! Today, we’re diving into the world of exponential equations, specifically: 8x+2x=130
Exponential equations can seem daunting at first, but they’re a crucial part of mathematics, with applications in fields ranging from physics to finance. In this video, we’ll break down the process of solving this equation step by step, ensuring you understand each part of the solution.
We’ll start by discussing what an exponential equation is and how it differs from other types of equations. We’ll then dive into the specific equation we’re solving today. This equation might look intimidating at first glance, but don’t worry - we’ll dissect it piece by piece.
Next, we’ll explore various methods for solving exponential equations. While there are several approaches, we’ll focus on the most efficient method for this particular equation. We’ll walk you through each step of the process, explaining why we’re doing what we’re doing and how each step brings us closer to the solution.
One of the most exciting parts of solving exponential equations is finding the roots. These are the values of x that make the equation true. In this video, we’ll show you how to find these roots and explain what they mean in the context of the equation.
Throughout the video, we’ll also discuss some common pitfalls and challenges you might encounter when solving exponential equations and how to overcome them. Our goal is to not only help you solve this specific equation but also equip you with the skills and knowledge to tackle similar equations in the future.
Remember, understanding complex mathematical concepts like this takes time and practice. Don’t be discouraged if you don’t get it right away. Keep practicing, stay curious, and you’ll get there!
We hope you find this video helpful and informative. If you have any questions or need further clarification on any points, please don’t hesitate to leave a comment. We love hearing from you and are always here to help. And if you enjoyed this video, don’t forget to like, share, and subscribe to Prime Math Lovers for more great math content!
Happy solving!
Exponential equations can seem daunting at first, but they’re a crucial part of mathematics, with applications in fields ranging from physics to finance. In this video, we’ll break down the process of solving this equation step by step, ensuring you understand each part of the solution.
We’ll start by discussing what an exponential equation is and how it differs from other types of equations. We’ll then dive into the specific equation we’re solving today. This equation might look intimidating at first glance, but don’t worry - we’ll dissect it piece by piece.
Next, we’ll explore various methods for solving exponential equations. While there are several approaches, we’ll focus on the most efficient method for this particular equation. We’ll walk you through each step of the process, explaining why we’re doing what we’re doing and how each step brings us closer to the solution.
One of the most exciting parts of solving exponential equations is finding the roots. These are the values of x that make the equation true. In this video, we’ll show you how to find these roots and explain what they mean in the context of the equation.
Throughout the video, we’ll also discuss some common pitfalls and challenges you might encounter when solving exponential equations and how to overcome them. Our goal is to not only help you solve this specific equation but also equip you with the skills and knowledge to tackle similar equations in the future.
Remember, understanding complex mathematical concepts like this takes time and practice. Don’t be discouraged if you don’t get it right away. Keep practicing, stay curious, and you’ll get there!
We hope you find this video helpful and informative. If you have any questions or need further clarification on any points, please don’t hesitate to leave a comment. We love hearing from you and are always here to help. And if you enjoyed this video, don’t forget to like, share, and subscribe to Prime Math Lovers for more great math content!
Happy solving!
 0:09:45
0:09:45
 0:05:58
0:05:58
 0:03:36
0:03:36
 0:10:00
0:10:00
 0:01:42
0:01:42
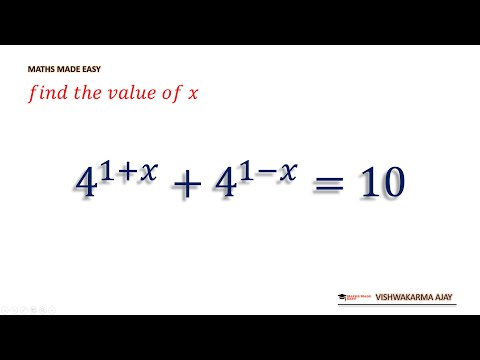 0:05:48
0:05:48
 0:10:19
0:10:19
 0:11:22
0:11:22
 0:09:04
0:09:04
 0:09:15
0:09:15
 0:01:16
0:01:16
 0:12:24
0:12:24
 0:08:57
0:08:57
 0:11:33
0:11:33
 0:08:36
0:08:36
 0:03:17
0:03:17
 0:01:55
0:01:55
 0:07:04
0:07:04
 0:12:22
0:12:22
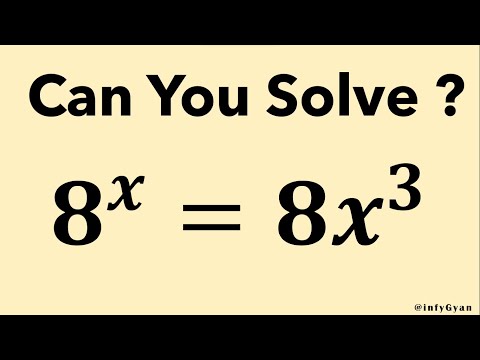 0:11:51
0:11:51
 0:03:35
0:03:35
 0:10:58
0:10:58
 0:08:46
0:08:46
 0:09:42
0:09:42