filmov
tv
Undefined limits by direct substitution | Limits and continuity | AP Calculus AB | Khan Academy

Показать описание
Sal gives an example of a limit where direct substitution ends in a quotient with 0 in the denominator and non-0 in the numerator. Such limits are undefined. What about limits where substitution ends in 0/0? Keep going and you'll see!
AP Calculus AB on Khan Academy: Bill Scott uses Khan Academy to teach AP Calculus at Phillips Academy in Andover, Massachusetts, and heÕs part of the teaching team that helped develop Khan AcademyÕs AP lessons. Phillips Academy was one of the first schools to teach AP nearly 60 years ago.
For free. For everyone. Forever. #YouCanLearnAnything
Undefined limits by direct substitution | Limits and continuity | AP Calculus AB | Khan Academy
Limit of a Function | When direct substitution gives an undefined expression
Limits by direct substitution | Limits and continuity | AP Calculus AB | Khan Academy
Undefined limits by direct substitution
Evaluating Limits By Factoring
Math Differential Calculus - Undefined limits by direct substitution
Calculus: Limits : Finding limit by direct substitution
Finding Limits with Direct Substitution
UNDEFINED LIMIT FUNCTION THAT DOES NOT EXIST IN PRECALCULUS
Evaluating limits using direct substitution
How to Find Limits with Direct Substitution in CALCULUS - Plug in and see!
How to Find Limits by Direct Substitution?
Undefined Limit for Rational Function
Strategies to Solve Limits - Direct Substitution
Learn how to evaluate the limit using direct substitution
Calculus 1 - Introduction to Limits
How to use direct substitution to evaluate the limit
Undefined Limits - 1
Test 1 Limits by Direct Substitution Calculus Lesson 1
Limit Properties - Direct Substitution (example problem)
Undefined limits by direct substitution | Functions | Post Sec Math | KA Urdu
Solving Indeterminate and Undefined Limits Using Algebraic Simplification| Calculus
Evaluate the limit of a rational expression by applying direct substitution
Find the general limit using direct substitution of rational expression
Комментарии
 0:02:13
0:02:13
 0:05:19
0:05:19
 0:02:06
0:02:06
 0:02:23
0:02:23
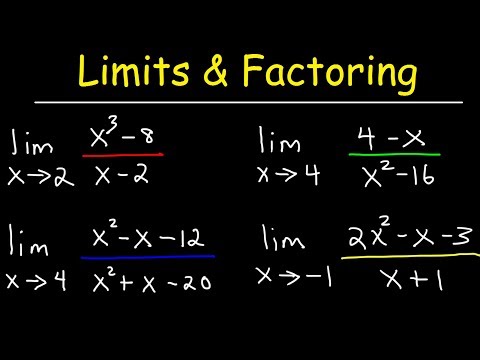 0:11:35
0:11:35
 0:02:14
0:02:14
 0:03:58
0:03:58
 0:07:36
0:07:36
 0:01:32
0:01:32
 0:10:31
0:10:31
 0:05:13
0:05:13
 0:02:10
0:02:10
 0:05:06
0:05:06
 0:04:50
0:04:50
 0:01:38
0:01:38
 0:20:20
0:20:20
 0:01:37
0:01:37
 0:15:53
0:15:53
 0:04:31
0:04:31
 0:01:37
0:01:37
 0:01:33
0:01:33
 0:15:55
0:15:55
 0:01:40
0:01:40
 0:00:24
0:00:24