filmov
tv
Topology Lecture 07: Hausdorff Spaces

Показать описание
We define what it means to be a Hausdorff space. Then we show that in Hausdorff spaces finite sets are closed, limits of convergent sequences are unique, and neighborhoods of limit points intersect the limiting set in infinitely many points.
00:00 Introduction
00:19 Motivation for Hausdorff Property
08:58 Definition: Hausdorff Space
09:35 Example: Metric Spaces
13:00 Example: Discrete Spaces
14:18 Prop: Finite subsets of Hausdorff spaces are closed
19:42 Prop: Limits are unique in Hausdorff spaces
24:50 Prop: Neighborhoods of limit points contain infinitely many points of limiting set
This lecture follows Lee's "Introduction to topological manifolds", chapter 2.
A playlist with all the videos in this series can be found here:
00:00 Introduction
00:19 Motivation for Hausdorff Property
08:58 Definition: Hausdorff Space
09:35 Example: Metric Spaces
13:00 Example: Discrete Spaces
14:18 Prop: Finite subsets of Hausdorff spaces are closed
19:42 Prop: Limits are unique in Hausdorff spaces
24:50 Prop: Neighborhoods of limit points contain infinitely many points of limiting set
This lecture follows Lee's "Introduction to topological manifolds", chapter 2.
A playlist with all the videos in this series can be found here:
Topology Lecture 07: Hausdorff Spaces
Topology ep07: Hausdorff spaces (Feb 1, 2023)
Topology: Lecture 10: Hausdorff Spaces
Intuitive Topology 7: Hausdorff
What is Hausdorff Topological Space?
Hausdorff spaces and the T1 axiom -- Topology video 7
TOPOLOGY Video 28: Hausdorff Spaces - Definition, Examples, Theorem & Problems ( BSc TY Sem 6 )
MAST30026 Lecture 7: Constructing topological spaces (Part 1)
Hausdorff spaces - Properties with proofs | Topology
Topology-Hausdorff Topological Spaces
#7 || Topology | Boundary Points | Dense Set | Hausdorff Space | Continuous Map | Topology Course
Hausdorff topological spaces
Hausdorff Space in a Topological Space - Dr. Sujitha
Homeomorphism and The Hausdorff Property
MAST30026 Lecture 7: Constructing topological spaces (Part 2)
Metric Spaces | Lecture 36 | Hausdorff Property
Hausdorff Spaces
Topology: Hausdorff Spaces
Completely Hausdorff space | Wikipedia audio article
Hausdorff Space
T2 Topological Space#Hausdorff Space#Convergent Sequence in Hausdorff space
Topology Lecture 14 (Lower Separation Axioms)
#80 Topology || Seperation Properties || Definitions || Hausdorff, Regular, Normal Spaces
Limits - Hausdorff spaces and Convergence | Topology
Комментарии
 0:32:01
0:32:01
 0:46:11
0:46:11
 0:28:19
0:28:19
 0:13:52
0:13:52
 0:04:43
0:04:43
 0:29:44
0:29:44
 0:18:47
0:18:47
 0:49:48
0:49:48
 0:14:26
0:14:26
 0:25:36
0:25:36
 1:20:21
1:20:21
 0:16:05
0:16:05
 0:05:08
0:05:08
 0:07:11
0:07:11
 0:47:29
0:47:29
 0:08:38
0:08:38
 0:10:36
0:10:36
 0:12:45
0:12:45
 0:04:29
0:04:29
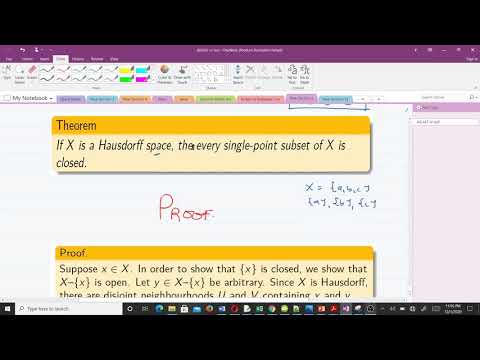 0:09:56
0:09:56
 0:12:39
0:12:39
 0:31:55
0:31:55
 0:17:37
0:17:37
 0:08:23
0:08:23