Applications of triple integrals, Multivariable Calculus Unit 4 Lecture 6

We look at three applications for integrating functions of the form w=f(x,y,z) over regions in the (x,y,z)-plane: (1) volume computations, (2) average value computations, and (3) center of mass computations. This is Multivariable Calculus Unit 4 Lecture 6.

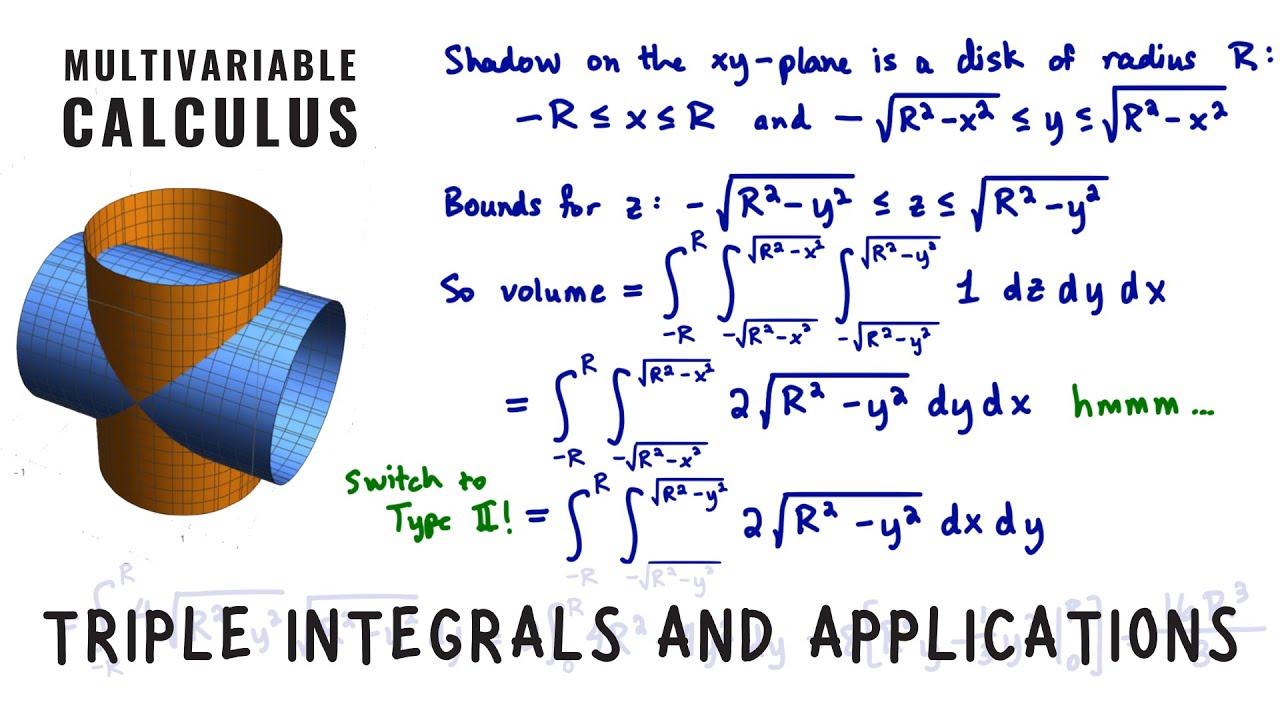
We begin by connecting the concept of triple integrals to the volume computations similar to area computations in double integrals. If we have a closed bounded region 𝐷 in 𝑥𝑦𝑧-space that can be described with inequalities, we can set up the triple integral of one over 𝐷 to compute its volume. We denote 𝑑𝑉 as the differential volume element, which is a shorthand for 𝑑𝑥𝑑𝑦𝑑𝑧, or any permutation of these differentials depending on the region type.

Volume of 𝐷=∭_𝐷 1 𝑑𝑉

We then discuss how to compute the average value of a scalar-valued function 𝑓 of three variables over a domain 𝐷. The average value is the triple integral of 𝑓 over 𝐷 divided by the volume of 𝐷. This can be re-written as a ratio of integrals:

Average value of 𝑓 = ∭_𝐷 𝑓(𝑥,𝑦,𝑧) 𝑑𝑉 /∭_𝐷 1 𝑑𝑉.

The final application we cover is computing the center of mass for a non-uniform three-dimensional object. The center of mass is the point (X,Y,Z) where we can balance the object. We find it by dividing the moments by the total mass 𝑀:

(X,Y,Z) = (𝑀𝑦𝑧/𝑀, 𝑀𝑥𝑧/𝑀, 𝑀𝑥𝑦/𝑀).

#calculus #multivariablecalculus #mathematics #tripleintegral #integration #iitjammathematics #calculus3 #averagevalue #centerofmass

[dhruv]

how to write matlab code for this intersection