filmov
tv
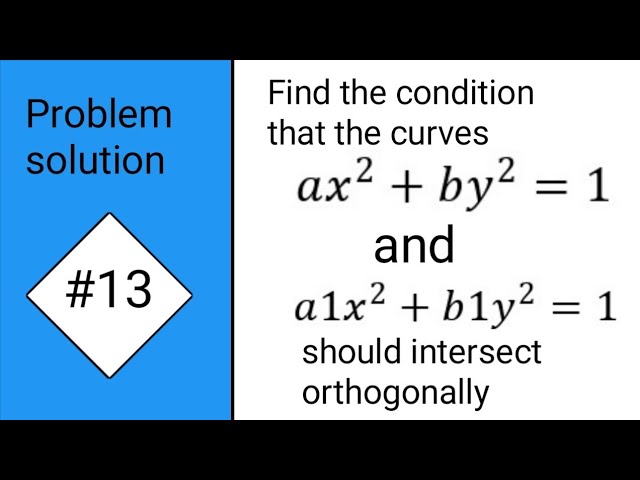
Find condition that the curves ax^2+by^2=1 and a1x^2+b1y^2=1intersect orthogonally
Показать описание
Find condition that the curves ax^2+by^2=1 and a1x^2+b1y^2=1intersect orthogonally
Find the condition that the curves intersect orthogonally
To find the condition for the curves \(ax^2 + by^2 = 1\) and \(a_1x^2 + b_1y^2 = 1\) to intersect orthogonally, we can use the fact that the product of the slopes of two curves at their point of intersection should be -1.
Chapter plane curve::
The general form of a curve is \(y = f(x)\), and its slope is given by \(f'(x)\). For the curves \(ax^2 + by^2 = 1\) and \(a_1x^2 + b_1y^2 = 1\), we need to find their derivatives with respect to \(x\), and then set the product of their slopes equal to -1.
This leads to the condition:
\[2ax + 2b\frac{dy}{dx} \cdot \frac{-1}{2b} + 2a_1x + 2b_1\frac{dy}{dx} \cdot \frac{-1}{2b_1} = 0\]
Hashtags
#orthogonal
#curve
#conicsection
#orthogonal
#analyticgeometry
#mathsolutions
#mathematics
#education
Keywords:::
OrthogonalCurves,
CurveIntersection,
ConicSections,
OrthogonalCondition,
AnalyticGeometry,
Equations,
Question 13,
IntersectionPoints,
OrthogonalTangents,
GeometricConditions,
find the condition that the zeros of
the polynomial x3-px2+qx-r are in ap,
find the condition that one root of the
equation is twice the other,
find the condition that the roots of
the equation are in hp,
find the condition that zeros of
polynomial x square + bx +care
reciprocal of each other,
find the condition that the roots of
the equation are in gp,
find the condition that the line
y=mx+c may touch the parabola
y2=4ax
find the condition that the roots of
the equation are in ap,
find the condition that the zeros of
the polynomial x3-px2+qx-r are in gp
Find the condition that the curves intersect orthogonally
To find the condition for the curves \(ax^2 + by^2 = 1\) and \(a_1x^2 + b_1y^2 = 1\) to intersect orthogonally, we can use the fact that the product of the slopes of two curves at their point of intersection should be -1.
Chapter plane curve::
The general form of a curve is \(y = f(x)\), and its slope is given by \(f'(x)\). For the curves \(ax^2 + by^2 = 1\) and \(a_1x^2 + b_1y^2 = 1\), we need to find their derivatives with respect to \(x\), and then set the product of their slopes equal to -1.
This leads to the condition:
\[2ax + 2b\frac{dy}{dx} \cdot \frac{-1}{2b} + 2a_1x + 2b_1\frac{dy}{dx} \cdot \frac{-1}{2b_1} = 0\]
Hashtags
#orthogonal
#curve
#conicsection
#orthogonal
#analyticgeometry
#mathsolutions
#mathematics
#education
Keywords:::
OrthogonalCurves,
CurveIntersection,
ConicSections,
OrthogonalCondition,
AnalyticGeometry,
Equations,
Question 13,
IntersectionPoints,
OrthogonalTangents,
GeometricConditions,
find the condition that the zeros of
the polynomial x3-px2+qx-r are in ap,
find the condition that one root of the
equation is twice the other,
find the condition that the roots of
the equation are in hp,
find the condition that zeros of
polynomial x square + bx +care
reciprocal of each other,
find the condition that the roots of
the equation are in gp,
find the condition that the line
y=mx+c may touch the parabola
y2=4ax
find the condition that the roots of
the equation are in ap,
find the condition that the zeros of
the polynomial x3-px2+qx-r are in gp
 0:05:04
0:05:04
 0:15:41
0:15:41
 0:04:58
0:04:58
 0:10:20
0:10:20
 0:18:28
0:18:28
 0:08:23
0:08:23
 0:11:06
0:11:06
 0:26:31
0:26:31
 0:10:52
0:10:52
 0:19:15
0:19:15
 0:00:17
0:00:17
 0:00:21
0:00:21
 0:00:33
0:00:33
 0:39:43
0:39:43
 0:07:23
0:07:23
 0:10:04
0:10:04
 0:02:19
0:02:19
 0:07:40
0:07:40
 0:05:56
0:05:56
 0:21:45
0:21:45
 0:09:30
0:09:30
 0:15:05
0:15:05
 0:11:24
0:11:24
 0:24:18
0:24:18