filmov
tv
Solving all the integrals from the 2023 MIT integration bee finals

Показать описание
Sit back, relax and enjoy the wild ride of evaluating the beastly integrals from the 2023 finals.
Thank you Myers for the wonderful solution development for problem 2.
Thank you Poro :D for the timestamps:
Q1 : 0:11
Q2 : 4:49
Q3 : 12:26
Q4 : 21:24
Thank you Myers for the wonderful solution development for problem 2.
Thank you Poro :D for the timestamps:
Q1 : 0:11
Q2 : 4:49
Q3 : 12:26
Q4 : 21:24
Solving all the integrals from the 2023 MIT integration bee finals
how to solve these HARD integrals
How REAL Men Integrate Functions
Integration (Calculus)
life changing integration by parts trick
Integration Tricks (That Teachers Won't Tell You) for Integral Calculus
100 integrals (world record?)
Integration and the fundamental theorem of calculus | Chapter 8, Essence of calculus
How to Solve Double Integrals in Polar Coordinates - Example 1
What Integration Technique Should I Use? (trig sub, u sub, DI method, partial fractions) calculus 2
Find The Integral – How To Do Basic Calculus Integration
Math Integration Timelapse | Real-life Application of Calculus #math #maths #justicethetutor
Feynman's technique is the greatest integration method of all time
It 'Cannot' Be Done (Integrals)
Solving 'impossible' integrals in seconds
Feynman's Technique: This is the greatest integration method of All Time
Calculus - Definite Integrals
Trick for Memorizing Trig Integrals
Basic Integration Problems
Calculus 1 - Integration & Antiderivatives
integration by parts, DI method, VERY EASY
How to solve double integrals (steps)
❖INTEGRATION All Formulas Quick Revision For Class 12th Maths with Tricks and Basics NCERT SOLUTIONS...
GCE 2018/2019 Paper 2 - Integration Calculus
Комментарии
 0:39:30
0:39:30
 0:09:45
0:09:45
 0:00:35
0:00:35
 0:07:04
0:07:04
 0:05:23
0:05:23
 0:11:26
0:11:26
 5:50:23
5:50:23
 0:20:46
0:20:46
 0:24:32
0:24:32
 0:22:40
0:22:40
 0:11:08
0:11:08
 0:00:09
0:00:09
 0:12:13
0:12:13
 0:03:11
0:03:11
 0:06:35
0:06:35
 0:01:07
0:01:07
 0:07:15
0:07:15
 0:07:17
0:07:17
 0:14:13
0:14:13
 0:40:04
0:40:04
 0:16:59
0:16:59
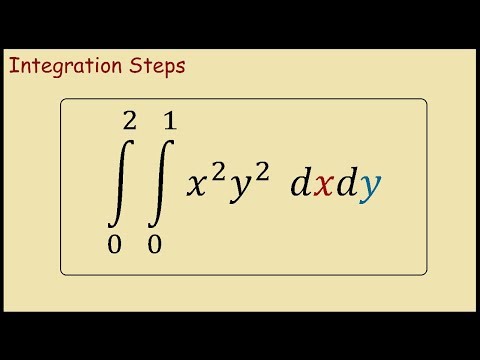 0:02:22
0:02:22
 0:26:43
0:26:43
 0:10:25
0:10:25