filmov
tv
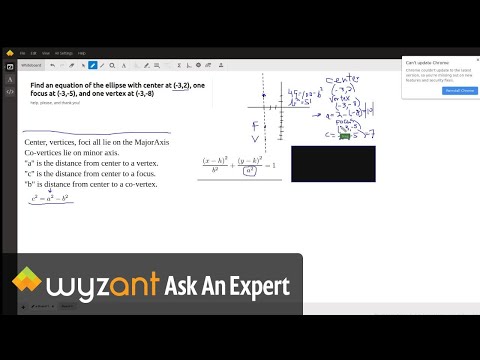
Given the center, vertex and co vertex, write the equation of the ellipse

Показать описание
Learn how to write the equation of an ellipse from its properties. The equation of an ellipse comprises of three major properties of the ellipse: the major radius (a), the minor radius (b) and the center (h, k). The ellipse is vertical if the major radius in vertical and the ellipse is horizontal if the major radius is horizontal.
The center of an ellipse is halfway between the major/minor axis. When given the vertices of an ellipse, the major radius of an ellipse is the distance between the center of the ellipse and its vertices. When given the co-vertices of an ellipse, the minor radius of an ellipse is the distance between the center of the ellipse and its co-vertices.
After obtaining the center, the major and the minor radius, they are plugged into the equation of an ellipse to obtain the desired equation.
Follow us on
#conicsections #ellipseconicsections
The center of an ellipse is halfway between the major/minor axis. When given the vertices of an ellipse, the major radius of an ellipse is the distance between the center of the ellipse and its vertices. When given the co-vertices of an ellipse, the minor radius of an ellipse is the distance between the center of the ellipse and its co-vertices.
After obtaining the center, the major and the minor radius, they are plugged into the equation of an ellipse to obtain the desired equation.
Follow us on
#conicsections #ellipseconicsections
 0:04:27
0:04:27
 0:03:36
0:03:36
 0:03:31
0:03:31
 0:03:45
0:03:45
 0:02:59
0:02:59
 0:04:46
0:04:46
 0:06:46
0:06:46
 0:04:49
0:04:49
 0:03:35
0:03:35
 0:03:16
0:03:16
 0:04:25
0:04:25
 0:03:58
0:03:58
 0:09:05
0:09:05
 0:04:51
0:04:51
 0:02:37
0:02:37
 0:06:51
0:06:51
 0:07:15
0:07:15
 0:10:52
0:10:52
 0:05:23
0:05:23
 0:06:53
0:06:53
 0:04:44
0:04:44
 0:04:45
0:04:45
![[PreCal] Writing Standard](https://i.ytimg.com/vi/ky1yAzhpqF8/hqdefault.jpg) 0:03:06
0:03:06
 0:08:06
0:08:06