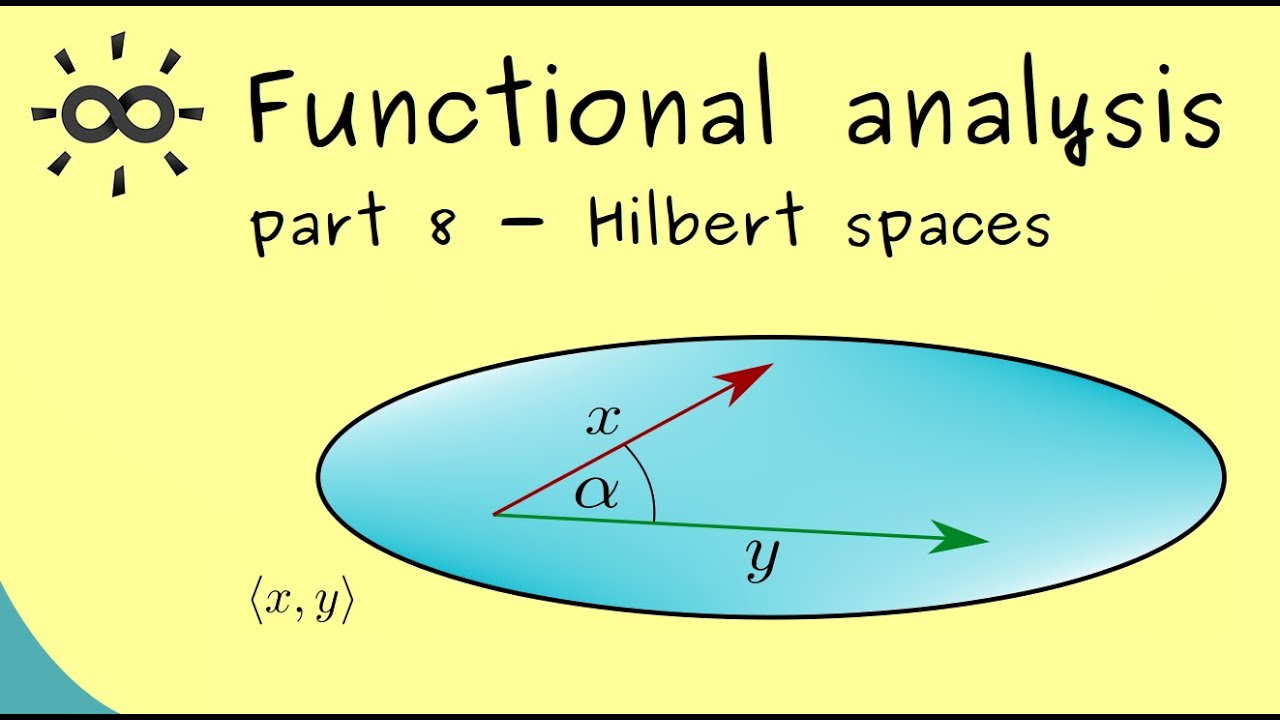
Functional Analysis 8 | Inner Products and Hilbert Spaces

Please consider to support me if this video was helpful such that I can continue to produce them :)

🙏 Thanks to all supporters! They are mentioned in the credits of the video :)

This is my video series about Functional Analysis where we start with metric spaces, talk about operators and spectral theory, and end with the famous Spectral Theorem. I hope that it will help everyone who wants to learn about it.

x

00:00 Introduction
02:25 Inner product definition
06:25 Norm and Hilbert space

#FunctionalAnalysis
#VectorSpaces
#Mathematics
#LearnMath
#calculus

I hope that this helps students, pupils and others. Have fun!

(This explanation fits to lectures for students in their first and second year of study: Mathematics for physicists, Mathematics for the natural science, Mathematics for engineers and so on)

[PunmasterSTP]

Man I really appreciate how that first slide broke down the differences in such a clear and simple way! Thanks again so much for making and sharing all of these wonderful videos.

[johnitaballmer]

Fantastic ability to explain abstract ideas! Thank you so much.

[HajjadyMathsClass]

this is a very concise and perfect definition

[amirkia]

Hi, thank you very much.
I have a question; at 6:58 you said that we can define a special norm based on inner product, and norm must satisfy three properties, I have no problem with first two properties, but about 3rd one, can't obtained it.
||x+y|| =< ||x|| + ||y||
for the left side,
||x+y|| = sqrt(<x+y, x+y>) =
sqrt(<x, x> + <x, y> + <y, x> + <y, y>).
now the right side,
sqrt(<x, x>) + sqrt(<y, y>).
I don't know how to continue!

[xwyl]

So, this is Hilbert space. I remember back in university I saw a super thick book with 'Hilbert space' on its hardcover. It was at that moment Hilbert space casted huge shadow over my soul. Now it doesn't seem to be very terrifying... at the first look.

[acgogle]

hey thanks a lot for these videos. I am currently taking a functional analysis lecture and it is awesome to hear the fundamental definitions explained by someone else :) weiter so!!

[MrWater]

A really brief recap of trigonometry would be useful when you defined the inner product using cos(\alpha). Some time ago I was trying to read Time Series Theory and Methods from Blackwell and Davis, they have a chapter (2) about Hilbert spaces. I wish I would have your videos in that moment! Probably I'm going to try to read again that book when I finish this series. Thanks again!

[saptarshisahoo]

Could you recommend a book for functional analysis?

[ifyhu]

I always give thumbs up to of your videos love you so much 😂❤❤❤

[Bangalidude]

Best video on inner space I've seen till now 🔥🔥

[sarwagyaprasad]

I had a question, is norm and inner product defined only for vector spaces over real or complex field? Is it not definable for any vector space over an arbitrary field, for ex. over Q?

What I'm guessing is that there are some problems that come in defining it for such cases, either losing consistency or usefulness. If yes, what are these problems?

[opokufrederick]

Please I would like you to make video on pseudo differential operators. I am now understanding the mathematics I did in my undergrad

[ferry]

Is linearity defined in second argument is common? because I try reading from some materials all says it defined linearity on first argument

[yuxinzhang]

4:42 I feel confused about why we need a complex conjugate, could anyone give me a further explanation?

[fernandolimoeirolaradeoliv]

Nice explaination, but you could've extended a little more on Hilbert Spaces and throw some examples!! I'm recomminding this video to lots of friends! =)

[luqmankhan]

Hello sir which software u will use for class

[SiriusFuenmayor]

There is a video also for topological spaces?

[LogicRk]

semi-inner product having all those properties except <X.X> =0 =>X=0

[sahhaf]

@7:13 I am not sure on this but I think one cannot measure angles in a hilbert space. Angles can only be measured if the hilbert space is real. Complex hilbert spaces do not have the concept of angles...

[StratosFair]

What's that about linearity in the second component only ? Shouldn't it be linear in both its arguments anyway ? And I'm also not 100% clear on why we need the conjugate when we're dealing with complex numbers, anyone got an example ?