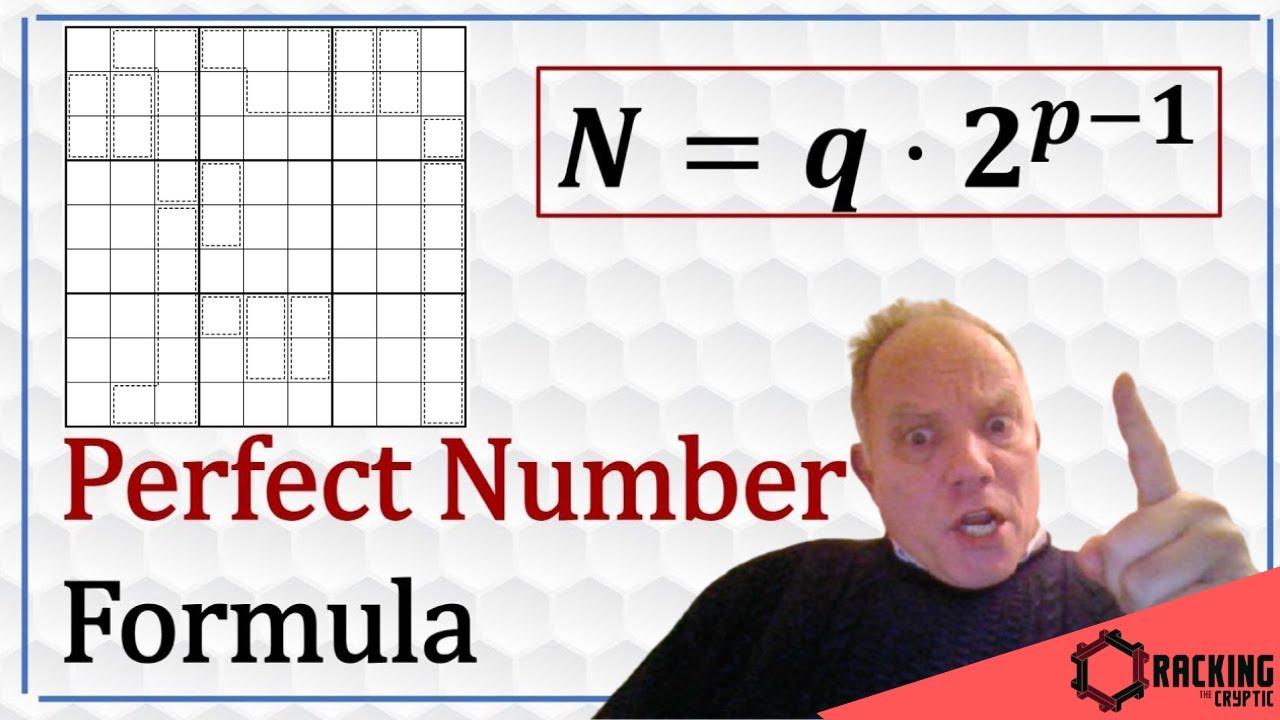
The Perfect Sudoku?

Normal sudoku rules apply. Orthogonally adjacent cells do not contain consecutive digits. Digits in a cage do not repeat and sum to a perfect number. A perfect number is a number that equals the sum of its proper divisors (i.e. each divisor excluding the number itself). For example, 20 is not a perfect number since the sum of the proper divisors is 1+2+4+5+10=22, which is not 20. [Hint: There are only two perfect numbers that are less than 45]

▶ OUR PATREON PAGE – JOIN THE COMMUNITY, SUPPORT US AND TRY OUR PUZZLE HUNTS◀
October’s Patreon reward is a treat (no tricks!) – Caroline’s Halloween Adventure by the PBN Institute! It’s just $2/month for the monthly reward, $3/month or higher for solution videos. Lots of other great content available, including crosswords, other sudoku solves, and Rockratzero’s excellent “600K Bang!” There is also a patreon-only channel on our discord for help with the puzzles. We appreciate your support!

▶ ALL OUR APPS ◀

▶NEWEST: Cracking the Cryptic’s Approachable Sudoku! ◀

▶ 600k Celebration pack ◀ You can get this collection on our Patreon site (link above) absolutely free of charge. Thank you very much for joining us in this wonderful community of setters and solvers. Watch the live stream(s) to see us solve some of these!

▶ HOLIDAY SHOPPING? Check out our books and merchandise, perfect gifts for the sudoku-lover in your life (or for yourself)!! ◀

▶ SUDOKU PAD ◀ Input classic sudoku puzzles into our software. With 12 free puzzles:
Amazon: Search for “SudokuPad”

▶ CTC FAN DISCORD SERVER◀

▶ OUR VIDEO CATALOGUE – CATEGORISED WITH LINKS◀

▶SEND US PUZZLES TO SOLVE/CONTACT US◀
Snailmail: Mark Goodliffe, Box 102, 56 Gloucester Road, London SW7 4UB

▶FOLLOW US◀
Twitter: #crypticcracking
@crypticcracking
Instagram @crackingthecryptic

▶Credits◀
Music: Tim McCaskey (Guitar) or Lucy Audrin (Piano): Mozart's Sonata no 16; and Mark’s outro music by Riffclown.
SOFTWARE: Thanks to Sam Cappleman-Lynes and Sven Neumann
Logo: Melvyn Mainini
Opening Credits: Joel Blundell

▶ABOUT US◀
Hi! We're Simon Anthony and Mark Goodliffe, two of the UK's most enthusiastic puzzle solvers. We have both represented the UK at the World Sudoku Championships and the World Puzzle Championships. We're also "cryptic crossword" aficionados. Mark is the twelve-time winner of The Times crossword championship and twice Senior World Champion in Sudoku, and Simon is the former record holder for most consecutive correct solutions to The Listener crossword. We hope we can help your puzzle solving while also introducing you to some of the world's best puzzles.

Thank you for watching!
Simon and Mark
#solvingsudoku #logicpuzzles
▶ Contents ◀
0:00 Theme Music & News around the channel
2:54 Rules of today’s puzzle
4:35 Start Of Solve - Let's Get Cracking!

[Lithium-Ion-]

Hello, Lithium-Ion here. Many thanks for the feature. Did you know that it is an open mathematical problem whether an odd perfect number exists? As Mark said, even perfect numbers are very rare with 496, 8128, 33550336 and 8589869056 being the next larger perfect numbers. I hope that you enjoy this puzzle and wish you a perfect day!

[eddieharwood]

I thought that was fantastic. I had to really look hard at it and struggled to find the next step 3 times but I always persevered and eventually triumphed in 3 times Mark's time, which I have not looked at.

[chocolateboy]

I finished in 38:46 minutes. I really dig these puzzles that have a hidden start like this one does. I did another puzzle earlier, which had the clues hidden in an unknown base by aliens. This puzzle reminded me of that. It's such a cool way to start a puzzle. Although, I did spend about 9 minutes working through every number. I didn't want to look it up, because I felt like the puzzle didn't want me to. It did give me a hint with their being only two below 45, so I thought I should respect it and manually determine both numbers. The puzzle itself was really enjoyable. I liked how a hidden 28 cage formed in column 2. My favorite part had to be calculating the possibilities of the empty space of box 2. I was able to get two possibilities, a 1439 and a 2357. I was wondering how they got resolved, then I remembered the anti-consecutive rule. The puzzle became much easier after that. I enjoyed this on every much. Great Puzzle!

[BarryKort]

There did turn out to be roping in all directions, and once there is some roping at hand, that speeds up filling in the candidates for the other sides of the roping pattern.

[MattYDdraig]

23:21
Some lovely work to use the non-consecutive rule make everything work out with only the cage contents identified. The roping helped a lot too.

[emilywilliams]

I have come to love the non-consecutive rule. It took me a while to make friends with it, but as you said, Mark, it does a lot of work once the puzzle is a bit underway. The Miracle Sudoku app is fantastic, one of my favorites. Thanks for the video!

[AcidDotCom]

What a perfect way to finish a day...a CTC video <3

[awilliams]

"I wonder if I'm missing something major" - mark with his mouse circling around R9C4 with a "3/9" candidate just below an "8/9" candidate.
9 can never go next to

[nfc]

It took me a long time to realise how the constructor had managed the orthogonal rule - I could have saved a lot of time if I'd thought about that earlier.

[Coyotek]

30:09 ... I was a bit slow on seeing things, but I'm glad I saw a pleasing pathway through

Perfect puzzle!

[Superalex]

The third perfect number is 496
The only thing you missed was in box 7, due to the rule square 6 in box 7, could be a 9, due to the 8 next to it.

[Jodawo]

Mathematicians think there is an infinite amount of perfect numbers. In fact, there is a formula for a perfect numbers that uses prime numbers. Each time they find a new record high prime number, the world record for the highest perfect number is found.

[eefaaf]

28:41 'And that's now allowed to be a 4 by the rule as well'... nor can it be a 9 next to the 8 leaving a 1.

[FrancisFjordCupola]

32:37 "It's probably something much more obvious..." What? Like the 19-pair in r8c3 next to the 8 that could get rid of the nine? Funny thing is Mark even wrote down 149 in r8c3 and noting that the 4 could not be next to the 5 below. Then again, spotting the obvious is easy when you're not distracted by the big picture.

[davidh.]

1:07:54@#1102. Very difficult, and I could only proceed with liberal use of the cage calculator, and a lazy look-up of perfect numbers. It took forever to find the break-in. But once I got some digits in the non-consecutive rule tore the rest down quickly. Definitely not my best, but oddly better than my performance on Simon's today.

Edit1: I am proud to have recognized the limit on the outie total in columns 2/3.
Edit2: My final break-in move was realizing that placing 6 in R2C3 would lead to an impossible 789 in box 2. That's what opened the rest of it up.

[scarletmanuka]

18:16 "If that's a 9, it's not so difficult in the row" - actually it breaks the row quite easily; the other two in the box are 67 and 678, so neither can be 7 and there's no 7 in the row.

[Edos]

the approach i followed for R2C4 was...

R3C1 and R3C2 would be either 14 or 25 in R3C4-6 the other pair would be accompanied by either 3 or 8

therefore the value of R2C4 would be (R2C4+R3C4-6 = 17)

(14 3) ->9
(25 3) ->7
(14 8) -> 4 X
(25 8) -> 2 X (these 2 are not valid as they repeat numbers)

So we can deduce that 8 CANNOT be in R3C4-6 and it goes in R3C3 and R2C4 is 7 or 9

[inspiringsand]

Let's Get Cracking: 00:35
Mark's time: 35m27s
Puzzle Solved: 36:02

What about this video's Top Tier Simarkisms?!
Three In the Corner: 1x (24:33)
The Secret: 1x (08:30)

And how about this video's Simarkisms?!
By Sudoku: 4x (28:04, 29:45, 32:38, 34:36)
Ah: 4x (10:03, 11:00, 13:18, 21:08)
In Fact: 3x (15:18, 25:54, 35:41)
Sorry: 2x (10:06, 32:59)
Goodness: 1x (10:28)
Clever: 1x (36:07)
Missing Something: 1x (18:38)
Naughty: 1x (24:29)
Lovely: 1x (35:59)
Extraordinary: 1x (26:46)
Come on Mark: 1x (19:35)
Bonkers: 1x (23:03)
Hang On: 1x (17:23)
Progress: 1x (34:12)
Wow: 1x (23:51)
Pencil Mark/mark: 1x (14:53)
Weird: 1x (32:21)

Most popular number(>9), digit and colour this video:
Twenty Eight (16 mentions)
Two (64 mentions)
Yellow (5 mentions)

Antithesis Battles:
Low (2) - High (0)
Even (2) - Odd (0)
Row (14) - Column (13)

FAQ:
Q1: You missed something!
A1: That could very well be the case! Human speech can be hard to understand for computers like me! Point out the ones that I missed and maybe I'll learn!
Q2: Can you do this for another channel?
A2: I've been thinking about that and wrote some code to make that possible. Let me know which channel you think would be a good fit!

[SpyrosSolda]

I'm sure there is a numberphile video about perfect numbers and no upper boundary is found yet

[littlston]

28.6 is going to be known as perfect day from now on