filmov
tv
Riemann Roch: genus 3 curves

Показать описание
This talk is about the Riemann-Roch theorem for genus 3 curves. We show that any such curve is either hyperelliptic or a nonsingular plane quartic. We find the Weierstrass points and the holomorphic 1-forms and the canonical divisors of these curves. Finally we give a brief description of the moduli space of genus 3 curves.
Riemann Roch: genus 3 curves
Applications of Riemann-Roch to Classifying Low genus curves
Riemann Roch: genus 2 curves
Riemann Roch: plane curves
Riemann Roch App
Example: Hirzebruch-Riemann-Roch for Curves
Riemann Roch Spaces
Selective Integration on Adelic Spaces and the Riemann-Roch theorem, W. Czerniawska (UNIGE)
Riemann Roch for genus 0 curves
Riemann-Roch
[ISS, Fall 2022] Lecture 4 - Riemann-Roch Theorem
Riemann Roch: structure of genus 1 curves
Divisor class group arithmetic on non-hyperelliptic genus 3 curves
14.2 The statement of the Riemann-Roch Theorem (Commutative Algebra and Algebraic Geometry)
Example: Hirzebruch-Riemann-Roch for threefolds
Analytic Grothendieck Riemann Roch Theorem || Prof. Xiang Tang || WOTOA
Elliptic Curves - Lecture 7 - Riemann-Roch, Hurwitz, and Weierstrass equations
Riemann Roch: Proof, part 1
Rewrite Riemann Roch with Serre Duality
Riemann Roch if space is a single point
Riemann Roch (Introduction)
The Riemann Hurwitz formula.
Philipp Habegger: The Number of Rational Points on a Curve of Genus at Least Two
Riemann Roch: genus 1
Комментарии
 0:30:51
0:30:51
 0:25:09
0:25:09
 0:30:03
0:30:03
 0:23:19
0:23:19
 0:16:57
0:16:57
 0:05:57
0:05:57
 0:10:21
0:10:21
 0:27:50
0:27:50
 0:21:14
0:21:14
 0:03:56
0:03:56
![[ISS, Fall 2022]](https://i.ytimg.com/vi/AuxvP6eQ-1g/hqdefault.jpg) 1:04:39
1:04:39
 0:18:05
0:18:05
 0:17:44
0:17:44
 0:16:09
0:16:09
 0:15:02
0:15:02
 1:00:47
1:00:47
 1:14:04
1:14:04
 0:29:48
0:29:48
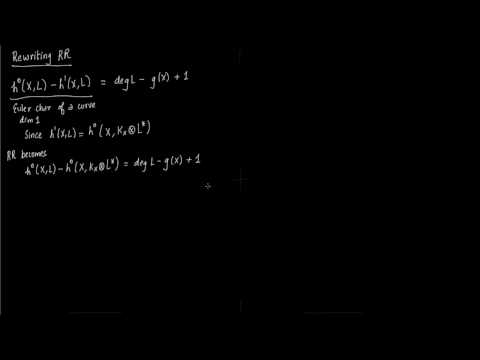 0:14:28
0:14:28
 0:17:45
0:17:45
 0:27:38
0:27:38
 0:33:13
0:33:13
 0:45:15
0:45:15
 0:31:37
0:31:37