filmov
tv
Integral of ln(sinx) from 0 to pi/3
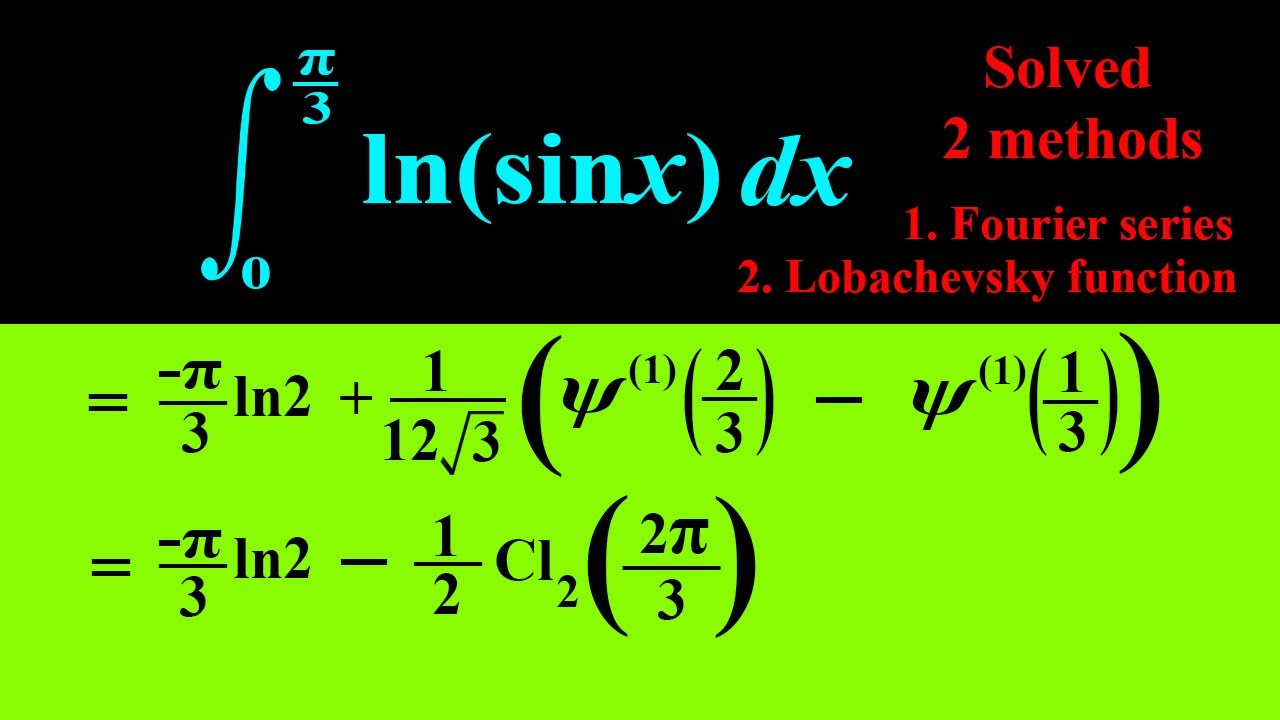
Показать описание
Integral of ln(sinx) from 0 to pi/3, is a little bit hard integral to integrate, however In this video I evaluated it by using 2 different method. First I used Fourier series for ln(sinx) and second time I used Lobachevski function.
In previous videos I evaluate Integral ln(sinx) from 0 to pi/2 and integral ln(sinx) from 0 to pi/4 so, I thought to create a content for integrate Integral of ln(sinx) from 0 to pi/3.
Integration of lnsinx from 0 to pi/3 | Integration | ln(sinx) integral | Integrals | Hard Integral
#Integration #lnSinx #Math
In previous videos I evaluate Integral ln(sinx) from 0 to pi/2 and integral ln(sinx) from 0 to pi/4 so, I thought to create a content for integrate Integral of ln(sinx) from 0 to pi/3.
Integration of lnsinx from 0 to pi/3 | Integration | ln(sinx) integral | Integrals | Hard Integral
#Integration #lnSinx #Math
integration of ln(sinx) from 0 to phi/2
Integral of ln(sinx) from 0 to pi/4
Integral of ln(sinx) from 0 to pi/3
The Most Useful Integration Formula : ∫ ln(sinx)dx
Improper Integral of ln(sinx) from 0 to pi/2: MIT Integration Bee (4)
Integral of ln(sinx) from 0 to pi/2
integral ln sinx from 0 to pi/2 | An interesting integral
Integral ln(sin x) from 0 to pi/2
Integral from 0 to pi/2 of (ln(cosx)ln(sinx))/(tanx)
Integral of ln(sinx) from 0 to pi/2 | #Shorts
Integral of ln(sin x)
2. (Calculus) Definite Integral of f(x)=ln(sinx) from 0 to pi/2 (important example)
Integral ln(sinx)ln(cosx)/tanx from 0 to pi/2
Integrate ln(sinx) or ln(cosx) from 0 to pai /2 | Definite Integral class-12 | Jee | Jee main
How to integrate cotx ln(sinx )dx
Video 2034.23 - Integrate sinx ln(sinx)
Derivative of ln[sinx], 20 seconds
Integral of ln(x) fast!
Consider is `int_(0)^(pi//2) ln (sinx)dx` equal to ? What is `int_(0)^(pi//2)
Calculus Derivative of Functions with Natural Log ln (sinx)
How REAL Men Integrate Functions
Integration of ln(sinx)
Consider is `int_(0)^(pi//2) ln (sinx)dx` equal to ? What is `int_(0)^(pi//2)ln (sinx) dx`
integration of ln(sinx) when upper limit is pi/2 and lower limit is 0
Комментарии
 0:04:25
0:04:25
 0:17:33
0:17:33
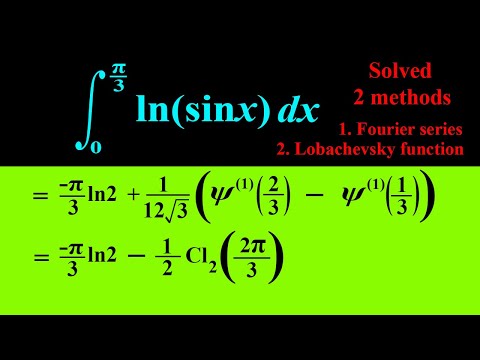 0:15:39
0:15:39
 0:07:01
0:07:01
 0:13:24
0:13:24
 0:00:31
0:00:31
 0:08:32
0:08:32
 0:13:37
0:13:37
 0:06:55
0:06:55
 0:00:55
0:00:55
 0:09:52
0:09:52
 0:14:42
0:14:42
 0:07:38
0:07:38
 0:06:48
0:06:48
 0:03:14
0:03:14
 0:02:46
0:02:46
![Derivative of ln[sinx],](https://i.ytimg.com/vi/f4jGgHflCMc/hqdefault.jpg) 0:00:22
0:00:22
 0:00:45
0:00:45
 0:02:57
0:02:57
 0:04:31
0:04:31
 0:00:35
0:00:35
 0:17:21
0:17:21
 0:03:11
0:03:11
 0:17:52
0:17:52