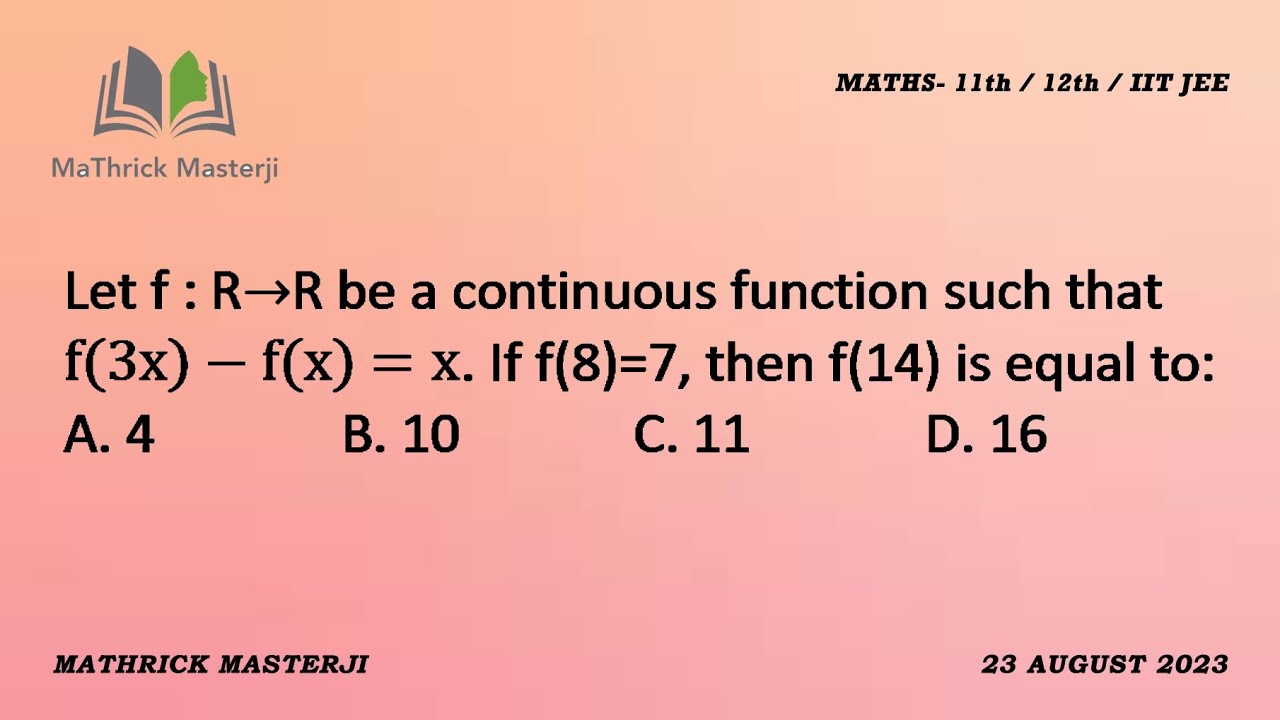
Let f:R-R be a continuous function such that f(3x)-f(x)=x. If f(8)=7, then f(14) is equal to:

Показать описание

Let f:R-R be a continuous function such that f(3x)-f(x)=x. If f(8)=7, then f(14) is equal to:
A. 4
B. 10
C. 11
D. 16

MaThrick Masterji

Рекомендации по теме

Комментарии

Simpler would be to just let g(x)=f(x)-x/2 so that g(3x)=g(x) and note that g must be constant over [1, 3) since otherwise lim_(x-->0) g(x) cannot exist. But then g must be constant, so you find f(x)=x/2+3, and hence f(14)=10.

LipschitzHutchinson

I am facing great difficulty in functional equation questions and no of functions type ques any tips ??

seema

I found out the value of f(24) which was 15. Then I thought that since f(24)=15, f(14) has to be definitely less than 15 so the options left are 10 and 11. I then saw that f(8)=7 and since there is a huge gap between 14 and 24, I guessed that the answer would be the lesser of the two i.e 10.
Would that be a correct assumption to make

Shogun

Why are we taking lim n tending to infinity?

mehulsahai

Let f:R-R be a continuous function such that f(3x)-f(x)=x. If f(8)=7, then f(14) is equal to:

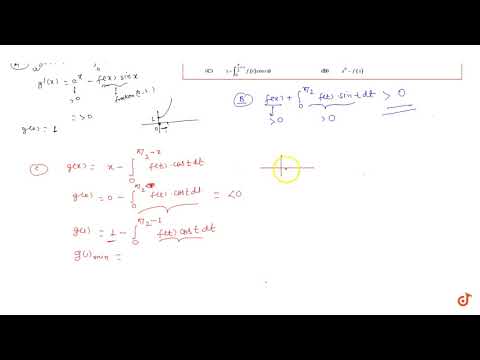
Let f : R→ (0,1) be a continuous function. Then, which of the following function(s) have the value

Let f : R?R be a continuous function such that for every x, y?R, f(x+y) =f(x) +f(y). Prove there ex…...

let `f:R- gtR` be a continuous function defined by `f(x)=1/(e^x+2e^-x)`

Are there continuous functions f:R. →R such that f(x) ∈ Q for x∈ R\Q and f(x)∈ R\Q for x∈ Q?...

Let f:R-R be a continuous function such that f(3x)-f(x)=x. If f(8)=7, then f(14) is equal to:

E. Let f: R → R be a continuous odd function, which ∑_z^* vanishes exactly at one point and f(1)=......

Let f : R &rarr; (0, 1) be a continuous function. Then, which of the following(s) function(s) ha...

Proof: f(x) = |x| is Continuous using Epsilon Delta Definition | Real Analysis Exercises

JEE MAINS 2018 Let f: R R be a continuous function defined by `f(x)''=1/(e^x+2e^(-x))`...

Let f : R rarr R be a continuous ​|JEE ADVANCED |Q 04 |YEAR 2009 | DEFINITE INTEGRATION| MATHEMATICS...

Let `f:R in R` be a continuous function such that f(x) is not identically equal to zero. If `int_(0

Let `f:R in R` be a continuous function such that f(1)=2. If `lim_(x to 1) int-(2)^(f(x)) (2t)/(x

Find the values a and b that make the piecewise function continuous

Let f: R → R be a continuous odd function, which vanishes exactly at one point and f(1)=1/2. Supp......

Let f:R---R be a continuous function which satisfies f(x)=... | jee advanced maths solution

Proof: sqrt(x) is Continuous using Epsilon Delta Definition | Real Analysis Exercises

Riemann Integration | Lecture 21 | Every Continuous Function is Riemann Integrable

Let I be a Closed Bounded Interval and f is continuous on I then it is also Uniform Continuous on I

Let `f:R to R` be continuous function such that f(x)=f(2x) for all `x in R`. If f(t)=3, then the v

Let f(x, y)=F^'(r)/r be a continuous radial function define on the annular region D=…

Let `f: Rvec(0,1)` be a continuous function. Then, which of the following function (s) has (...

If \(f: R \rightarrow R\) be a continuous function satisfying \(\int_0^{\pi / 2} f(\sin 2 x) \cd....

Let `f:R- gtR` be a continuous function such that `|f(x)-f(y)| gt=|x-y|` for all `x,y in R` , ...

Show that f(x) is continuous at x=4

Let f : R → (0, 1) be a continuous function. Then, which of the following(s) function(s) ha...

Let f : R rarr R be a continuous |JEE ADVANCED |Q 04 |YEAR 2009 | DEFINITE INTEGRATION| MATHEMATICS...