filmov
tv
Gaussian Integral 10 Fourier Way

Показать описание
Welcome to the awesome 12-part series on the Gaussian integral. In this series of videos, I calculate the Gaussian integral in 12 different ways. Which method is the best? Watch and find out!
In this video, I show how the Gaussian integral appears in the Fourier transform: Namely if you take the Fourier transform of the Fourier transform of f, you get 2pi f(-x), and I show how that 2pi relates to the Gaussian integral. At the same time, I calculate the Fourier transform of e^-ax^2 and show that this class of functions plays the analog of e^x in differential equations
In this video, I show how the Gaussian integral appears in the Fourier transform: Namely if you take the Fourier transform of the Fourier transform of f, you get 2pi f(-x), and I show how that 2pi relates to the Gaussian integral. At the same time, I calculate the Fourier transform of e^-ax^2 and show that this class of functions plays the analog of e^x in differential equations
Gaussian Integral 10 Fourier Way
Gaussian Integral 12 Ramanujan Way
The Gaussian Integral
Video#6: Gaussian Integral
An Epic Way To Do The Gaussian Integral
Gaussian Integral 8 Original Way
Fourier Transform of a Gaussian
Solving the Gaussian Integral the cool way
QM 2020 gaussian integrals
How REAL Men Integrate Functions
(MCx99) The INFAMOUS Gaussian Integral
Why π is in the normal distribution (beyond integral tricks)
Week 9 clip 7 - Fourier transform of a Gaussian
Gaussian Integral
Fourier Transformacion - Integral de Gauss
Gaussian Integral
Integration by Parts and using the Gaussian Integral!
∫(-1)^(ix²/π) dx [-∞, ∞] = ?? Is this Gaussian Integral? Can we solve it with Euler’s Formula?...
Fourier series
The Fourier transform of a Gaussian
Math and Physics: The Gaussian Integral
The Easiest Way for Calculating the Fourier Transform of a Gaussian | Signals and Systems | TDG
ADVANCED integral of e^ (-x^2) [GAUSSIAN INTEGRAL]
Gaussian Integral
Комментарии
 0:19:54
0:19:54
 0:07:19
0:07:19
 0:10:09
0:10:09
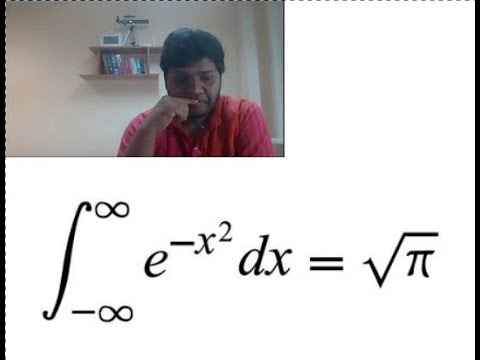 0:16:06
0:16:06
 0:03:41
0:03:41
 0:24:21
0:24:21
 0:06:42
0:06:42
 0:09:39
0:09:39
 1:20:27
1:20:27
 0:00:35
0:00:35
 0:10:28
0:10:28
 0:24:46
0:24:46
 0:04:06
0:04:06
 0:06:39
0:06:39
 0:06:33
0:06:33
 0:10:03
0:10:03
 0:05:32
0:05:32
 0:00:34
0:00:34
 0:01:00
0:01:00
 0:09:43
0:09:43
 0:18:33
0:18:33
 0:31:31
0:31:31
 0:10:57
0:10:57
 0:11:43
0:11:43