filmov
tv
[Discrete Mathematics] Indexed Sets and Well Ordering Principle

Показать описание
Today we discuss indexed sets and the well ordering principle.
*--Playlists--*
*--Recommended Textbooks--*
Hello, welcome to TheTrevTutor. I'm here to help you learn your college courses in an easy, efficient manner. If you like what you see, feel free to subscribe and follow me for updates. If you have any questions, leave them below. I try to answer as many questions as possible. If something isn't quite clear or needs more explanation, I can easily make additional videos to satisfy your need for knowledge and understanding.
*--Playlists--*
*--Recommended Textbooks--*
Hello, welcome to TheTrevTutor. I'm here to help you learn your college courses in an easy, efficient manner. If you like what you see, feel free to subscribe and follow me for updates. If you have any questions, leave them below. I try to answer as many questions as possible. If something isn't quite clear or needs more explanation, I can easily make additional videos to satisfy your need for knowledge and understanding.
[Discrete Mathematics] Indexed Sets and Well Ordering Principle
Indexed Sets
Proof and Problem Solving - Family of Sets and Indexed Family of Sets
Set Theory and Logic Lecture 6 Indexed Sets
Lesson 4: Intro to Indexed sets and Example 1
Set Theory | All-in-One Video
Indexed sets
LEC 1 INDEX SET, INDEXED FAMILY OF SETS, UNION AND INTERSECTION, EXAMPLES #TGT_JSSC_MATH #settheory
Discrete Mathematics Indexed Sets and Well Ordering Principle
Set Theory - Indexed Sets: Intersection and Union Proof
Generalized Unions and Intersections
Performing an indexed union and intersection
Index Set, Indexed Sets, Indexed Family of Sets || SIOM || MAHENDRA SAGAR ||
[Discrete Mathematics] Set Operations
Countable and Uncountable Sets - Discrete Mathematics
Set Theory #mathematics
Sets Notations#sets symbols #Maths#shorts
What is a Power Set? | Set Theory, Subsets, Cardinality
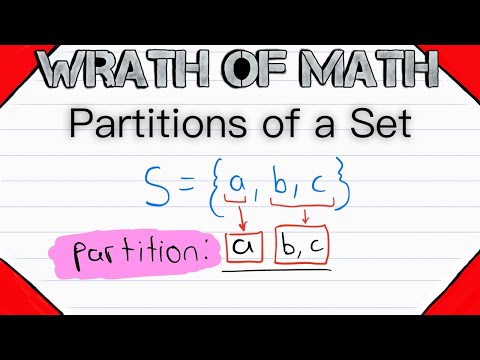
Partitions of a Set | Set Theory
IIT Bombay CSE 😍 #shorts #iit #iitbombay
Geometric Mean Class 10 #shorts
Lecture 6 (Discrete Mathematics I)-part 1
L-1.1: Introduction to Set Theory | Set, Subset, Proper Subset
Union And Intersection Of More Than Two Sets
Комментарии
![[Discrete Mathematics] Indexed](https://i.ytimg.com/vi/TDpQSa3hJRw/hqdefault.jpg) 0:07:38
0:07:38
 0:09:38
0:09:38
 0:05:16
0:05:16
 0:32:19
0:32:19
 0:12:34
0:12:34
 0:29:24
0:29:24
 0:11:12
0:11:12
 0:17:06
0:17:06
 0:07:38
0:07:38
 0:05:35
0:05:35
 0:03:35
0:03:35
 0:04:52
0:04:52
 0:33:08
0:33:08
![[Discrete Mathematics] Set](https://i.ytimg.com/vi/jRuKua-afK0/hqdefault.jpg) 0:13:35
0:13:35
 0:10:02
0:10:02
 0:00:52
0:00:52
 0:00:41
0:00:41
 0:04:05
0:04:05
 0:07:59
0:07:59
 0:00:11
0:00:11
 0:00:47
0:00:47
 0:44:43
0:44:43
 0:09:23
0:09:23
 0:13:05
0:13:05