filmov
tv
Proof Construction: A function with bounded derivative is uniformly continuous

Показать описание
This pen-cast illustrates the construction of a proof, from the statement of the proposition, through the analysis of the terminology, to the proof itself. Along the way, we give one example that shows the proposition is worth proving: the arctangent function is easily shown to be uniformly continuous. In fact, the proof of the general statement could be applied to this example by the viewer, to further solidify their understanding of the proof.
The proof is based on the use of the Mean Value Theorem for Derivatives, which is reviewed.
[caveat: there is one "error" on the last page. One of the ≤ could be = (which one?). Nonetheless, the proof is still correct with the ≤ as it stands]
Prerequisites: If you have a good grasp of Calculus I, you should be able to understand the elements of this proof. This video was made for those struggling to learn how to construct proofs. Some people without such troubles may find there are too many details or that the proof is made overly complicated. My purpose was not to make the simplest proof, but to make a clear proof and to explain as much as possible what ingredients are needed to construct the proof, in order to help math learners who would like to understand higher mathematics yet don't understand how to construct proofs or how to reason.
The proof is based on the use of the Mean Value Theorem for Derivatives, which is reviewed.
[caveat: there is one "error" on the last page. One of the ≤ could be = (which one?). Nonetheless, the proof is still correct with the ≤ as it stands]
Prerequisites: If you have a good grasp of Calculus I, you should be able to understand the elements of this proof. This video was made for those struggling to learn how to construct proofs. Some people without such troubles may find there are too many details or that the proof is made overly complicated. My purpose was not to make the simplest proof, but to make a clear proof and to explain as much as possible what ingredients are needed to construct the proof, in order to help math learners who would like to understand higher mathematics yet don't understand how to construct proofs or how to reason.
Комментарии
 0:24:25
0:24:25
 0:07:33
0:07:33
 0:08:06
0:08:06
 0:10:55
0:10:55
 0:03:51
0:03:51
 0:01:48
0:01:48
 0:13:19
0:13:19
 0:06:25
0:06:25
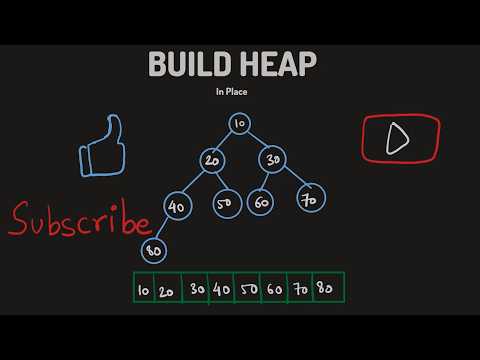 0:13:18
0:13:18
 0:07:16
0:07:16
 0:17:05
0:17:05
 0:06:04
0:06:04
 0:15:11
0:15:11
 0:01:01
0:01:01
 0:02:18
0:02:18
 0:27:07
0:27:07
 0:05:07
0:05:07
 0:07:25
0:07:25
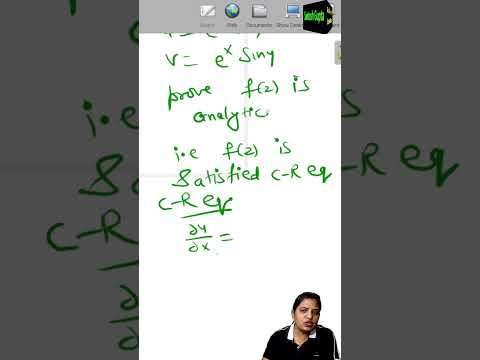 0:01:00
0:01:00
 0:04:13
0:04:13
 0:12:55
0:12:55
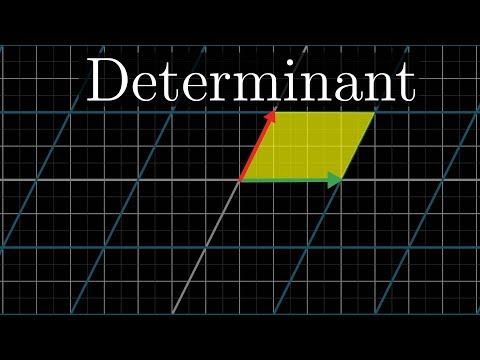 0:10:03
0:10:03
 0:11:51
0:11:51
 0:01:18
0:01:18