filmov
tv
Solving Radical Equations

Показать описание
Solve equations involving radicals (roots) or rational exponents by undoing (using inverse operations) and getting the x by itself. Extraneous solutions are explained and a number of examples are shown.
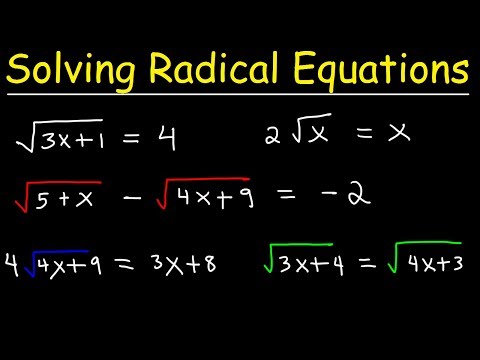
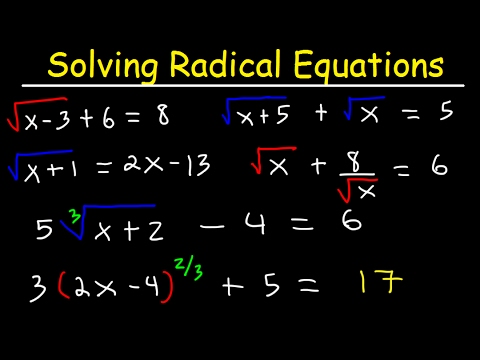
Solving Radical Equations
Solving Radical Equations With Square Roots, Cube Roots, Two Radicals, Fractions, Rational Exponents
Solving radical equations | Exponent expressions and equations | Algebra I | Khan Academy
Solving Radical Equations
#9. Solve the Radical Equation with Two Square Roots
Solving a Radical Equation Using Factoring and Checking Your Answers
05 - Solving Radical Equations & Identifying Extraneous Solutions - Part 1
Math Tutorial for Solving Radical Equations
J.E.D.I. Training MATH for DIGITAL SAT - Day 4 of 7 (ADVANCED MATH Critical Concepts)
Solving Radical Equations | SHS 1 ELECTIVE MATH
Solve Radical (Square Root) Equations with Two Radicals
Solving Radical Equations (More Challenging)
How To Solve Radical Equations
Solving Radical Equations - Solution of Radical Equations
Radical equation – each step to solve…
The most important thing you need to know to solve radical equations
How To Solve Complex Radical Equations
Learn How to Solve a Radical Equation and Check Your Answers
How to Solve Radical Equation with Two Radicals?
SOLVING RADICAL EQUATIONS || GRADE 9 MATHEMATICS Q2
How to Solve Radical Equations that have Two Radicals - Simple Method
How to solve a radical equation by using rational powers using absolute value
Solving an Equation with the Difference of Two Radical Expressions
Radical Equation with a Extraneous Solution
Комментарии
 0:17:11
0:17:11
 0:18:05
0:18:05
 0:03:11
0:03:11
 0:03:31
0:03:31
 0:05:17
0:05:17
 0:04:25
0:04:25
 0:27:27
0:27:27
 0:03:45
0:03:45
 1:20:40
1:20:40
 0:11:48
0:11:48
 0:09:31
0:09:31
 0:04:14
0:04:14
 0:10:40
0:10:40
 0:10:01
0:10:01
 0:14:34
0:14:34
 0:03:42
0:03:42
 0:10:07
0:10:07
 0:03:18
0:03:18
 0:05:45
0:05:45
 0:12:53
0:12:53
 0:08:23
0:08:23
 0:07:38
0:07:38
 0:06:00
0:06:00
 0:05:11
0:05:11