Line Integrals of Scalar Functions: Evaluate Line Integrals : Contour Integrals

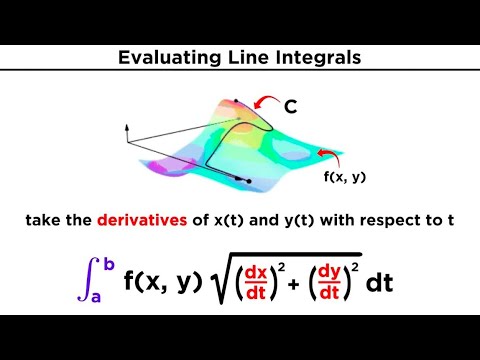
Here we discuss how to evaluate line integrals of scalar functions or scalar fields. Often this is called contour integration where the curve is the contour.

A scalar function is a function that outputs a real number rather than a vector.

When evaluating the line integral of a scalar function, we must remember that we will multiply the integrand f(r(t)) by |r'(t)|.

Once we have everything in terms of t we integrate the single variable integral.

Often we must parameterize the curve ourselves as is the case in part a)
we have
a) ∫xy^4 ds where C is the right half of the circle of radius 4 centered at the origin.

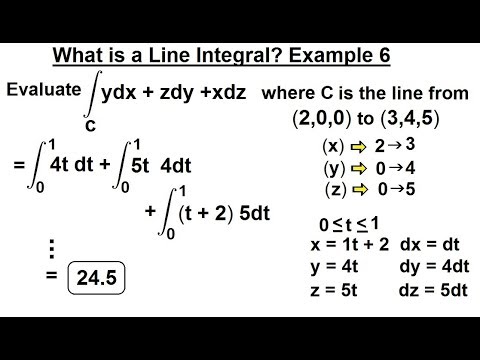
b) ∫2x+9y ds where C is given by x=t, y=t^2, z=t^3, for t between 0 and 1..

Thanks for watching! A

As always if you have questions let me know in the comments and I will reply! Thanks!