Prove that the limit of √(n+√n) - √n = 1/2 (ILIEKMATHPHYSICS)

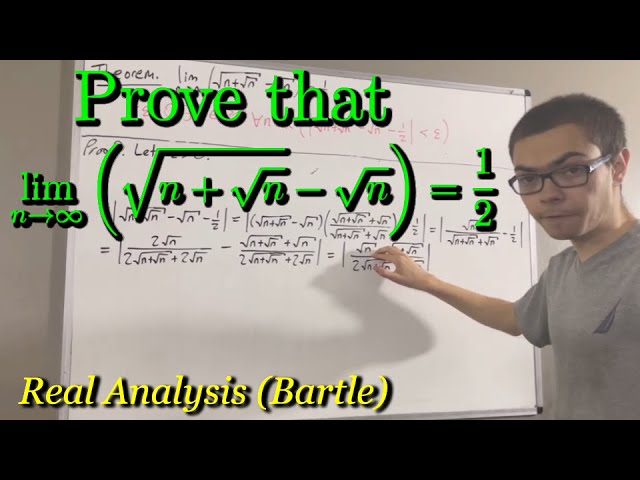
preview_player
Показать описание
This video references the book "Introduction to Real Analysis" by Bartle and Sherbert (Fourth Edition). For more details regarding the techniques used in this video, see Section 2.4 and Section 3.1.

Thanks and enjoy the video!

  1. ILIEKMATHPHYSICS
Рекомендации по теме
Proof of a 0:09:17

Proof of a Limit Value Using Epsilon and Delta

Definition of the 0:13:59

Definition of the Limit of a Sequence | Real Analysis

proving the limit 0:28:27

proving the limit of a product is the product of the limits, epsilon-delta definition

Proof: The Limit 0:13:22

Proof: The Limit of a Sequence is Unique | Real Analysis

This is a 0:00:48

This is a very famous limit

1.7 Proving a 0:14:04

1.7 Proving a Limit: x^2 = 4 (advanced)

The most important 0:11:54

The most important limit in Calculus // Geometric Proof & Applications

Limit Laws - 0:08:18

Limit Laws - Proof of Sum Law

How To Find 0:13:14

How To Find The Limit At Infinity

Epsilon delta limit 0:05:36

Epsilon delta limit (Example 4): Limits at infinity

The Most Important 0:03:05

The Most Important Limit In Calculus

Proof: Central Limit 0:35:14

Proof: Central Limit Proof

How to prove 0:06:04

How to prove that the limit does not exist (KristaKingMath)

The Limit of 0:05:14

The Limit of a Sequence is Unique Proof

The Limit (do 0:12:08

The Limit (do not use L'Hospital rule)

How to Prove 0:11:39

How to Prove that the Limit of (2n + 1)/(3n + 7) as n approaches infinity is 2/3

Prove the limit 0:12:35

Prove the limit does not exist. Real Analysis Example (2.1)

Proving All the 0:54:07

Proving All the Sequence Limit Laws | Real Analysis

Simple Limit Proof 0:07:39

Simple Limit Proof Using Epsilon-Delta Definition of a Limit

2.9 How to 0:09:24

2.9 How to prove a limit DNE from the definition

Proof: Limit of 0:10:21

Proof: Limit of sinx/x as x approaches 0 with Squeeze Theorem | Calculus 1

Epsilon Delta Proof 0:06:17

Epsilon Delta Proof of a Limit 1

Limit of a 0:07:55

Limit of a Composite Function Theorem: Proof

2.11 Proof of 0:08:21

2.11 Proof of the limit law for sums

Комментарии
Автор

Factor sqrt(n) from the expression, to get sqrt(n) ( sqrt(1 + 1/sqrt(n) ) - 1).

Let h = 1/sqrt(n).

lim n -> infinity sqrt(n) (sqrt(1-1/sqrt(n)) -1) = lim h -> 0+ (sqrt(1 + h) -1) / h.

This is, up to the one-sided limit, the definition of the derivative of sqrt(x) evaluated at x = 1.

The two-sided limit exists and it is 1/2.

This method pushes the formal limit argument onto the proving the derivative.

PrincetonAthleticClub
Автор

fantastic video! keep up the great work :)

DemigodTet
Автор

Change to a fraction with (1/n) denominator, then apply L'H -simples!

johnpaterson