Differentiable convex functions II

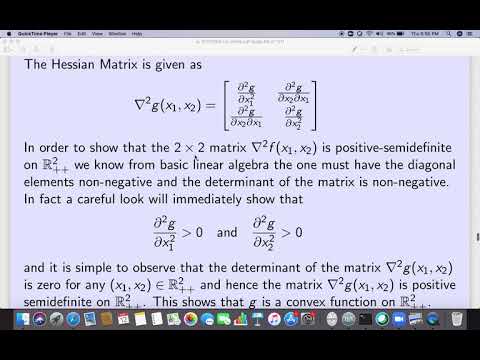
We finish the proof of the theorem by showing that a monotone gradient implies convexity and prove that convexity of a twice differentiable function is equivalent to the Hessian being positive semidefinite at each point.

[sbanert]

Correction: It must be $d \in \mathcal{H}$ instead of $d \in \mathbb{R}^n$ at the beginning of the second proof.

[fariasmaia]

Nice! I am loving that playlist. Thanks a lot!