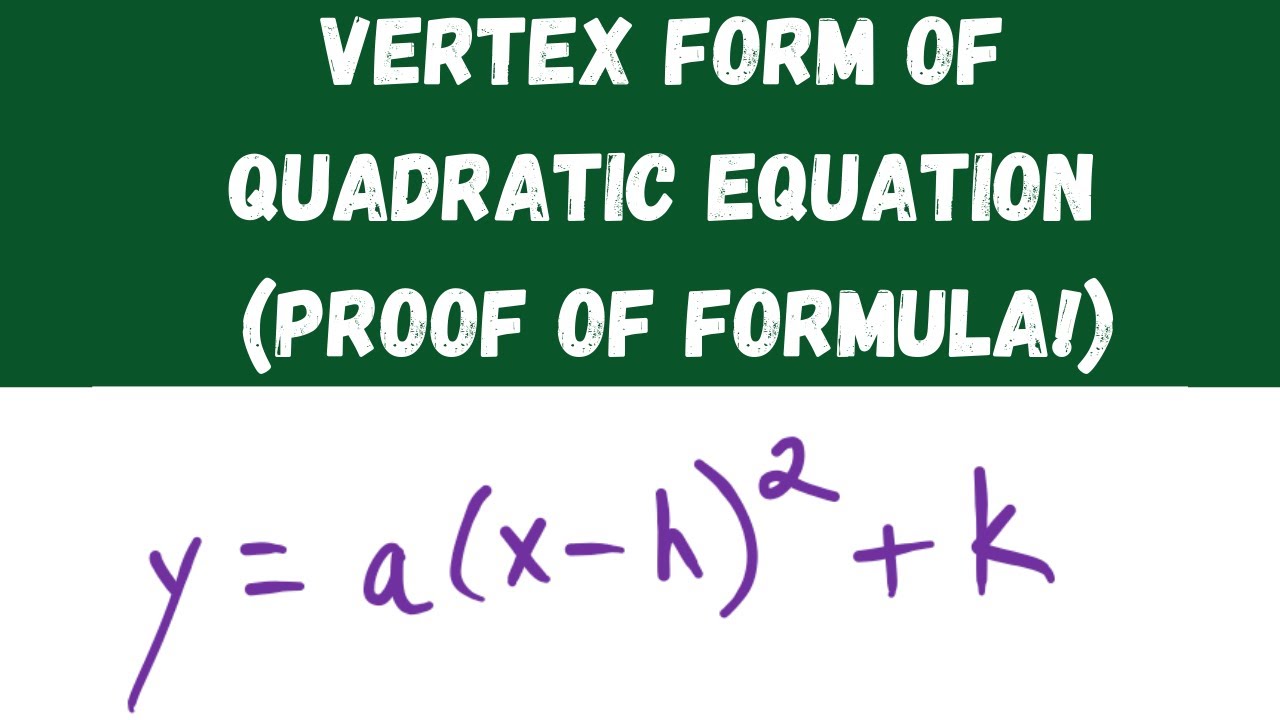
vertex form of a quadratic equation (proof of formula!)

preview_player
Показать описание
In this video I prove the formula for the vertex form of a quadratic equation. I show how the standard form of a quadratic equation, along with the formula for the vertex of a quadratic equation can be used to derive the vertex form of a quadratic equation formula.

If this video was helpful, please LIKE and SUBSCRIBE. Leave any video requests in the COMMENT section below. Thanks for watching!
  1. vinteachesmath
  2. vertex form
  3. vertex form of a quadratic equation
  4. proof of vertex form quadratic equation
  5. vertex form quadratic equation
  6. how to put a quadratic equation in vertex form
Рекомендации по теме
Standard Form to 0:02:43

Standard Form to Vertex Form - Quadratic Equations

Introduction to vertex 0:06:13

Introduction to vertex form of a quadratic

Standard to Vertex 0:05:46

Standard to Vertex form - Quadratic Equations

How do you 0:08:08

How do you convert from standard form to vertex form of a quadratic

The Vertex Form 0:03:36

The Vertex Form of Quadratic Functions - Nerdstudy

Graphing Quadratic Functions 0:07:58

Graphing Quadratic Functions In Vertex Form

Graphing a parabola 0:03:16

Graphing a parabola in vertex form | Quadratic equations | Algebra I | Khan Academy

Completing the square 0:06:14

Completing the square for vertex form | Quadratic equations | Algebra I | Khan Academy

vertex form of 0:04:45

vertex form of a quadratic equation (proof of formula!)

Rewriting Quadratic Functions 0:10:54

Rewriting Quadratic Functions To Vertex Form y = a(x - h)² + k: 9th Grade Math

Graphing Parabolas in 0:02:44

Graphing Parabolas in Vertex Form

Vertex Form of 0:09:31

Vertex Form of a Quadratic Function (Lesson 2-1)

Convert from Standard 0:07:16

Convert from Standard Form to Vertex Form of a Quadratic (Parabola) 2 Methods

Converting a Quadratic 0:07:27

Converting a Quadratic Function From Standard Form to Vertex Form (Completing the Square)

Parabola Vertex Form 0:03:34

Parabola Vertex Form for Quadratic Functions (by Completing the Square)

Standard to Vertex 0:13:40

Standard to Vertex Form when a is NOT 1 - Quadratic Equations

12 - Writing 0:35:51

12 - Writing Quadratic Functions in Vertex Form - Part 1 (Graphing Parabolas)

Graphing Quadratic Functions 0:09:01

Graphing Quadratic Functions in Standard Form (Vertex Form)

Vertex Form of 0:03:50

Vertex Form of a Quadratic Equation

Completing the square 0:04:14

Completing the square to transform from standard to vertex form

Quadratic Transformations Vertex 0:05:12

Quadratic Transformations Vertex Form Tutorial

Writing an equation 0:05:52

Writing an equation in vertex form from quadratic form

How to Convert 0:04:35

How to Convert a Quadratic Equation from Standard Form to Vertex Form

Learn how to 0:03:04

Learn how to find the solutions x intercepts and vertex of a quadratic in vertex form ex 7

Комментарии
Автор

This might be the greatest math video I have ever seen

realsm
Автор

Excellent video! I remember seeing this formula in school, but I never quite understood where it came from. You explained it beautifully.

The only thing some people might get a bit confused with is why h = -b/2a. The way I understand that one is to take the first derivative of a generic quadratic equation ax²+bx+c, which becomes 2ax+b. The slope of the quadratic is 0 at its vertex point, so 0 = 2ax+b, which you can solve for x to get x = -b/2a. Hopefully this comment might help someone to understand this a bit better, although it does require an understanding of derivatives which normally comes after studying this part of functions.

FootballPsychoPST
Автор

This is beautiful. Is it just luck that we get a perfect square trinomial at the end? I want to see how they found this in the first place. Gorgeous equation

Nick_Reinhardt