Repeated Eigenvalues and Secular Terms: Transient Growth in Non-Normal Systems

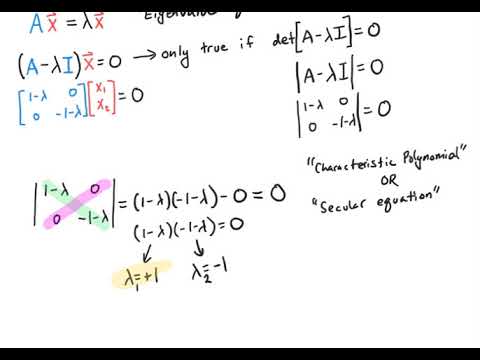
This video investigates how to analytically solve a linear system of differential equations with repeated eigenvalues. Specifically we derive the "secular terms" te^{lambda*t} for non-normal systems.

@eigensteve on Twitter

This video was produced at the University of Washington

%%% CHAPTERS %%%

[VasilevArtem-gu]

This part of the playlist is so lifesaving. I wasn't able to get the grip of this part when things become messy with eigenvalues but now it became clear. Thank you, Steve

[tomosmallpond]

Hello Dr. Brunton. This is irrelevant from the content of the video but is there anyway you could make a video about Galerkin method for PDEs?

[hellfirebb]

Hi professor, since we are hitting Jordan block matrix now, will you cover Jordan decomposition and generalized eigenvectors in upcoming lectures?

[johnfinn]

The matrix A={{lambda, 1}, {0, lambda}} is nonnormal but also a Jordan block. Nonnormal matrices can have transient growth but only with degeneracy do you get secular terms \propto t exp(lambda t). The nondegenerate matrix {{lambda, 1}, {0, mu}} has transient growth (a combination of exp(lambda t) and exp(mu t)) even for lambda \neq mu.

[superuser]

Can you estimate/model the transient growth/stable decay with a beta distribution?

[michaelward]

I asked Chat GPT to prove that proposition for me

Prove that for matrices S and T, if ST = TS then e^(S+T) = (e^S)e^T

ChatGPT's response

"""
This statement can be proven using the matrix exponential definition and the properties of matrix multiplication. The matrix exponential of a matrix A is defined as e^A = I + A + (A^2)/2! + (A^3)/3! + ... where I is the identity matrix. Using this definition, we can expand e^(S+T) as:

e^(S+T) = I + (S+T) + (S+T)^2/2! + (S+T)^3/3! + ...

Using the assumption that ST = TS, we can expand the term (S+T)^2 as:

(S+T)^2 = S^2 + ST + TS + T^2 = S^2 + T^2 + 2ST

Similarly, we can expand the term (S+T)^3 as:

(S+T)^3 = S^3 + STS + TST + T^3 + 3S^2T + 3ST^2 = S^3 + T^3 + 3ST(S+T)

By substituting these expanded terms back into the definition of e^(S+T), we can see that the terms involving ST and TS cancel out, leaving us with:

e^(S+T) = I + S + T + S^2/2! + T^2/2! + S^3/3! + T^3/3! + ...

This is equivalent to:

e^(S+T) = (I + S + S^2/2! + S^3/3! + ...)(I + T + T^2/2! + T^3/3! + ...) = e^S e^T

Therefore, we have shown that e^(S+T) = (e^S)e^T if ST = TS.
"""

[byronwatkins]

In this case we eventually have exponential growth instead of exponential decay.

[younique]

Why the matrix e^St is made by putting e (at 7:30) while the matrix e^Tt is made by Power Series Expansion (at 7:50)? Why the matrix e^Tt is not [0t;00] instead of [1t;01]?